Two vertices of a triangle are (3,−1) and (−2,3) and its orthocenter is at the origin,. Find the coordinates of its third vertex.
Let the vertices of △ABC are A(h,k),5(3,−1) and(−2,3).
Side AB passes through point (3,−1) and is perpendicular to DC whose slope is−32
Equation of AB
⇒y+1=23(x−3)
⇒3y+3=2x−6
⇒2x−3y−9=0 ....(i)
Similarly AC passes through (−2,3) and is perpendicular to OB, whose slope is −13
Thus equation of side AC
y−3=3(x+2)
⇒3x−y+9=0.....(ii)
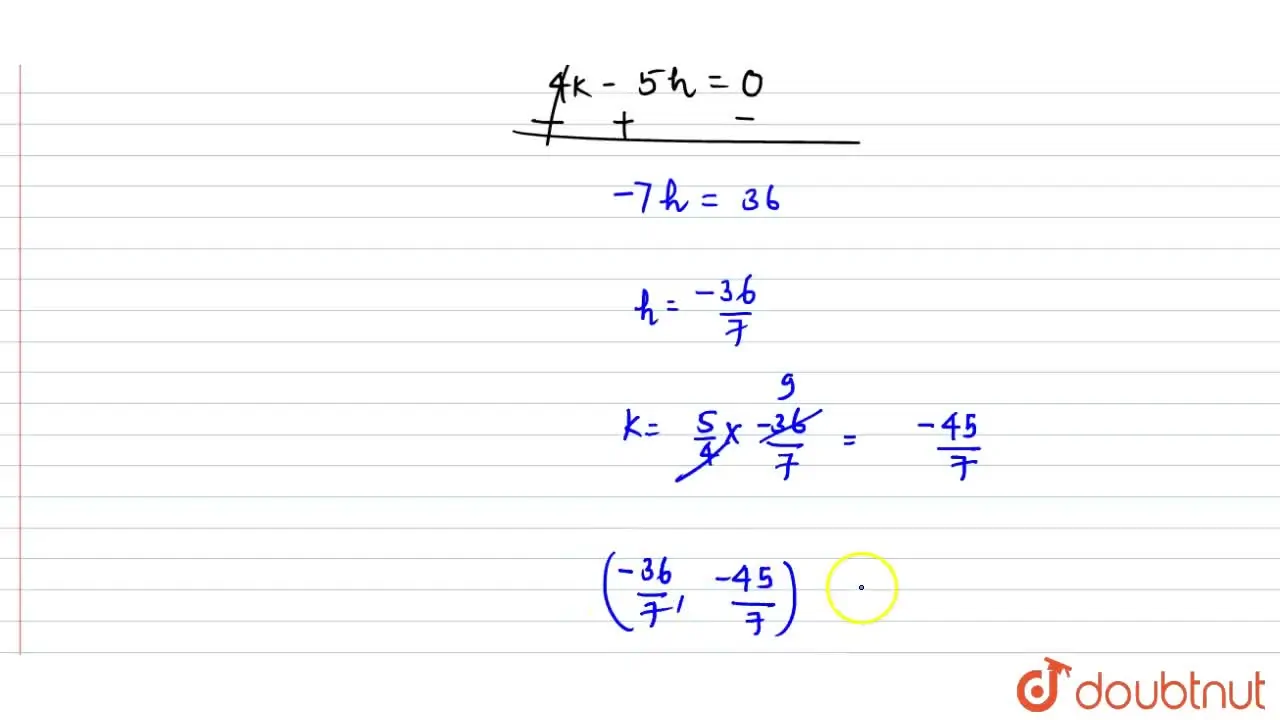
Solving equation (i) and (ii)
x=−367 and y=−457
Thus, coordinates of A is (−367,−457)
Related Playlists
Similar Questions
Two vertices of a triangle are (3,−1) and (−2,3) and its orthocenter is at the origin,. Find the coordinates of its third vertex.
View SolutionIf two vertices of a triangle are (5, -1) and (-2, 3) and if its orthocentre lies at the origin, then the coordinates of third vertex are
View SolutionTwo vertices of a triangle are (1, 2), (3, 5) and its centroid is at the origin. Find the coordinates of the third vertex.
View SolutionTwo vertices of a triangle are (5,−1) and (−2,3) If the orthocentre of the triangle is the origin, find the coordinates of the third point.
View SolutionTwo vertices of a triangle ABC are B(5,-1) and C(-2,3) .If the orthocentre of the triangle is the origin , find the thrid vertex.
View SolutionTwo vertices of a triangle are at (- 3, 1) and (0,2) and the centroid is at the origin. Find its third vertex.
View SolutionTwo vertices of a △ABC are B (- 3,1) and C (0,-2) and its centroid is at the origin. The third vertex A is:
View SolutionTwo vertices of a triangle are (4,−3)&(−2,5). If the orthocentre of the triangle is at (1,2), find coordinates of the third vertex .
View SolutionTwo vertices of a triangle are (5,−1) and (−2,3). If the orthocentre of the triangle is the origin, then the third vertex is
View SolutionTwo vertices of a triangle ABC are B(5,-1) and C(-2,3) .If the orthocentre of the triangle is the origin , find the thrid vertex.
View Solution