The velocity time graph for the veticaly component of the velocity of a body thrown upwards from the ground and landing on the roof of a building is given in the figure.The height of the building is:
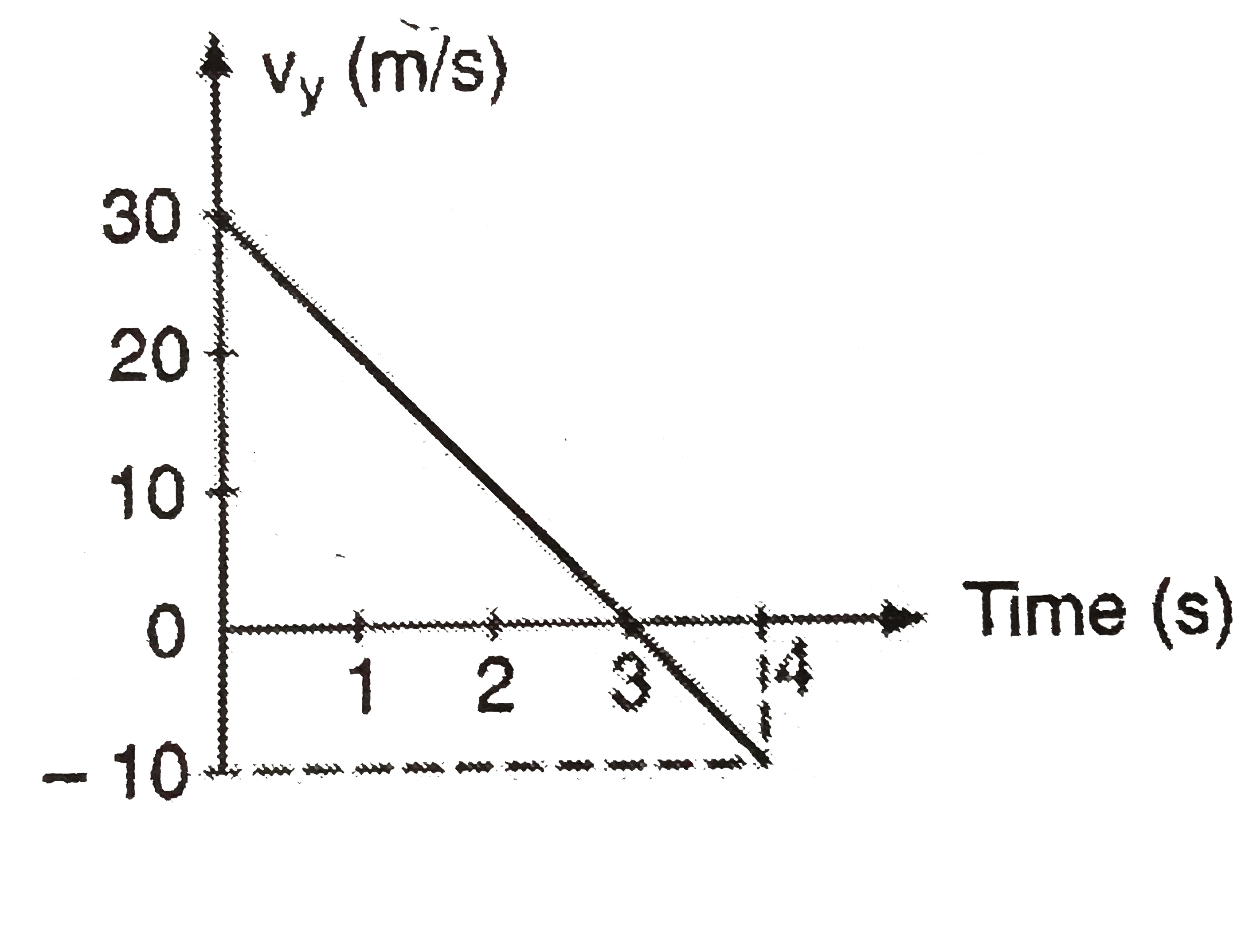
50 m
40 m
20 m
30 m
The correct Answer is:B
Height = algebraic sum of area under u−t graph
=12×30×3−12×10×1
=40m
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBOOK - GRB PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - More than one choice is correct
16 Videos
- MOTION IN A STRAIGHT LINEBOOK - GRB PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Assertion Reason
12 Videos
- MOTION IN A STRAIGHT LINEBOOK - GRB PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Problems and Practice
16 Videos
- FRICTION AND CIRCULAR MOTION BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - Comprehension type
11 Videos
- MOTION IN TWO AND THREE DIMENSIONSBOOK - GRB PUBLICATIONCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - All Questions
210 Videos
Similar Questions
Knowledge Check
The velocity time graph for the veticaly component of the velocity of a body thrown upwards from the ground and landing on the roof of a building is given in the figure.The height of the building is:
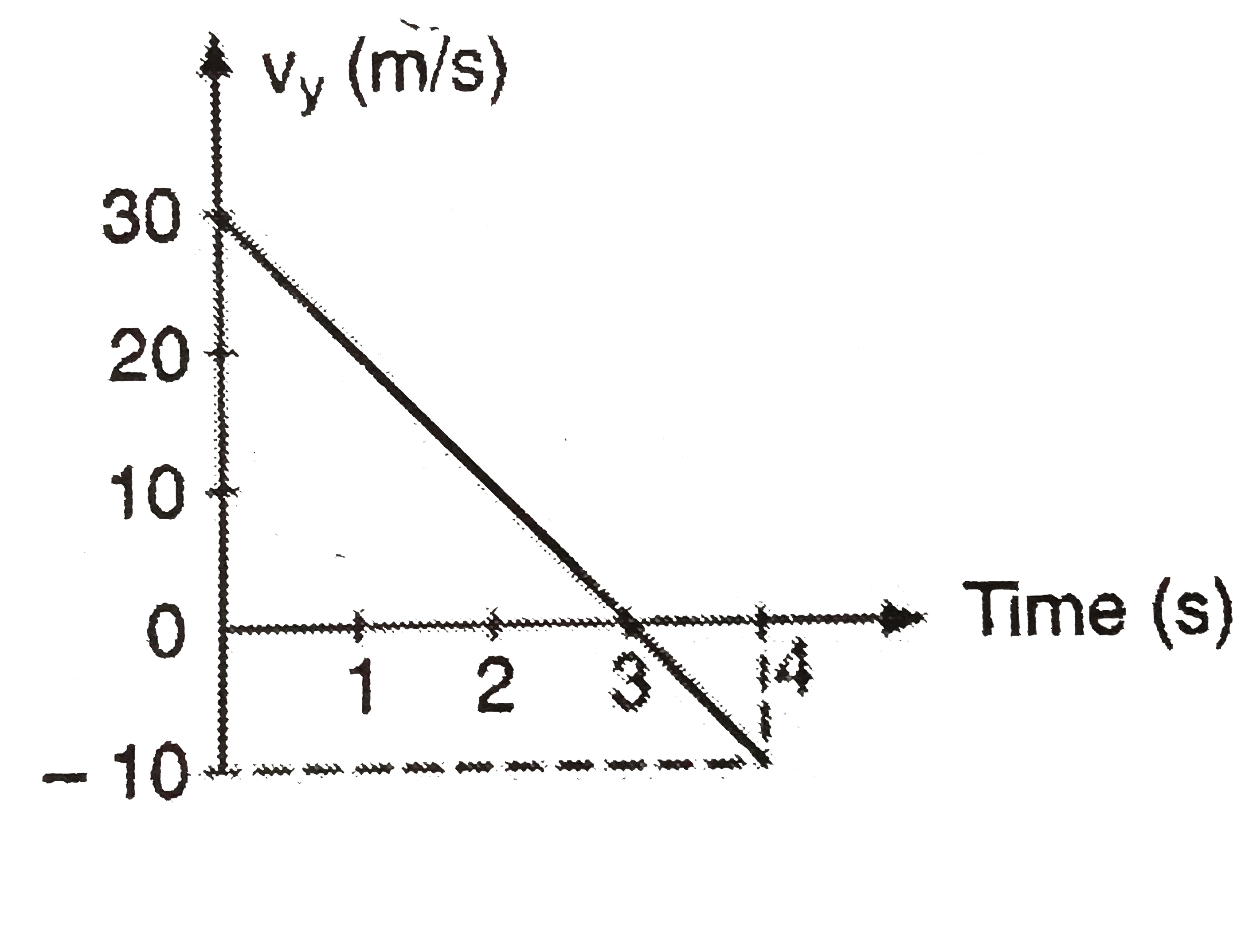 A50 mB40 mC20 mD30 m
A50 mB40 mC20 mD30 mVelocity-time graph of a body thrown vertically up is
Aa straight lineBa parabolaCa hyperbolaDcircleA stone dropped from a building takes 4 s to reach the ground. The height of the building is
A19.6mB80.4mC78.4mD156.8m
The given bar graph shows the heights of different buildings.
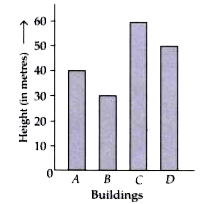
What is the height of building C ?
View SolutionA stone is thrown downwards with velocity 10 m/s from the roof of a builng and it reaches the ground with speed 40 m/s. the height of the building would be
View SolutionThe angle of elevation of the top of a building and the top of the chimney on the roof of the building from a point on the ground are x and 45∘ respectively .The height of building is h metre .Then the height of the chimney (in metre ) is :
View SolutionA stone thrown upward with a speed u from the top of a tower reaches the ground with a velocity 4u. The height of the tower is
View SolutionIf a ball is thrown up with a velocity v from the top of a building the ball reaches the ground with a velocity 3u. Find the height of the building.
View Solution
