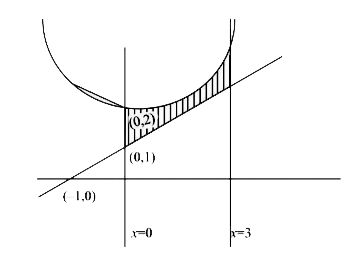
The area (in sq. units) of the region bounded by the parabola $y = x^{2} + 2 and the lines y = x + 1, x = 0 and x = 3$ , is

$\frac{15}{4}$

$\frac{15}{2}$

$\frac{21}{2}$

$\frac{17}{4}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

The area of the region bounded by the parabola $y = x^{2} + 2$ and the lines y = x, x = 0 and x = 3 is
A $\frac{7}{2} s q . u n i t s$
B $\frac{17}{2} s q . u n i t s$
C $\frac{21}{2} s q . u n i t s$
D $\frac{15}{2} s q . u n i t s$
The area (in sq units) of the region bounded by the curve $2 x = y^{2} - 1 and x = 0$ is
A $1 / 3$
B $2 / 3$
C1
D2
The area of the region bounded by the parabola $y = x^{2}$ and the line y = 4x is
A $\frac{32}{3} s q . u n i t s$
B $\frac{16}{3} s q . u n i t s$
C $\frac{8}{3} s q . u n i t s$
D $\frac{4}{3} s q . u n i t s$