If A = { (x,y) : y = sin x, x ∈ R } and B = { (x,y) : y = cos x, x ∈ R} then A ∩ B contains Solution in Tamil
no element
infinitely many elements
only one element
cannot be determined
The correct Answer is:infinitely many elements
Step by step video, text & image solution for If A = { (x,y) : y = sin x, x in R } and B = { (x,y) : y = cos x, x in R} then A cap B contains by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SURAS MODAL QUESTION PAPER-1 MATHEMATICS BOOK - SURA PUBLICATIONCHAPTER - SURAS MODAL QUESTION PAPER-1 MATHEMATICS EXERCISE - Section-II
11 Videos
- SURAS MODAL QUESTION PAPER-1 MATHEMATICS BOOK - SURA PUBLICATIONCHAPTER - SURAS MODAL QUESTION PAPER-1 MATHEMATICS EXERCISE - Section-III
10 Videos
- SETS RELATIONS AND FUNCTIONSBOOK - SURA PUBLICATIONCHAPTER - SETS RELATIONS AND FUNCTIONSEXERCISE - ADDITIONAL PROBLEMS (SECTION - D) (MARKS 5)
11 Videos
- SURAs MODEL QUESTION PAPER - 1BOOK - SURA PUBLICATIONCHAPTER - SURAs MODEL QUESTION PAPER - 1EXERCISE - PART-IV
11 Videos
Similar Questions
Show that the relation R in the set {1,2,3} given by R={(1,1),(2,2),(3,3),(1,2),(2,3)} is reflexive but neither symmetric nor transitive.
View SolutionA plumber can be paid according to the following schemes, In the first scheme he will be paid rupees 500 plus rupees 70 per hour, and in the second scheme he will paid 120 rupees per hour. If he works x hours. Then for what value of x does the first scheme give better wages?
View SolutionA relation R is defined on the set z of integers as follows : (x,y) ∈R⇔x2+y2=25. Express R and R−1 as the set of ordered pairs and hence find their respective domains.
View SolutionExplain the meaning of heat and work with suitable examples.
View SolutionIn some question of sets, we have to make the use of graphs
For example
A={(x,y):y=ex,x∈R}
B={{x,y}:y=−x.x∈R}
Find n(A∩B)
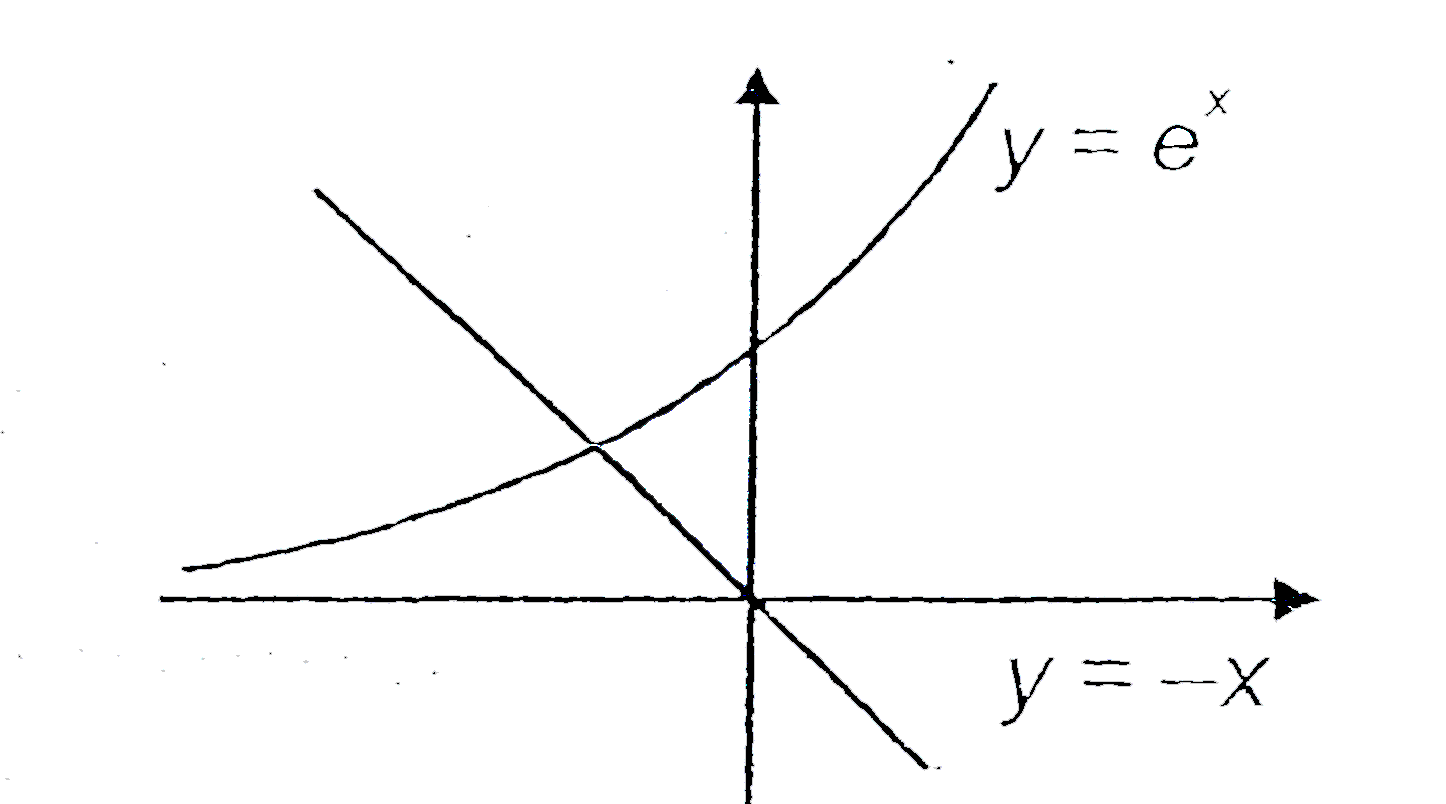
It is clear that y=exandy=−x intersect at one pont. Hence n(A∩B)=1
A:{(x,y):y=√4−x2,x∈[−2,2]}
B={(x,y):y=|x|,x∈R}
Then n(A∩B)
View SolutionIn some question of sets, we have to make the use of graphs
For example
A={(x,y):y=ex,x∈R}
B={{x,y}:y=−x.x∈R}
Find n(A∩B)
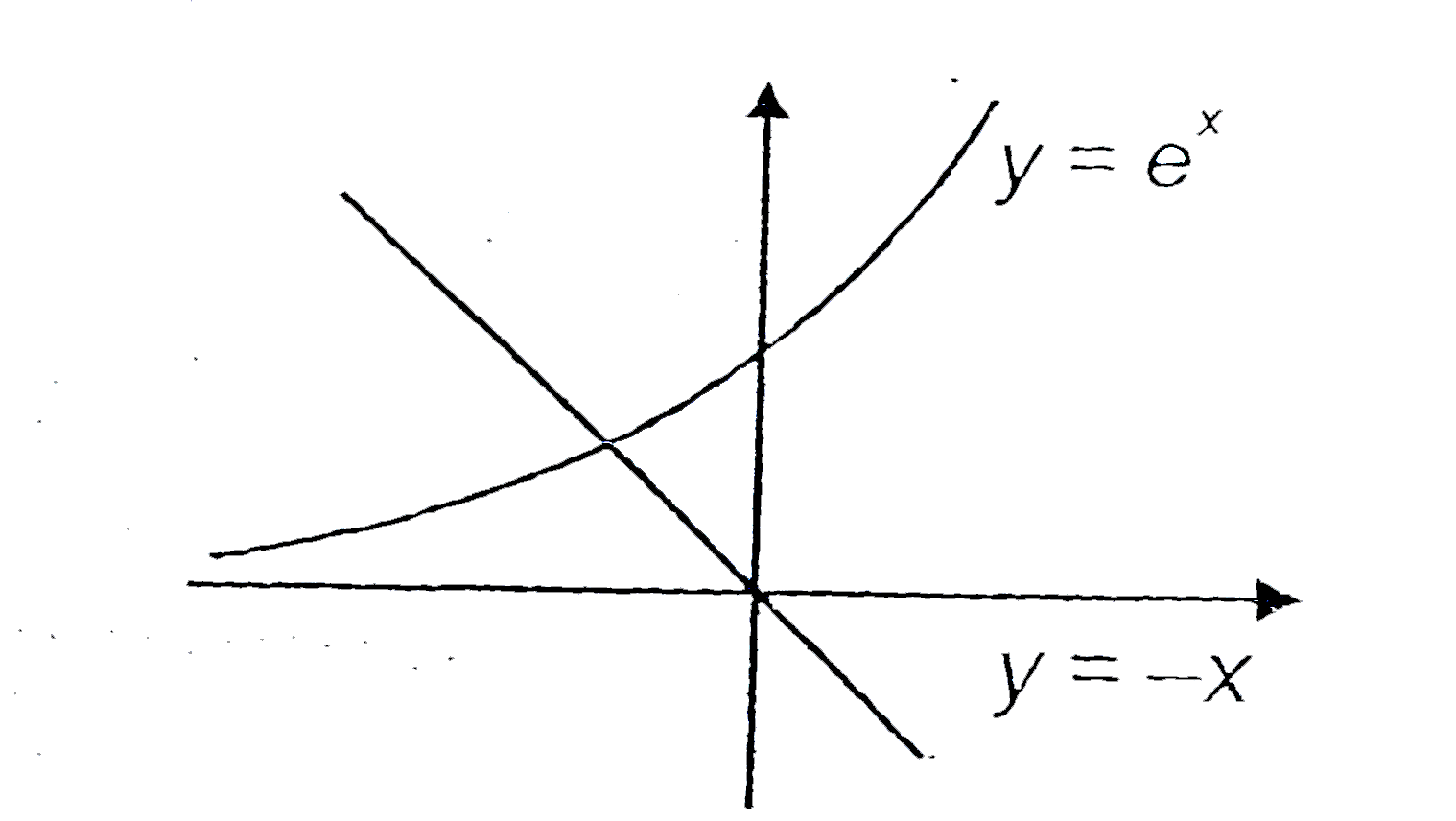
It is clear that y=exandy=−x intersect at one pont. Hence n(A∩B)=1
A={(x,y):x2+y2≤2,x,y∈R}
B={{x,y):y≥x2,x,y∈R}
Then domain of A∩B is
View SolutionIn some question of sets, we have to make the use of graphs
For example
A={(x,y):y=ex,x∈R}
B={{x,y}:y=−x.x∈R}
Find n(A∩B)
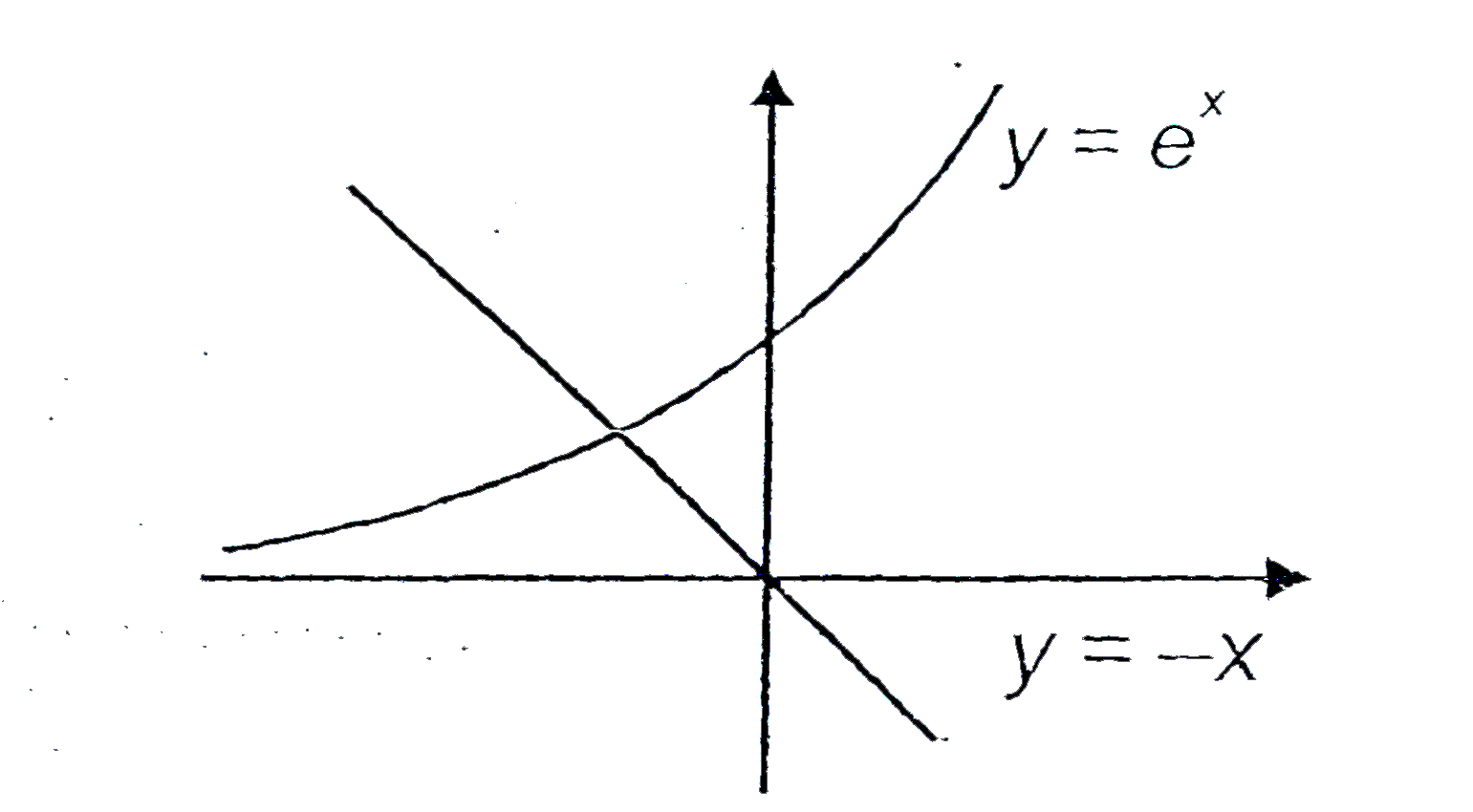
It is clear that y=exandy=−x intersect at one pont. Hence n(A∩B)=1
A={(x,y):y=sinπx,x∈R}
B={(x,y):y=|In|x||,x∈R−{0}}
Then, n(A∩B)
View SolutionLet A={(x,y):y=ex,x∈R}B={(x,y),Y=e−x,x∈R}then
View SolutionIf A= {(x,y) : y = ex,x∈R} and B = { (x,y) : y = e−x,x∈R } then n (A ∩ B ) is
View SolutionIf A = { (x,y) : y = sin x, x ∈ R } and B = { (x,y) : y = cos x, x ∈ R} then A ∩ B contains
View SolutionA={(x,y):y=1/x,0≠x∈R},B={(x,y):y=−x,x∈R} then
View SolutionA={(x,y):y=ex,x∈R},B={(x,y):y=x,x∈R} then
View SolutionLet A={(x,y): y=ex,x∈R } , B={(x,y): y= e−x,x∈R } . Then :
View SolutionLet A={(x,y : y=ex,x∈R} , B={(x,y): y=x, x ∈ R} . Then :
View SolutionThe number of elements in the set A∩B∩C, where
A={(x,y)∈R×R,|x|+|y|≥1}
B={(x,y)∈R×R,x2+y2≥1}
and C={(x,y)∈R×R: max |x|,|y|=1} is:
View SolutionLet A={(x,y)/y=ex,x∈R},B={(x,y)/y=e−x,x∈R}, then
View Solution
