If h(x)=2kx−1, what is the value of h(a)h(b)h(a+b) ?
4
2
1
0.5
The correct Answer is:D
To solve the problem, we need to find the value of h(a)h(b)h(a+b) given the function h(x)=2kx−1.
1. Define h(a):
h(a)=2ka−1
Here, we substitute x with a in the function definition.
2. Define h(b):
h(b)=2kb−1
Similarly, we substitute x with b in the function definition.
3. Define h(a+b):
h(a+b)=2k(a+b)−1
Here, we substitute x with a+b in the function definition.
4. Calculate h(a)h(b):
h(a)h(b)=(2ka−1)(2kb−1)=2(ka−1)+(kb−1)=2ka+kb−2
We add the exponents since the bases are the same.
5. Substitute h(a+b) into the expression:
h(a)h(b)h(a+b)=2ka+kb−22k(a+b)−1
6. Simplify the expression:
=2(ka+kb−2)−(k(a+b)−1)=2(ka+kb−2)−(ka+kb−k)=2−2+k
Here, we simplify the exponent by distributing the negative sign and combining like terms.
7. Final simplification:
=2k−2
8. Evaluate the expression:
Since we need to find the numerical value, we can express 2k−2 as:
=2k22=2k4
Without a specific value for k, we cannot simplify further, but we can evaluate it for specific cases if needed.
Conclusion:
The final expression for h(a)h(b)h(a+b) simplifies to 2k−2.
h(a)=2ka−1
Here, we substitute x with a in the function definition.
h(b)=2kb−1
Similarly, we substitute x with b in the function definition.
h(a+b)=2k(a+b)−1
Here, we substitute x with a+b in the function definition.
h(a)h(b)=(2ka−1)(2kb−1)=2(ka−1)+(kb−1)=2ka+kb−2
We add the exponents since the bases are the same.
h(a)h(b)h(a+b)=2ka+kb−22k(a+b)−1
=2(ka+kb−2)−(k(a+b)−1)=2(ka+kb−2)−(ka+kb−k)=2−2+k
Here, we simplify the exponent by distributing the negative sign and combining like terms.
=2k−2
Since we need to find the numerical value, we can express 2k−2 as:
=2k22=2k4
Without a specific value for k, we cannot simplify further, but we can evaluate it for specific cases if needed.
The final expression for h(a)h(b)h(a+b) simplifies to 2k−2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Suppose that h(x)=4x−5andh(b)=17. What is the value of b?
A4B5.5C10D17.5If point E(5,h) is on the line that contains A(0,1)andB(−2,−1), what is the value of h?
A−1B0C1D6
The figure above shows the graph of function h. If function f is defined by f(x)=h(2x)+1, what is the value of f(−1)?
View Solution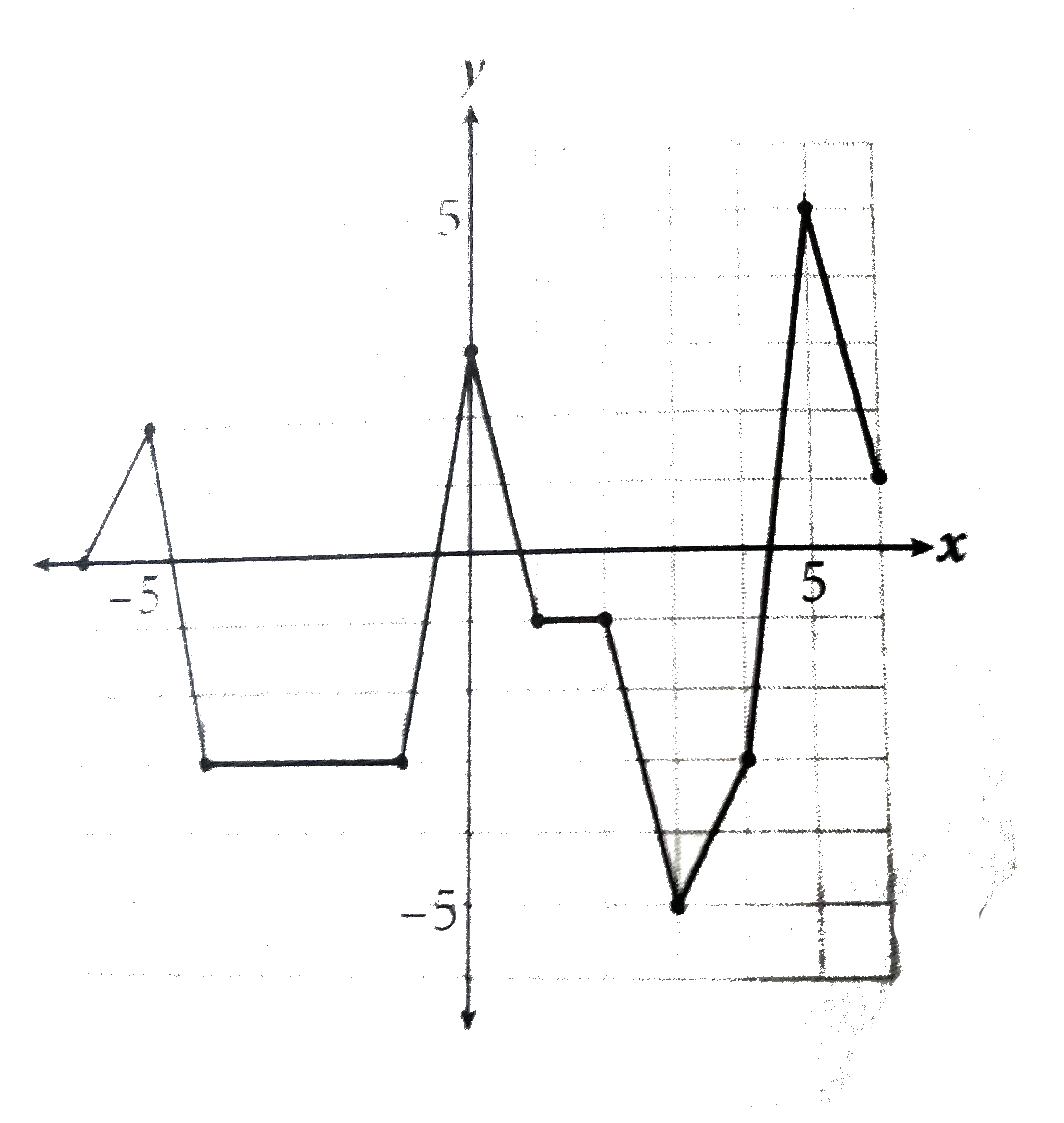
The figure above shows the graph of h(x) on the interval −6≤x≤6. If h(3)=a, what is the value of h(a)?
View SolutionLet g(x)=|x−2|andh(x)=g(g(x)) be two functions, then the value of h'(−1)+h'(1)+h'(3)+h'(5) is equal to (where, h' denotes the derivative of h)
View Solutiong(x)=2x
h(x)=8x2
if functions g and h are defined in the above equations, what is the value of g(h(3))?
View SolutionThe height h of cylinder equals the circumference of the cylinder. In terms of h, what is the volume of the cylinder? (a) h34π (b) h22π (c) h32 (d) πh3
View SolutionIf H1.H2....,Hn are n harmonic means between a and b(≠a), then the value of H1+aH1−a+Hn+bHn−b=
View Solution
