A shell of mass 2 kg and moving at a rate of 4m/s suddenly explodes into two equal fragments. The fragments go in directions inclined with the original line of motion with equal velocities. If the explosion imparts 48 J of translational kinetic energy to the fragments divided equall, the velocity (magnitude and direction both) of each fragment:
2m/s,45∘
8m/s,45∘
8m/s,60∘
2m/s,60∘
The correct Answer is:C
Initial horizontal momentum = Final horizontal momentum
2kg×r4/m/s=1kg×vcosθm/+1kg×vcosθm/s
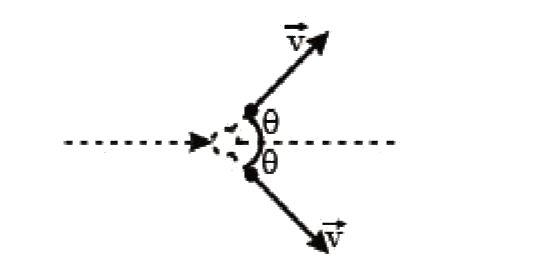
8=2vcosθ
vcosθ=4m/s……I
The initial kinetic energy of the shell
=12×2kg×(4m/s)2
=16J
An amount of 48J is imparted by explosion. Thus, the total energy of the fragments is 64J, i.e. each fragment has 32 J kinetic energy.
=12×1kg×v2=32J
v2=64
v=8m/s
and vcosθ=4
cosθ=12⇒θ=60∘
Topper's Solved these Questions
Similar Questions
A shell of mass 2kg moving at a rate of 4m/s suddenly explodes into two equal fragments. The fragments go in directions inclined with the original line of motion with equal velocities, If the explosion imparts 48J of translational kinetic energy to the fragments, find the velocity and direction of each fragment.
View SolutionA bomb explodes in mid air into two equal fragments . What is the angle between their directions of motion ?
View Solution
Knowledge Check
A shell of mass 2 kg and moving at a rate of 4 m/s suddenly explodes into two equal fragments. The fragments go in directions inclined with the original line of motion with equal velocities. If the explosion imparts 48 J of translational kinetic energy to the fragments divided equally, the velocity (magnitude and direction both) of each fragment :-
A2 m/s , 45∘B8 m/s , 45∘C8 m/s , 60∘D2 m/s , 60∘A srtone of mass m1 moving at uniform speed v suddenly explodes into two fragments. If the fragment of mass m2 is at rest, the speed of the other fragment is
Am1v(m1−m2)Bm2vm1−m2Cm1v(m1+m2)Dm1vm2A shell of mass 20kg at rest explodes into two fragments whose masses are in the ratio 2:3. The smaller fragment moves with a velocity of 6m/s. The kinetic energy of the larger fragment is
A96JB216JC144JD360J
A particles has velocity 40 m/s is exploded into two equal parts one with 60 m/s in same direction. The ratio of kinetic energy of individual particles after explosion is K:4, Then K is
View SolutionA shell of mass 20kg at rest explodes into two fragments whose masses are in the ratio 2:3. The smaller fragment moves with a velocity of 6m/s. The kinetic energy of the larger fragment is
View SolutionA bomb explodes in air in two equal fragments. If one of the fragments is moving vertically upwards with velocity v0, then the other fragment is moving-
View SolutionAt high altitude , a body explodes at rest into two equal fragments with one fragment receiving horizontal velocity of 10m/s. Time taken by the two radius vectors connecting of explosion to fragments to make 90∘ is
View SolutionAt a certain height a shell at rest explodes into two equal fragments one of the fragments receives a horizontal velocity u.The time interval after which the velocity vectors will be inclined at 120∘ to each other is
View Solution
