A radioactive nucleus A with a half-life T, decays into a nucleus B. At t=0, there is no nucleus B. At sometime t, the ratio of the number of B to that of A is 0.3. Then, t is given by -

(A) $t = \frac{T}{2} \frac{log 2}{log 1.3}$
(B) $t = T \frac{log 1.3}{log 2}$
(C) $t = T log (1.3)$
(D) $t = \frac{T}{log (1.3)}$

$t = T log (1.3)$

$t = \frac{T}{log (1.3)}$

$t = \frac{T}{2} \frac{log 2}{log 1.3}$

$t = T \frac{log 1.3}{log 2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

A radioactive nucleus A with a half life T, decays into nucleus B. At t=0, there is no nucleus B. At somewhat t, the ratio of the number of B to that of A is 0.3 . Then, t is given by
A $t = T log (1.3)$
B $t = \frac{T}{log (1.3)}$
C $t = \frac{T}{2} \frac{log 2}{log 1.3}$
D $t = T \frac{log 1.3}{log 2}$
In the circuit shown the key $(K)$ is closed at $t = 0$ , the current through the key at the instant $t = 10^{- 3} ln, 2$ is

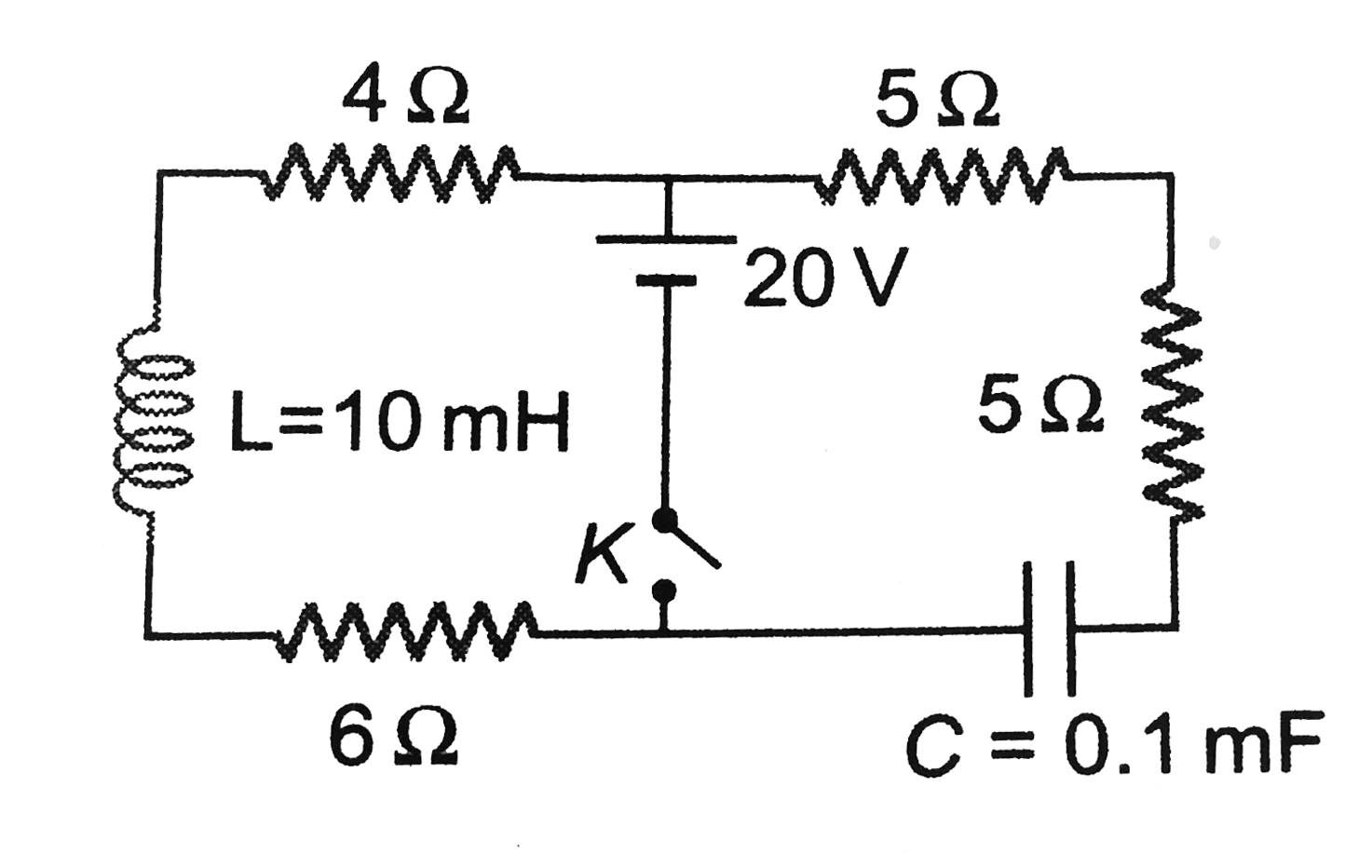A $2 A$
B $8 A$
C $4 A$
Dzero
The half-life of a radioactive nucleus is $50$ days. The time interval $(t_{2} - t_{1})$ between the time $t_{2}$ when $\frac{2}{3}$ of it has decayed and the time $t_{1}$ when $\frac{1}{3}$ of it had decayed is
A30 days
B50 days
C60 days
D15 days