The stopping potential are V1 and V2. Calculate the (V1−V2), if the λ1 and λ2 are wavelength of incident lights, respectively.
hce(1λ1−1λ2)
hce(1λ1+1λ1)
ehc(1λ1+1λ2)
ehc(1λ1−1λ2)
The correct Answer is:A
eV1=hcλ1 and eV2=hcλ2⇒(V1−V2)=hce(1λ1−1λ2)
Topper's Solved these Questions
- CATHODE RAY, PHOTOELECTRIC EFFECT AND X-RAYSBook:BITSAT GUIDEChapter:CATHODE RAY, PHOTOELECTRIC EFFECT AND X-RAYSExercise:BITSAT Archives
Explore 6 Videos
- ATOMIC STRUCTUREBook:BITSAT GUIDEChapter:ATOMIC STRUCTUREExercise:BITSAT Archives
Explore 4 Videos
- CURRENT ELECTRICITYBook:BITSAT GUIDEChapter:CURRENT ELECTRICITYExercise:Bitsat Archives
Explore 26 Videos
Similar Questions
Figure shows the stopping potential (V0) for the photo electron vers (1λ) graph, for two metals A and B, λ being the wavelength of incident light.
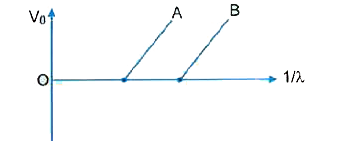
How is the value of Planck's constant determined from the graph ?
View SolutionFigure shows the stopping potential (V0) for the photo electron vers (1λ) graph, for two metals A and B, λ being the wavelength of incident light.

If the distance between the light source and the surface of metal A is increased, how will the stopping potential for the electrons emitted from it be affected ? Justify your answer.
View Solution
Knowledge Check
if λv',λx and λm represent the wavelengths of visible light X-rays and microwaves respectively then:
Aλm>λx>λvBλk>λm>λxCλm>λv>λxDλy>λx>λmIf λv,λx and λm represents the wavelength of visible light X-ray and microwave respectively then :
Aλm>λv>λxBλm>λx>λvCλv>λm>λxDλv>λx>λmFrom the above figure the values of stopping potentials for M1 and M2 for a frequency v3(>v02) of the incident radiatioins are V1 and V2 respectively. Then the slope of the line is equal to
AV2−V1v02−v01BV1−V2v02−v01CV2v02−v01DV1v02−v01
The potential energy of a particle of mass m is given by
V(x)=<br>lambda_(1) and lambda_(2) arethede−Brog≤wave≤n>hofthepartic≤,when= lex le 1 andxgt 1repectively,ifthe→tale≠rgyofpartic≤is2 E_(0) findλ1/λ2
View SolutionIn an experiment of photoelectric effect the stopping potential was measured to be V1 and V2 with incident light of wavelength λ and λ2, respectively. The relation between V1 and V2 is:
View SolutionIn a photoelectric effect measurement, the stoppingg potential for a given metal is found to be V0 volt, when radiation of wavelength λ0 is used. If radiation of wavelength 2λ0 is used with the same metal, then the stopping potential (in V) will be
View SolutionA surface receives light of wavelength λ1=450 nm, causing the ejection of photo-electrons for which the stopping potential is VS1=0.2V. If the radiations of wavelength λ2=120 nm are now incident on the surface, the threshold frequency for the surface is
View SolutionThe stopping potential for a certain photocell is 1.2 V when light of wavelength λ1=400 nm is used. It is 0.6 V, when light of wavelength λ2=500 nm is used. What would be the stopping potential, when both λ1 and λ2 are incident simultaneously ?
View Solution