A steel bar 10.0 cm long is welded end to end to a copper bae 20.0 cm long. Both bars are insulated perfectly on their sides . Each bar has a separate cross-section, 2.00 cm on a side . The free end of the steel bar is maintained at 100∘C by placing it in contact with ice . find the temperature at the junction of the two bars and the total rate of heat flow . thermal conductivity of steel =50.2Wm−1K−1.thermal conductivity of copper =385Wm−1K−1.
To solve the problem, we need to find the temperature at the junction of the steel and copper bars and the total rate of heat flow. We will use the concept of thermal conductivity and the formula for heat transfer through a material.
Step 1: Identify the given values
- Length of steel bar, Ls=10.0cm=0.1m
- Length of copper bar, Lc=20.0cm=0.2m
- Thermal conductivity of steel, ks=50.2W/m⋅K
- Thermal conductivity of copper, kc=385W/m⋅K
- Temperature at the free end of the steel bar, Ts=100∘C
- Temperature at the free end of the copper bar, Tc=0∘C
Step 2: Set up the heat flow equations
The heat flow H through each bar can be expressed using the formula:
H=k⋅A⋅(T1−T2)L
Where:
- H is the heat flow rate (W)
- k is the thermal conductivity (W/m·K)
- A is the cross-sectional area (m²)
- T1 and T2 are the temperatures at the two ends (K)
- L is the length of the bar (m)
Step 3: Calculate the cross-sectional area
The cross-sectional area A of the bars is given as:
A=(2.00cm)2=(0.02m)2=0.0004m2
Step 4: Write the heat flow equations for both bars
For the steel bar:
Hs=ks⋅A⋅(Ts−T)Ls
For the copper bar:
Hc=kc⋅A⋅(T−Tc)Lc
Step 5: Set the heat flows equal to each other
Since the heat flow through both bars is the same at steady state:
Hs=Hc
Substituting the equations:
ks⋅A⋅(Ts−T)Ls=kc⋅A⋅(T−Tc)Lc
Step 6: Simplify and solve for T
Cancel A from both sides:
ks(Ts−T)Ls=kc(T−Tc)Lc
Substituting the known values:
50.2(100−T)0.1=385(T−0)0.2
Cross-multiplying gives:
50.2⋅200(100−T)=385⋅100(T)
This simplifies to:
10040(100−T)=38500T
Expanding and rearranging:
1004000−10040T=38500T
1004000=10040T+38500T
1004000=48540T
T=100400048540≈20.67∘C
Step 7: Calculate the total rate of heat flow
Substituting T back into either heat flow equation, we can use the steel bar equation:
Hs=50.2⋅0.0004⋅(100−20.67)0.1
Calculating:
Hs=50.2⋅0.0004⋅79.330.1≈160.06W
Final Answers
- Temperature at the junction T≈20.67∘C
- Total rate of heat flow H≈160.06W
- Length of steel bar, Ls=10.0cm=0.1m
- Length of copper bar, Lc=20.0cm=0.2m
- Thermal conductivity of steel, ks=50.2W/m⋅K
- Thermal conductivity of copper, kc=385W/m⋅K
- Temperature at the free end of the steel bar, Ts=100∘C
- Temperature at the free end of the copper bar, Tc=0∘C
The heat flow H through each bar can be expressed using the formula:
H=k⋅A⋅(T1−T2)L
Where:
- H is the heat flow rate (W)
- k is the thermal conductivity (W/m·K)
- A is the cross-sectional area (m²)
- T1 and T2 are the temperatures at the two ends (K)
- L is the length of the bar (m)
The cross-sectional area A of the bars is given as:
A=(2.00cm)2=(0.02m)2=0.0004m2
For the steel bar:
Hs=ks⋅A⋅(Ts−T)Ls
For the copper bar:
Hc=kc⋅A⋅(T−Tc)Lc
Since the heat flow through both bars is the same at steady state:
Hs=Hc
ks⋅A⋅(Ts−T)Ls=kc⋅A⋅(T−Tc)Lc
Cancel A from both sides:
ks(Ts−T)Ls=kc(T−Tc)Lc
50.2(100−T)0.1=385(T−0)0.2
50.2⋅200(100−T)=385⋅100(T)
10040(100−T)=38500T
1004000−10040T=38500T
1004000=10040T+38500T
1004000=48540T
T=100400048540≈20.67∘C
Substituting T back into either heat flow equation, we can use the steel bar equation:
Hs=50.2⋅0.0004⋅(100−20.67)0.1
Calculating:
Hs=50.2⋅0.0004⋅79.330.1≈160.06W
- Temperature at the junction T≈20.67∘C
- Total rate of heat flow H≈160.06W
Topper's Solved these Questions
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEYChapter:CALORIMETRY AND HEAT TRANSFERExercise:Check point 16.1
Explore 10 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEYChapter:CALORIMETRY AND HEAT TRANSFERExercise:Check point 16.2
Explore 10 Videos
- CALORIMETRY & HEAT TRANSFERBook:DC PANDEYChapter:CALORIMETRY & HEAT TRANSFERExercise:Level 2 Subjective
Explore 14 Videos
- CENTRE OF MASSBook:DC PANDEYChapter:CENTRE OF MASSExercise:Medical entrances gallery
Explore 27 Videos
Similar Questions
A steel bar 10.0 cm long is welded end to end to a copper bar 20.0 cm long. Both bars are insulated perfectly on their sides. Each bar has a square cross-section, 20.00 cm on a side. The free end of the steel bar is maintained at 100∘C by placing it in contact with steam, and the free end of the copper bar is maintained at 0∘C by placing it in contact with ice. find the temperature at the junction of the two bars and the total rate of heat flow.
View SolutionA bar of copper of length 75 cm and a bar of steel of length 125 cm are joined together end to end . Both are of circular cross - section with diameter 2 cm . The free ends of the copper and steel bars are maintained at 100∘C and 0∘C respectively . The surface of the bars are thermally insulted . What is the temperature of the copper - steel junction ? What is the heat transmitted per unit time across the junction ? Thermal conductivty of copper 9.2×10−2kcalm−1∘C−1s−1 and that of steel is 1.1×10−2kcalm−1s−1(∘)C−1.
View Solution
Knowledge Check
Cylinder copper rod of length 1m and a cylinder steel rod of length 1.5m are joined together end to end. The cross sectional area of eac rod is 3.14cm. The free ends of steel rod and copper rods are maintained at 0∘C and 100∘C respectively. The surfaces of rods are thermally insulated. Find the temperature of copper steel junction. (Given thermal conductivity of steel =46J/m−s.∘C and the thermal conductivity of copper =386J/m−s.∘C)
A40∘B60∘C93∘D80.64∘CA cylindrical steel rod of length 0.10 m and thermal conductivity 50W.m−1K−1 is welded end to end to copper rod of thermal conductivity 400W.m−1.K−1 and of the same area of cross section but 0.20 m long. The free end of the steel rod is maintained at 100∘C and that of the copper and at 0∘C. Assuming that the rods are perfectly insulated from the surrounding, the temperature at the junction of the two rods–
A20∘CB30∘CC40∘CD50∘CA large cylindrical rod of length L is made by joining two identical rods of copper and steel of length (L2) each. The rods are completely insulated from the surroundings. If the free end of copper rod is maintained at 100∘C and that of steel at 0∘C then the temperature of junction is (Thermal conductivity of copper is 9 times that of steel)
A90∘CB50∘CC10∘CD67∘C
A bar of copper of length 75cm and a abr of steel of length 125cm are joined together end to end. Both are of circular cross section with diameter 2cm. The free ends of the copper and the steel bors are maintained at 100∘C and 0^(@)Crespectively.Thecurvedsurfaceofthebors.arethermally∈sa––ted.Wˆisthetemperatureofthecoppe−steeljunction?Wˆistheamountofheattransmiedperunittimeacrossthejunction?Thermalconductivityofcopperis386Js^(-1)m^(-1)^(∘)C−1 and that of steel is 46Js^(-1)m^(-1)(@)C^(-1)
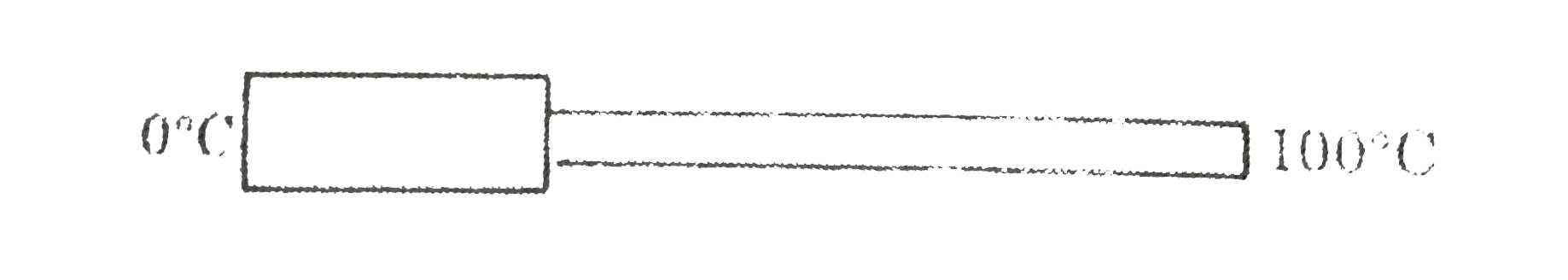
View SolutionIn fig, two bars of the same metal are connected. The length of the first bar is half of that of the second, but the cross-sectional area is double. What is the temperature of the junction of the bars ?
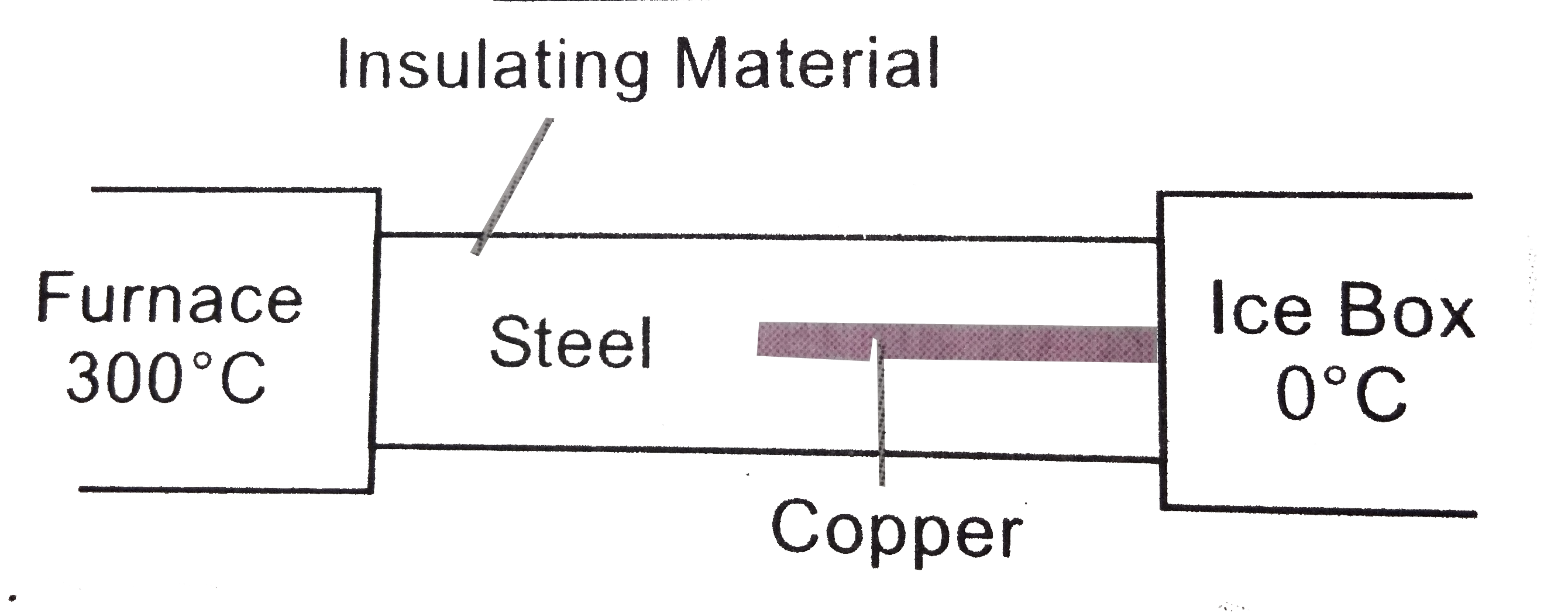
View SolutionWhat is the temperature of the steel-copper junction in the steady state system show in Fig. 7(e).17? Length of steel rod = 15.0 cm, length of the copper rod = 10.0 cm, temperature of the furnace =300∘C, temperature of other end 0∘C. The are of cross-section of the steel rod is twice that of the copper rod. (Thermal conductivity of steel =50.2js−1m−1K−1 and of copper =3895js−1m−1K−1).
View Solution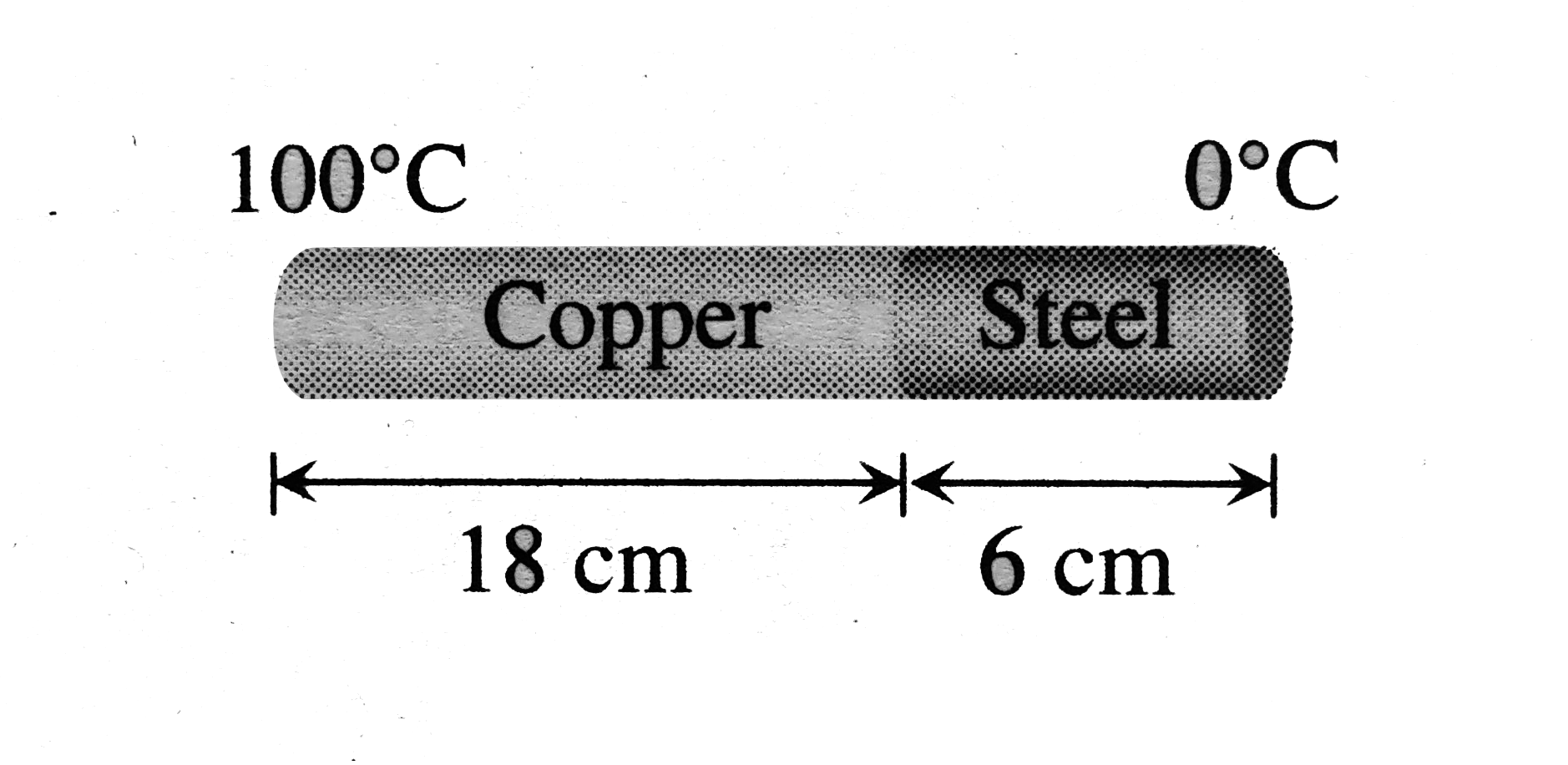
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?
View Solution
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?
View Solution
