A body rotating with uniform angular acceleration covers 100π (radian) in the first 5 s after the start. Its angular speed at the end of 5 s (in rad/s) is
40π
30π
20π
10π
The correct Answer is:C
To solve the problem step by step, let's break it down:
Step 1: Identify the given values
- Angular displacement (θ) = 100π radians
- Initial angular speed (ω0) = 0 rad/s (the body starts from rest)
- Time (t) = 5 seconds
Step 2: Use the second equation of motion for rotational motion
The second equation of motion for rotational motion is:
θ=ω0t+12αt2
Since the initial angular speed ω0 is 0, the equation simplifies to:
θ=12αt2
Step 3: Rearrange the equation to solve for angular acceleration (α)
Rearranging the equation gives:
α=2θt2
Step 4: Substitute the known values into the equation
Substituting the values we have:
α=2×100π52
Calculating 52:
52=25
Now substituting this back into the equation:
α=200π25=8πrad/s2
Step 5: Use the angular acceleration to find the final angular speed (ω)
The formula to find the final angular speed is:
ω=ω0+αt
Substituting the known values:
ω=0+(8π)(5)
Calculating this gives:
ω=40πrad/s
Final Answer
The angular speed at the end of 5 seconds is:
ω=40πrad/s
---
- Angular displacement (θ) = 100π radians
- Initial angular speed (ω0) = 0 rad/s (the body starts from rest)
- Time (t) = 5 seconds
The second equation of motion for rotational motion is:
θ=ω0t+12αt2
Since the initial angular speed ω0 is 0, the equation simplifies to:
θ=12αt2
Rearranging the equation gives:
α=2θt2
Substituting the values we have:
α=2×100π52
Calculating 52:
52=25
Now substituting this back into the equation:
α=200π25=8πrad/s2
The formula to find the final angular speed is:
ω=ω0+αt
Substituting the known values:
ω=0+(8π)(5)
Calculating this gives:
ω=40πrad/s
The angular speed at the end of 5 seconds is:
ω=40πrad/s
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - Check point 9.220videos
ROTATION
- BOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - Check point 9.315videos
ROTATION
- BOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises39videos
ROTATION
- BOOK - DC PANDEYCHAPTER - RAY OPTICSEXERCISE - Integer type q.15videos
RAY OPTICS
- BOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions2videos
ROTATIONAL MECHANICS
Similar Questions
A wheel rotating wilth unifrom angular acceleration covers 50 revolutions in the first five seconds after the start. Find the angular acceleration and the angular velocity at the end of five seconds.
View SolutionA wheel rotating with uniform angular acceleration covers 50 revolutions in the first five seconds after the start. Find the angular acceleration and the angular velocity at the end of five seconds.
View Solution
Knowledge Check
- Question 1 - Select One
A disc initially at rest , is rotated about its axis with uniform angular acceleration . In the first 2 s, it rotates an angle θ . In the next 2s, the disc rotates through an angle
AθB2θC3θD4θ - Question 2 - Select One
A body rotates about a fixed axis with an angular acceleration of 3rad/s2 The angle rotated by it during the time when its angular velocity increases frm 10 rad/s to 20 rad/s (in radian) is
A50B100C150D200 - Question 3 - Select One
A particle starts from rest and moves with an angular acceleration of 3 rad/s2 in circle of radius 3 m . Its linear speed after 5 seconds will be
A15 m/sB30m/sC45m/sD7.5m/s
Starting from rest a wheel rotates with uniform angular acceleration 2πrads−2 . After 4s ,if the angular acceleration ceases to act,its angular displacement in the next 4s is?
View SolutionStarting from rest a wheel rotates with uniform angular acceleration 2π rads−2.After 4s ,if the angular acceleration ceases to act,its angular displacement in the next 4s is?
View SolutionStarting from rest, a disk rotates about its central axis with constant angular acceleration. In 5.0 s, it rotates 20 rad. During that time, what are the magnitudes of (a) the angular acceleration and (b) the average angular velocity? ( c) What is the instantaneous angular velocity of the disk at the end of the 5.0 s? (d) With the angular acceleration unchanged, through what additional angle will the disk turn during the next 5.0 s?
View SolutionA particle starts moving in a non-uniform circular motion, lies angular acceleration as shown in figure. The angular velocity at the end of 4 radian is given by ω rad/s then find the value of ω.
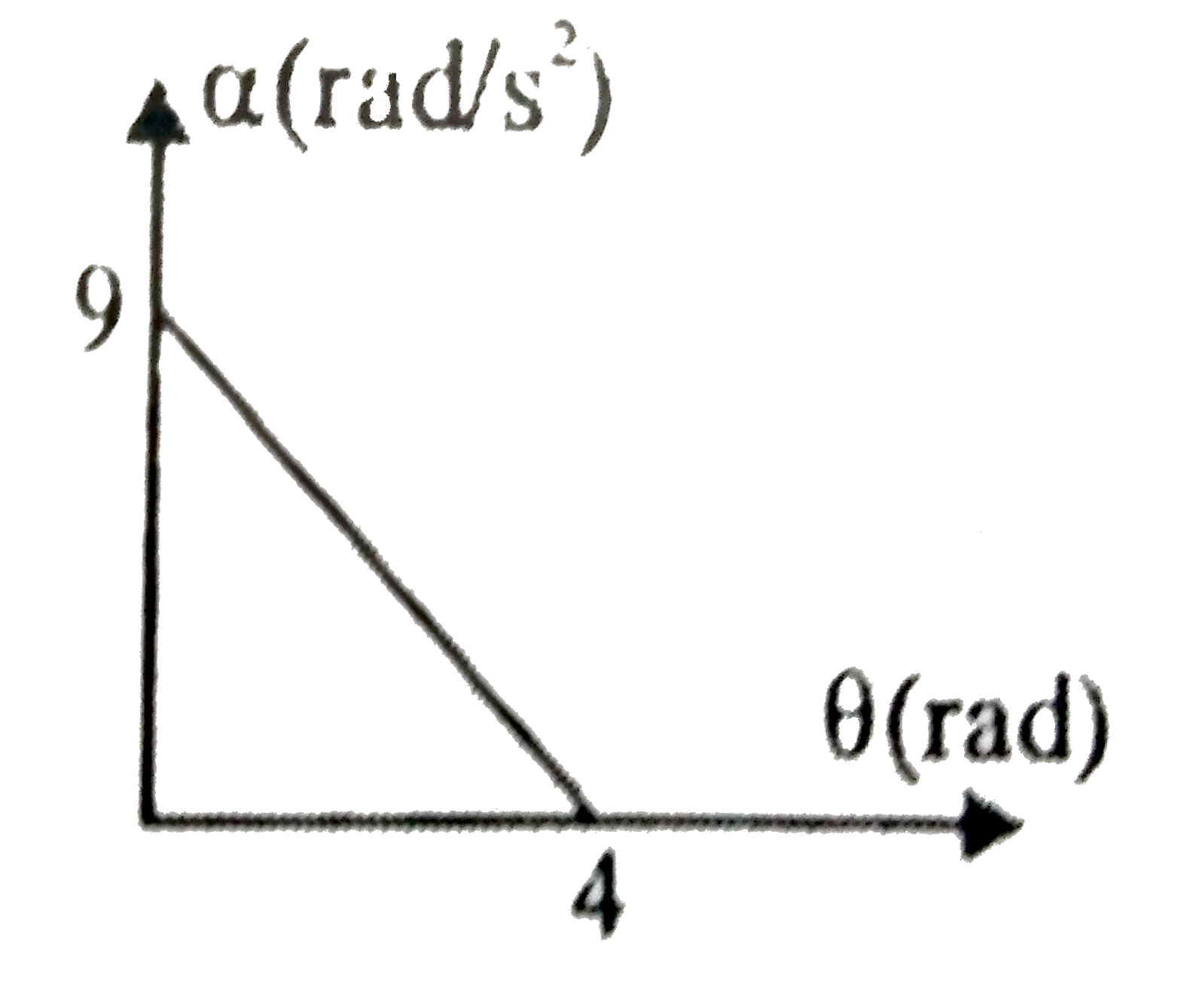 View Solution
View SolutionA disc starts rotating with constant angular acceleration of πrads2 about a fixed axis perpendicular to its plane and through its centre .
(a) Find the angular velocity of the disc after 4s .
(b) Find the angular displacement of the disc after 4 s and
(c ) Find number d of turns accomplished by the disc in 4 s .View Solution

