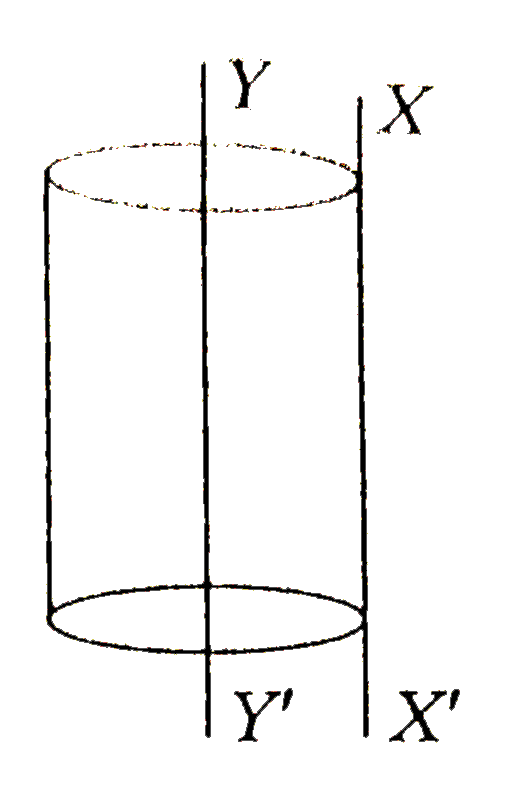
The mass of the cylinder is 25 kg and radius of cylinder is 5 m. Find the moment of inertia of a solid cylinder about a tangent to the cylinder.
The moment of inertia of a cylinder about its own axis (YY')
=12MR2
Applying the theorem of parallel axes, the moment of inertia of the cylinder about tangent (XX') is
It=I+MR2=12MR2+MR2⇒It=32MR2
Here, M=25kg,R=5m∴It=32(25)(5)=937.5kg-m2
Topper's Solved these Questions
- ROTATIONBook:DC PANDEYChapter:ROTATIONExercise:Check point 9.1
Explore 20 Videos
- ROTATIONBook:DC PANDEYChapter:ROTATIONExercise:Check point 9.2
Explore 20 Videos
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:Integer type q.
Explore 15 Videos
- ROTATIONAL MECHANICSBook:DC PANDEYChapter:ROTATIONAL MECHANICSExercise:Subjective Questions
Explore 2 Videos
Similar Questions
Knowledge Check
What is moment of inertia of a cylinder of radius r, along its height?
Amr2Bmr22C2mr25Dmr25If the volume of the cylinder is 3564 cm3. The external radius of the cylinder is 12 cm and thickness is 3 cm. Then find the length of the cylinder.
A14 cmB18 cmC16 cmD10 cmMoment of inertia of a hollow cylinder of mass M and radius R, about the axis of cylinder is
A12MR2BMR2C23MR2D25MR2
The total surface area of a cylinder is 1540cm2. The height of cylinder is 4 times the radius of the base. Find the height of the cylinder.
View SolutionDerive an expression for the moment of inertia of a (i) hollow cylinder (ii) solid cylinder about its own axis.
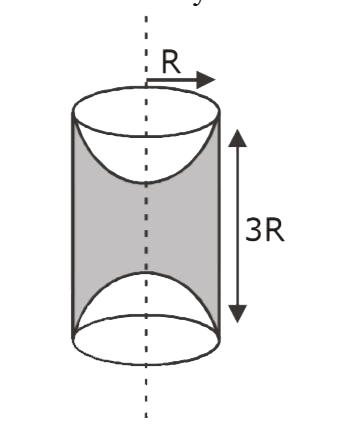
View SolutionA solid cylinder of radius R & length 3R is made of a metarial having density ρ . Now two hemispheres each of radius R is removed from two ends of cylinder as shown in figure. Determine the moment of inertia of this object about axis of cylinder?
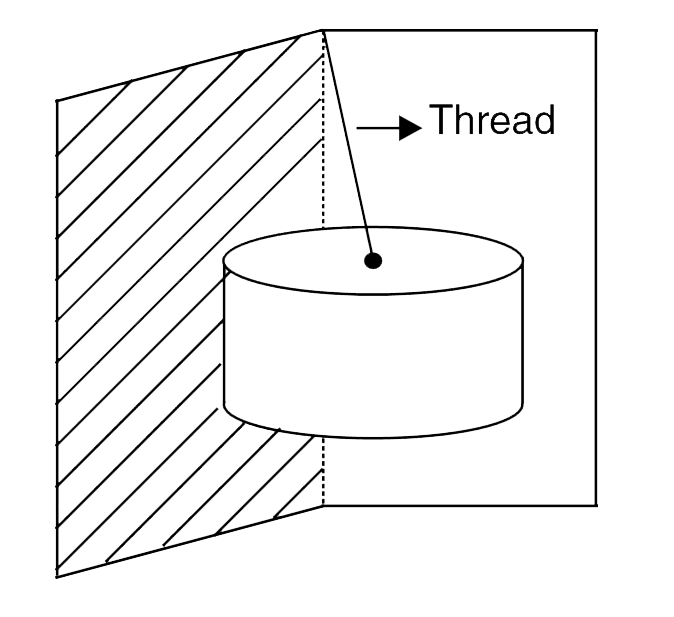
View SolutionA cylinder of mass M and radius r is suspended at the corner of a room. Length of the thread is twice the radius of the cylinder. Find the tension in the thread and normal force applied by each wall on the cylinder assuming the walls to be smooth.
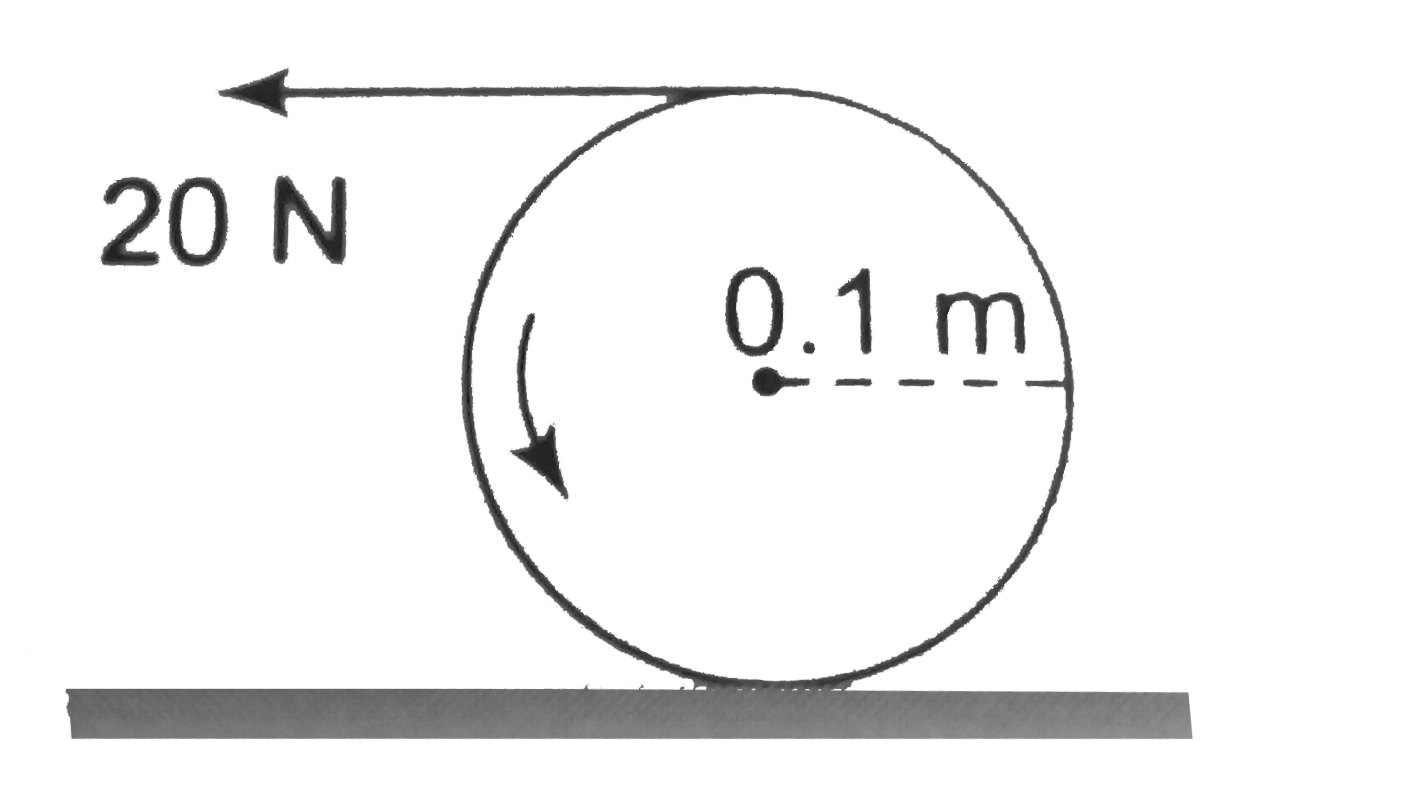
View SolutionThe rope shown in figure is wound around a cylinder of mass 4kg and moment of inertia 0.02kgm2 about the cylinder axis. If the cylinder rolls without slipping, then the linear acceleration of its centre of mass is.
 .
.
View Solution