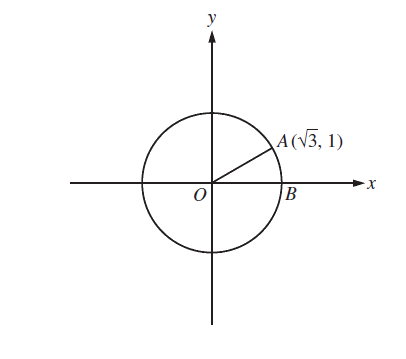
What is the measure in degrees of an angle that is π4 radians?
4∘
25∘
45∘
90∘
The correct Answer is:C
To convert an angle from radians to degrees, we can use the relationship that π radians is equal to 180 degrees.
1. Identify the given angle in radians:
The angle given is π4 radians.
2. Use the conversion factor:
We know that:
π radians=180 degrees
3. Set up the conversion for π4 radians:
To convert π4 radians to degrees, we can set up the equation:
π4 radians=180 degreesπ⋅π4
4. Simplify the equation:
By multiplying, we have:
1804 degrees=45 degrees
5. Conclusion:
Therefore, the measure of the angle π4 radians is equal to 45 degrees.
Final Answer:
The measure in degrees of an angle that is π4 radians is 45 degrees.
The angle given is π4 radians.
We know that:
π radians=180 degrees
To convert π4 radians to degrees, we can set up the equation:
π4 radians=180 degreesπ⋅π4
By multiplying, we have:
1804 degrees=45 degrees
Therefore, the measure of the angle π4 radians is equal to 45 degrees.
The measure in degrees of an angle that is π4 radians is 45 degrees.
Topper's Solved these Questions
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #7
3 Videos
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #8
3 Videos
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #5
3 Videos
- ADVANCED ARITHMETICBOOK - PRINCETONCHAPTER - ADVANCED ARITHMETICEXERCISE - Examples
25 Videos
Similar Questions
Knowledge Check
What is the degree measure of an angle that measures 7π15 radians ?
A(360−7π15)∘B(180−7π15)∘C252∘D84∘In a circle with center O, the measure of central angle POQ is 3π2 radians. The length of the arc formed by central angle POQ is that fraction of the circumference of the circle?
A316B38C34D32What is the angle measure of 4 radians ?
A114.591∘B141.372∘C229.183∘D282.743∘
(a) In a right angled triangle , the difference between two acute angles is π5 in radians Express the angles in degrees.
(b) Evaluate : 6cosπ9−8cos3π9
View SolutionCan we use the equations of rotational motion if θ is measured in degrees and not in radian ?
View SolutionThe angles in a triangle are in A.P. and the ratio of the smallest angle in degrees to the greatest angle in radians is 60:ϕ Find the angle of the triangle in degrees and radians.
View SolutionIF n is a constant equal to the number of degrees in an angle measuring 3π radians, what is the value of n?
View Solution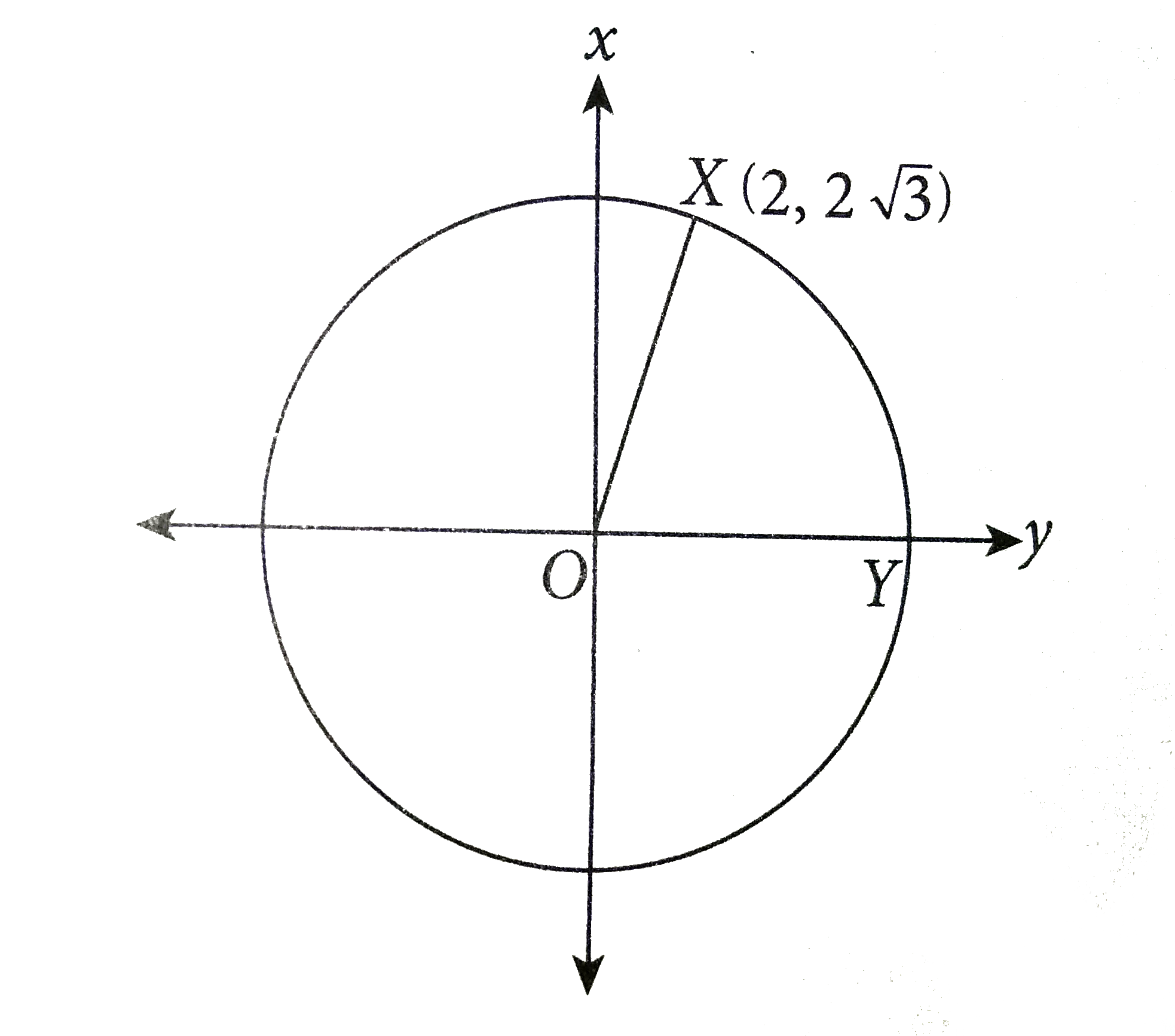
In the xy-plane above, the circle has center O, and the measure of ∠XOY is πn radians. What is the value of n?
View Solution