If the equation above is true, then what is the positive value of the expression 10√x−15 ?
A
20
B
25
C
30
D
35
Video Solution
Text Solution
The correct Answer is:A
The presence of 4,9 and 16- all perfect squares -is a big clue. Notye that 4x−12√x+9 is an instance of the classic quadratic (a−b)2=a2−2ab+b2. Use this shortcut to factor the equation ans se where that leads you:
4x−12√x+9=16
(2√x−3)2=16
2√x−3=±4
The question asks for 10√x−15, which happens to be 5 times the quantity on the left side of the last equation above, so multiply the positive result, 4 by 5 to get 20. The correct answer is (A).
|
Topper's Solved these Questions
- QUADRATICSBOOK - KAPLANCHAPTER - QUADRATICSEXERCISE - SOLVING QUADRATICS BY FACTORING
1 Video
- QUADRATICSBOOK - KAPLANCHAPTER - QUADRATICSEXERCISE - CLASSIC QUADRATICS
1 Video
- QUADRATICSBOOK - KAPLANCHAPTER - QUADRATICSEXERCISE - SYSTEMS OF QUADRATIC AND LINEAR EQUATIONS
1 Video
- PAIRED PASSAGES AND PRIMARY SOURCE PASSAGES BOOK - KAPLANCHAPTER - PAIRED PASSAGES AND PRIMARY SOURCE PASSAGES EXERCISE - HOW MUCH HAVE YOU LEARNED
11 Videos
- SAT MATH: TIMING ANS SECTION MANAGEMENT STRATEGIESBOOK - KAPLANCHAPTER - SAT MATH: TIMING ANS SECTION MANAGEMENT STRATEGIESEXERCISE - TRY ON YOU OWN
5 Videos
Similar Questions
Knowledge Check
Fow what set of values of x is the expression |3x+4|<0 true?
A−43<0<xBx<−43CNo real numbersDAll real numbery=x2−4,x+y=2
Based on the system of equations above , what is the minimum value of the product xy ?A2B0C−5D−15The graph of the equation above is a parabola in the xy-plane. If k>0, what is the minimum value of y expressed in terms of k?
A−7kB−16kC−25kD−73k
If x,y∈R satisfy the equation x2+y2−4x−2y+5=0, then the value of the expression (√x−√y)2+4√xy(x+√xy) is
View Solutionx8+y5=3140
In the equation above, if x and y are positive integers, what is the value of x+y?
View Solutiona−ba=c
In the equation above, if a is negative and b is positive, which of the following must be true?
View Solution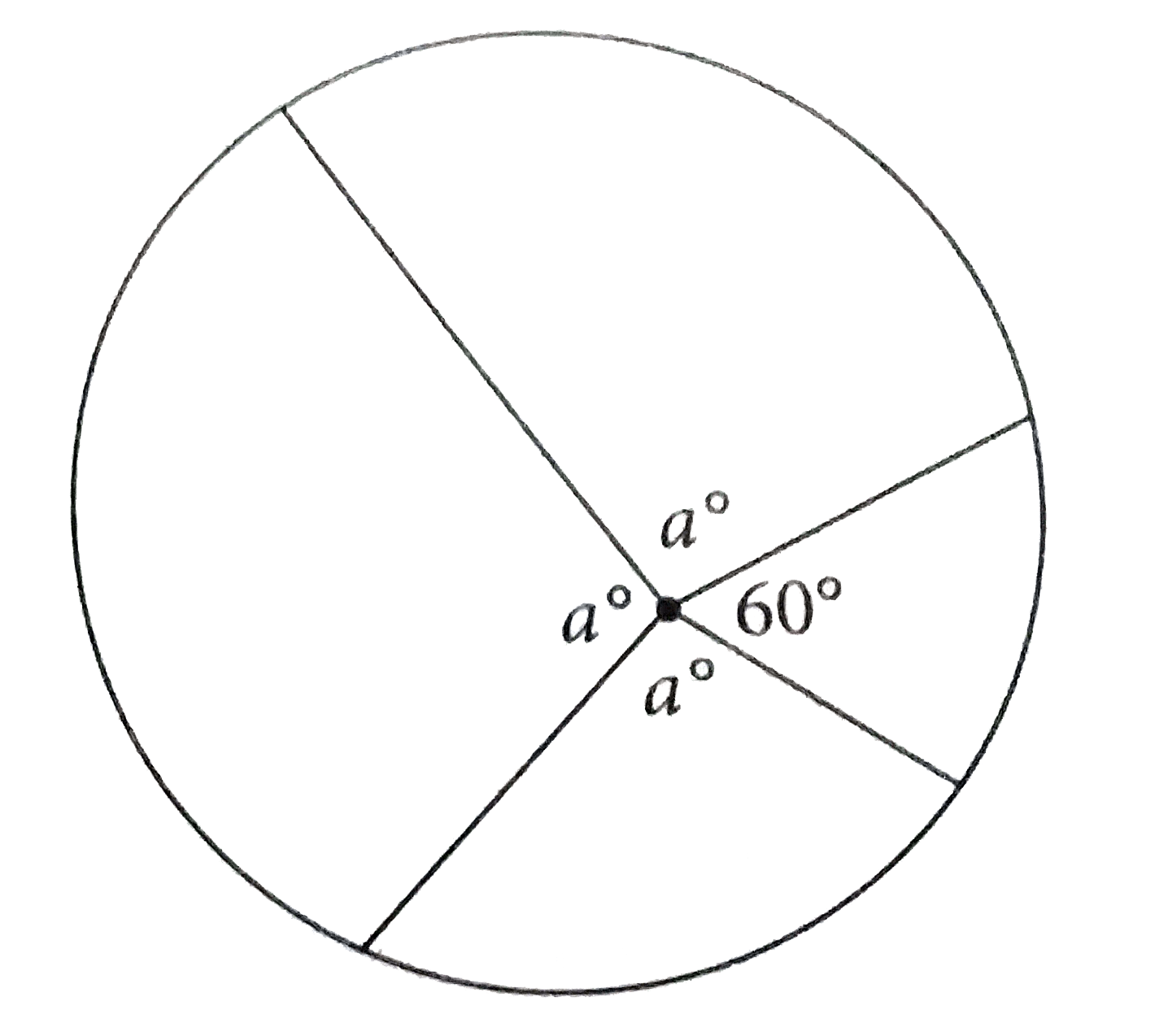
In the figure above , what is the value of a?
View Solution(ax+4)(bx−1)=21x2+kx−4
If the equation above is true for all values of x and a+b=10, what are the possible values for the constant k?
View Solution
