In the xy-plane, the point (4,7) lies on the line t, which is perpendicular to the line y=−43x+6. What is the equation of line t ?
A
y=34x+4
B
y=−43x+4
C
y=34x+7
D
y=−34x+4
Video Solution
Text Solution
The correct Answer is:A
The first useful pices of information is that the slope of the line perpendicular to line t is −43. Perpendicular lines have negative reciprocal slopes, so the slope of line t is 34. Eliminate (B) and (D) because they have the incorrect slopes.
Plug the values for the slope and the coordinate point (4,7) into the slope-intercept equation to solve for b:
7=34(4)+b
7=3+b
7−3=b
b=4
Eliminate (C ) because it does not have the correct y-intercept. Choice (A) is correct.
|
Topper's Solved these Questions
- LINEAR EQUATIONS AND GRAPHSBook:KAPLANChapter:LINEAR EQUATIONS AND GRAPHSExercise:SOLVING EQUATIONS
Explore 1 Video
- LINEAR EQUATIONS AND GRAPHSBook:KAPLANChapter:LINEAR EQUATIONS AND GRAPHSExercise:WORD PROBLEMS
Explore 1 Video
- LINEAR EQUATIONS AND GRAPHSBook:KAPLANChapter:LINEAR EQUATIONS AND GRAPHSExercise:LINEAR GRAPH
Explore 1 Video
- INEQUALITIESBook:KAPLANChapter:INEQUALITIESExercise:MODELING REAL-LIFE SITUATIONS WITH INEQUALITIES
Explore 1 Video
- PAIRED PASSAGES AND PRIMARY SOURCE PASSAGES Book:KAPLANChapter:PAIRED PASSAGES AND PRIMARY SOURCE PASSAGES Exercise:HOW MUCH HAVE YOU LEARNED
Explore 11 Videos
Similar Questions
Knowledge Check
In the xy-plane, the point (p, r) lies on the line with equation y = x + b, where b is a constant. The point with coordinates (2p, 5r) lies on the line with equation y = 2x + b. If p ≠ 0, what is the value of rp ?
A25B34C43D52
Is the line 3x+4y+7=0 perpendicular to the line 28x−21y+50=0?
View SolutionFind the equation of the line that has y-intercept −3 and is perpendicular to the line 3x+5y=4.
View SolutionThe point P is the foot of the perpendicular from A(0, t) to the line whose equation is y=tx. Determine
the equation of the line AP
View SolutionAt what points on the curve y=x2−4x+5 is the tangent perpendicular to the line 2y+x=7 ?
View SolutionFind the equation of a line passing through the point (−1,0) and perpendicular to the line x+5y=4.
View SolutionFind the equation of the straight line through (2, 3) and perpendicular to the line 4x−3y=10
View SolutionLine t is shown in the xy-plane below
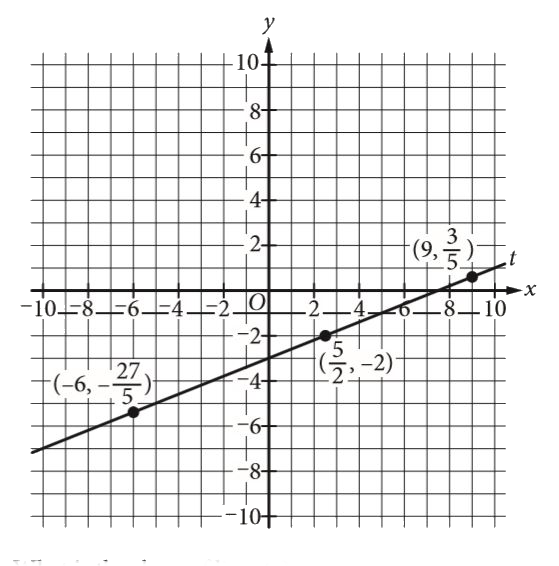
What is the slope of line t ?
View Solution