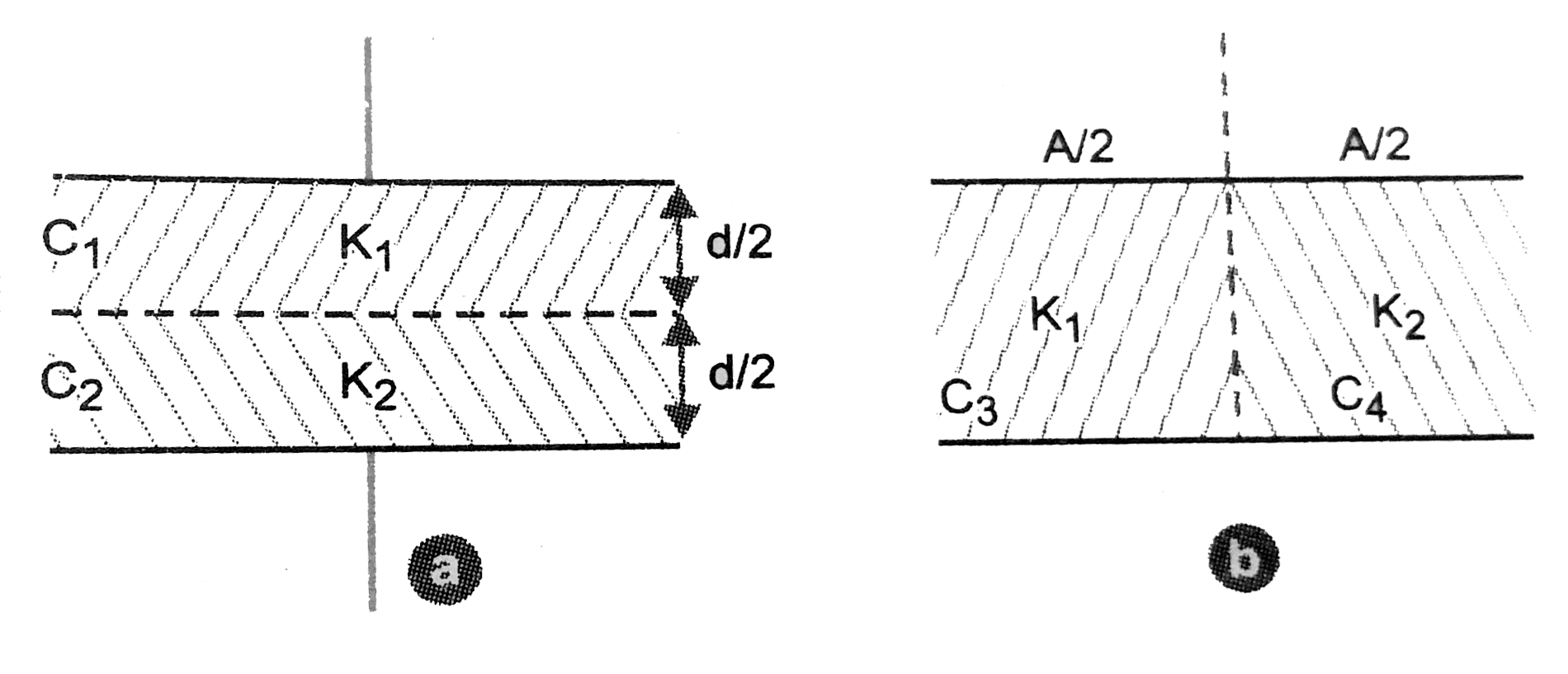
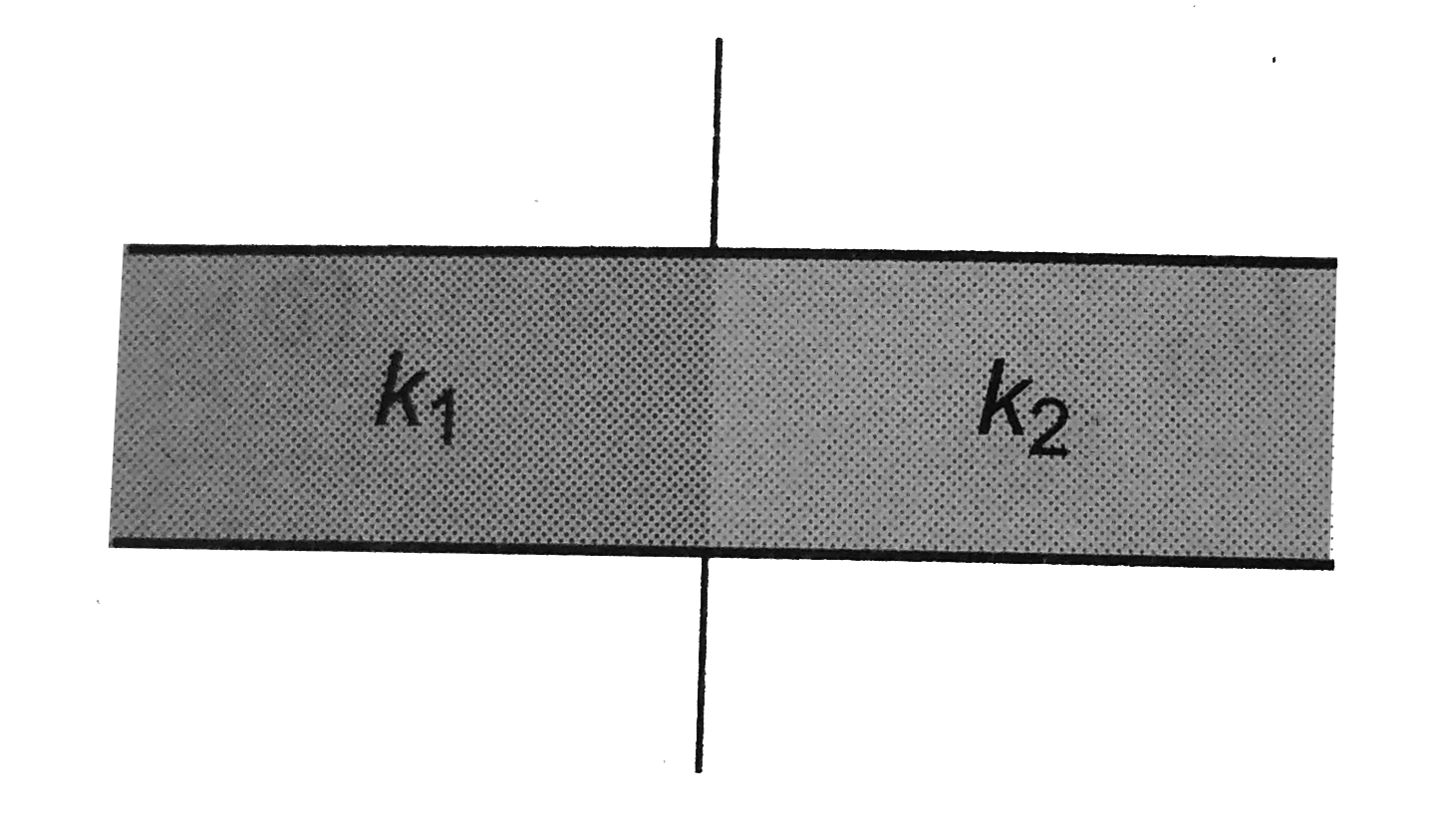
Calculate the ratio of capacitance of two capacitors of same dimension of same dimensions but of different values K and K4 arranged in two ways as shown in Fig. (i) and (ii).
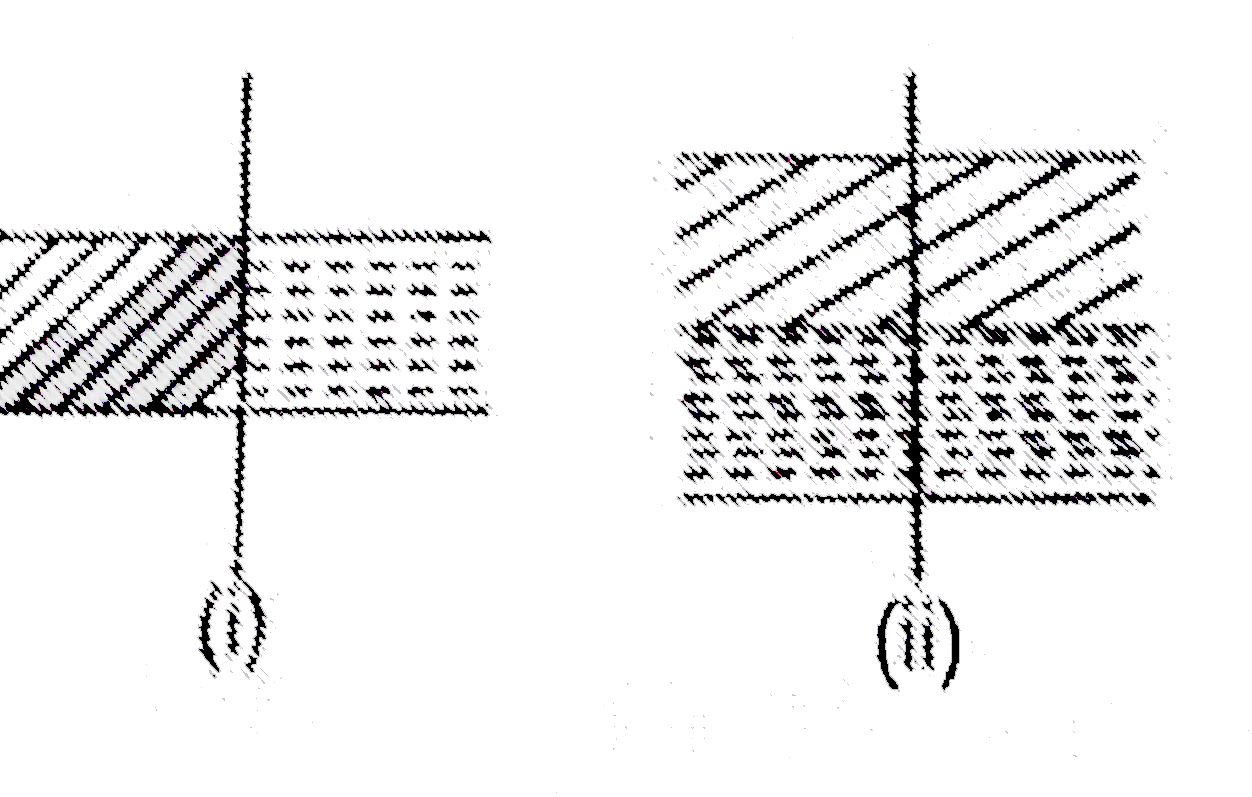
A
5:2
B
25:16
C
5:4
D
2:5
Video Solution
Text Solution
The correct Answer is:B
Here, C1=ε0K1A2d+ε0K2A2d
=ε0KA2d+ε0KA8d (Given,K1=K),(andK2=K4)
=ε0KAd(12+18)=ε0KAd(4+18)
⇒C1=5ε0KA8d .....(i)
and C2=ε0Ad2K1+d2K2=ε0Ad2K+d2K4=(2K)ε0A5d ......(ii)
Diving Eq. (i) by Eq. (ii), we get
C1C2=5ε0KA8d2Kε0A5d=5×52×8=2516
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
Which of the two have same dimensions
AForce and strainBForce and stressCAngular velocity and frequencyDEnergy and strainWhich of the two have same dimensions ?
AForce and strainBForce and stressCAngular velocity and frequencyDEnergy and strainThe capacitance of a capacitor, filled with two dielectrics of same dimensions but of dielectric constants K1andK2 respectively as shown will be -
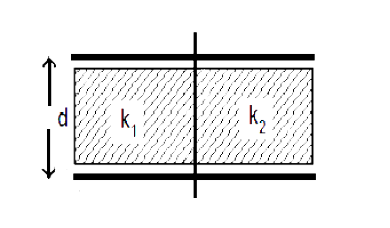 A∈0A2d(K1+K2)B∈0Ad(K1+K2)C∈0A2d(K1+K2K1+K2)D∈0Ad(K1K2K1+K2)
A∈0A2d(K1+K2)B∈0Ad(K1+K2)C∈0A2d(K1+K2K1+K2)D∈0Ad(K1K2K1+K2)
Which of the two have the same dimensions ?
View SolutionTwo physical quantities whose dimensions are not same, cannot be :
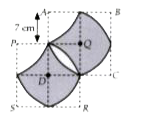
View SolutionABCD and PQRS are two squares of same dimensions. Find the area of shaded part.
View SolutionA parallel plate capacitor with air as medium between the plates has a capacitance of 10μF. The area of capacitor is divided into two equal halves and filled with two media as shown in the figure having dielectric constant k1=2 and k2=4. the capacitance of the system will now be
View SolutionGiven that C denotes capacitance of a capacitor and V is the potential difference across its plates. Then the dimensions of CV2 are same as that of
View Solution