One junction of a certain thermoelectric couple is at a fixed temperature r T and the other junction is at temperature T . The thermo electromotive force for this is expressed by E=K(T−Tr)[T0−12(T+Tr)]. At temperature T=12T0, the thermoelectric power is
12kT0
kT0
12kT20
12k(T0−Tr)2
a) Thermoelectric power,
S=dEdT=ddT[kT−Tr.{T0−12(T+Tr}]
Specific latent heat of vaporisatioin
=(22.68×105J/kg= X×10−3×22.68×105 ltrbgt =Y×10−3×3.36×105+Y×10−3×4200×100)
∴ XY=7.5622.68=13
S=k(T−Tr×(−12)+[T0−12(T02+Tr)}×k
When T=T02
S=−k2(T02−Tr)+{T0−12(T02+Tr)}×k
=−kT04+kTr2+kT0−kT04−kTr2=12kT0
Topper's Solved these Questions
- HEAT, TEMPERATURE AND CALORIMETRYBOOK - BITSAT GUIDECHAPTER - HEAT, TEMPERATURE AND CALORIMETRYEXERCISE - Bitsat archives
9 Videos
- GRAVITATIONBOOK - BITSAT GUIDECHAPTER - GRAVITATIONEXERCISE - BITSAT Archives
15 Videos
- LAWS OF THERMODYNAMICSBOOK - BITSAT GUIDECHAPTER - LAWS OF THERMODYNAMICSEXERCISE - 40
1 Videos
Similar Questions
Knowledge Check
One junction of a certain thermoelectric couple is at a fixed temperature Tr and the other junction is at temperature T. The thermo-electromotive force for this is expressed by E=k(T−Tr)[T0−(12)(T+Tr)]. At temperature T=12T0, the thermoelectric power is
A12kT0BkT0C12kT20D12k(T0−Yr)2One junction of a certain thermoelectric couple is at a fixed temperature Tr and the other junction is at temperature T. The thermo-electromotive force for this is expressed by E=k(T−Tr)[T0−(12)(T+Tr)]. At temperature T=12T0, the thermoelectric power is
A12kT0BkT0C12kT20D12k(T0−Yr)2If for a thermocouple Tn) is the neutral temperature, Tc is the tempreture of the cold junction and Ti) is the temperature of inversion, then
ATi=2Tn−TcBTi=Tn−2TcCTi=Tn−TcDNone of these
The thermo emf E of a thermocouple is found to vary wit temperature T of the junction (cold junction is 0∘C) as
E=40T−T220
The temperature of inversion for the thermocouple is
View SolutionIf the cold junction of thermocouple is kept at 0∘C and the hot junction is kept at T∘C, then the relation between neutral temperature (Tn) and temperature of inversion (Ti) is
View SolutionIf the cold junction of thermocouple is kept at 0∘C and the hot junction is kept at T∘C, then the relation between neutral temperature (Tn) and temperature of inversion (Ti) is
View SolutionThe temperature of the cold junction of the thermocouple is ^(∘)C and the temperature of the hot junction is T∘C. The relation for the thermo emf is given by, E=AT=12BT2 ltbRgt (when A=16 and B=0.08). The temperature of inversion will be
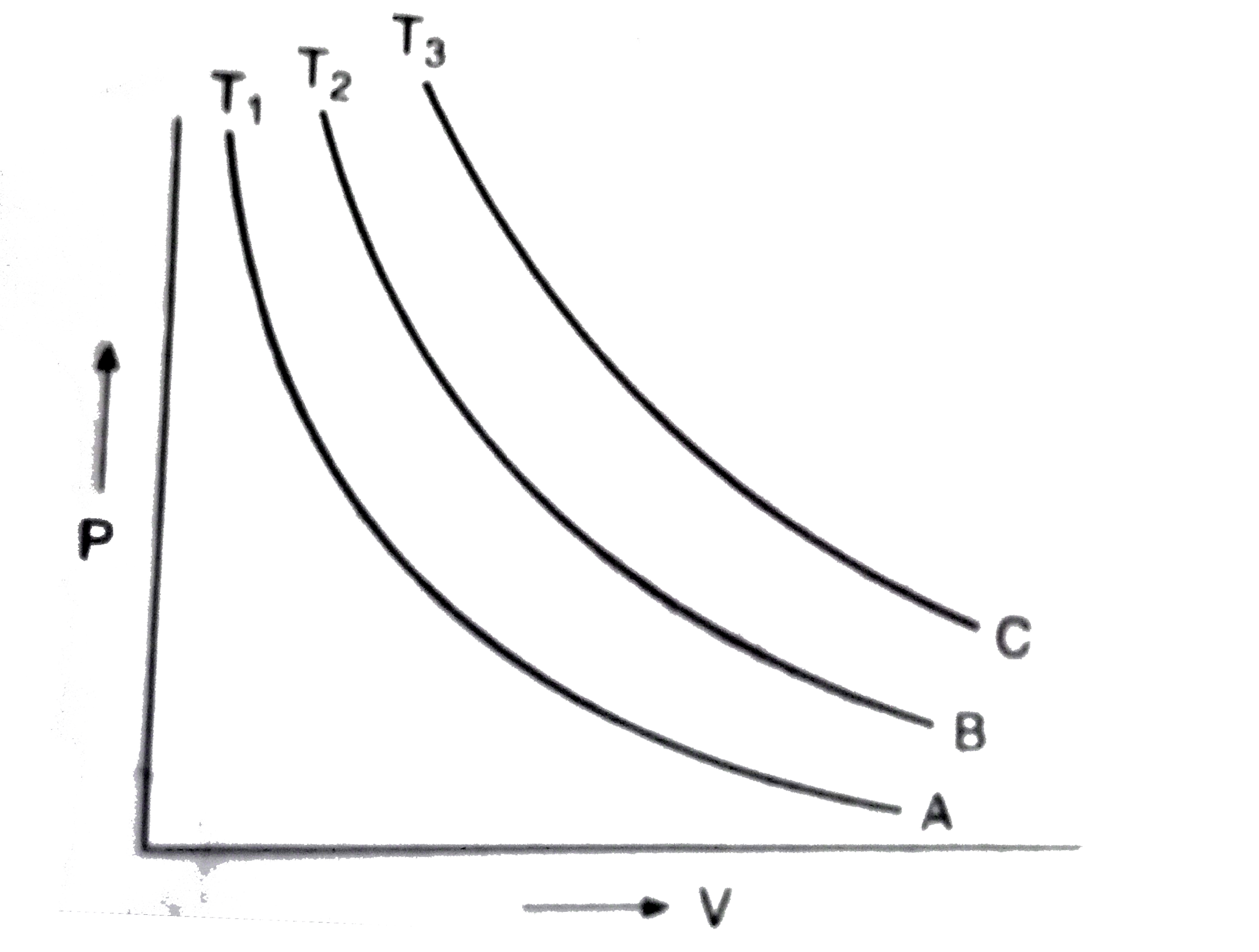
View SolutionIn following isothermal graphs A, B and C at temperatures T1,T2 and T3, the correct order of temperatures will be :
View Solution