6Ω and 12Ω resistors are connected in parallel. This combination is connected in series with 10 V battery and 6Ω resistor. What is the potential differnce between the terminals of the 12Ω resistor ?
4 V
16 V
2
8 V
The correct Answer is:A
To solve the problem step by step, let's break it down:
Step 1: Identify the Circuit Configuration
We have a 6Ω resistor and a 12Ω resistor connected in parallel. This combination is then connected in series with a 6Ω resistor and a 10V battery.
Step 2: Calculate the Equivalent Resistance of the Parallel Combination
The formula for the equivalent resistance \( R_{eq} \) of two resistors \( R_1 \) and \( R_2 \) in parallel is given by:
\(
\frac{1}{R_{eq}} = \frac{1}{R_1} + \frac{1}{R_2}
\)
Substituting \( R_1 = 6Ω \) and \( R_2 = 12Ω \):
\(
\frac{1}{R_{eq}} = \frac{1}{6} + \frac{1}{12}
\)
Finding a common denominator (which is 12):
\(
\frac{1}{R_{eq}} = \frac{2}{12} + \frac{1}{12} = \frac{3}{12} = \frac{1}{4}
\)
Thus,
\(
R_{eq} = 4Ω
\)
Step 3: Total Resistance in the Circuit
Now, we have the equivalent resistance of the parallel combination \( R_{eq} = 4Ω \) in series with the internal resistance of the battery \( R_{internal} = 6Ω \). The total resistance \( R_{total} \) is:
\(
R_{total} = R_{eq} + R_{internal} = 4Ω + 6Ω = 10Ω
\)
Step 4: Calculate the Current in the Circuit
Using Ohm's law, the current \( I \) flowing through the circuit can be calculated as:
\(
I = \frac{V}{R_{total}} = \frac{10V}{10Ω} = 1A
\)
Step 5: Determine the Voltage Across the Parallel Resistors
Since the 6Ω and 12Ω resistors are in parallel, they share the same voltage. The voltage across the parallel combination \( V_{parallel} \) can be calculated using Ohm's law again:
\(
V_{parallel} = I \times R_{eq} = 1A \times 4Ω = 4V
\)
Step 6: Calculate the Current Through the 12Ω Resistor
In a parallel circuit, the current divides inversely proportional to the resistance. The total current \( I \) is 1A, and we can find the current through the 12Ω resistor \( I_{12} \):
Using the current division rule:
\(
I_{12} = I \times \frac{R_{other}}{R_{1} + R_{2}} = 1A \times \frac{6Ω}{6Ω + 12Ω} = 1A \times \frac{6}{18} = \frac{1}{3}A
\)
Step 7: Calculate the Voltage Across the 12Ω Resistor
Now, we can find the potential difference \( V_{12} \) across the 12Ω resistor using Ohm's law:
\(
V_{12} = I_{12} \times R_{12} = \frac{1}{3}A \times 12Ω = 4V
\)
Conclusion
The potential difference between the terminals of the 12Ω resistor is 4 volts.
---
We have a 6Ω resistor and a 12Ω resistor connected in parallel. This combination is then connected in series with a 6Ω resistor and a 10V battery.
The formula for the equivalent resistance \( R_{eq} \) of two resistors \( R_1 \) and \( R_2 \) in parallel is given by:
\frac{1}{R_{eq}} = \frac{1}{R_1} + \frac{1}{R_2}
\)
\frac{1}{R_{eq}} = \frac{1}{6} + \frac{1}{12}
\)
\frac{1}{R_{eq}} = \frac{2}{12} + \frac{1}{12} = \frac{3}{12} = \frac{1}{4}
\)
R_{eq} = 4Ω
\)
Now, we have the equivalent resistance of the parallel combination \( R_{eq} = 4Ω \) in series with the internal resistance of the battery \( R_{internal} = 6Ω \). The total resistance \( R_{total} \) is:
R_{total} = R_{eq} + R_{internal} = 4Ω + 6Ω = 10Ω
\)
Using Ohm's law, the current \( I \) flowing through the circuit can be calculated as:
I = \frac{V}{R_{total}} = \frac{10V}{10Ω} = 1A
\)
Since the 6Ω and 12Ω resistors are in parallel, they share the same voltage. The voltage across the parallel combination \( V_{parallel} \) can be calculated using Ohm's law again:
V_{parallel} = I \times R_{eq} = 1A \times 4Ω = 4V
\)
In a parallel circuit, the current divides inversely proportional to the resistance. The total current \( I \) is 1A, and we can find the current through the 12Ω resistor \( I_{12} \):
I_{12} = I \times \frac{R_{other}}{R_{1} + R_{2}} = 1A \times \frac{6Ω}{6Ω + 12Ω} = 1A \times \frac{6}{18} = \frac{1}{3}A
\)
Now, we can find the potential difference \( V_{12} \) across the 12Ω resistor using Ohm's law:
V_{12} = I_{12} \times R_{12} = \frac{1}{3}A \times 12Ω = 4V
\)
The potential difference between the terminals of the 12Ω resistor is 4 volts.
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:DC PANDEYChapter:CURRENT ELECTRICITYExercise:Match the columns
Explore 4 Videos
- COMMUNICATION SYSTEMBook:DC PANDEYChapter:COMMUNICATION SYSTEMExercise:Subjective
Explore 11 Videos
- ELECTROMAGNETIC INDUCTIONBook:DC PANDEYChapter:ELECTROMAGNETIC INDUCTIONExercise:Medical entrances gallery
Explore 25 Videos
Similar Questions
Two resistors of 4Ω and 6Ω are connected in parallel. The combination is connected across a 6 volt battery of negligible resistance. Calculate (i) the power supplied by the battery, (ii) the power dissipated in each resistor.
View SolutionTwo resistors 4Ωand6Ω connected in parallel. The combination is connected across a 6V battery of negligible resistance. Calculate
(a) the current through the battery
(b) current through each resistor.
View Solution
Knowledge Check
A 3Ω resistor and a 6Ω resistor are connected in parallel and the combination is connected in series to a battery of 5 V and a 3Ω resistor. The potential difference across the 6Ω resistor
A2VB4VC3VD1V50Ω and 100Ω resistors are connected in series. This connection is connected with a battery of 24 volts. When a voltmeter of 100Ω resistance is connected across 100Ω resistor, then the reading of the voltmeter will be
A1.6VB1.0VC1.2VD2.0V50Ω and 100Ω resistors are connected in series. This connection is connected with a battery of 2.4 volts. Whne a voltmeter, then the reading of the voltmeter will be 100Ω resistor, then eh reading of the voltmeter will be
A1.6VB1.0VC1.2VD2.0V
Six 18.0Ω resistors are connected in parallel across a 12.0V ideal battery. What is the current through the battery?
View SolutionTwo resistors of resistances 2Ω and 6Ω are connected in parallel. This combination is then connected to a battery of emf 2 V and internal resistance 0.5Ω. What is the current flowing thrpough the battery ?
View SolutionTwo resistors 4Ω and 800Ω are connected in series with a 6V battery. The potential difference measured by voltmeter of 10kΩ across 400Ω resistor is
View SolutionA 5V battery with internal resistance 2Ω and a 2V battery with internal resistance1Ω are connected to a 10Ω resistor as shown in the figure.
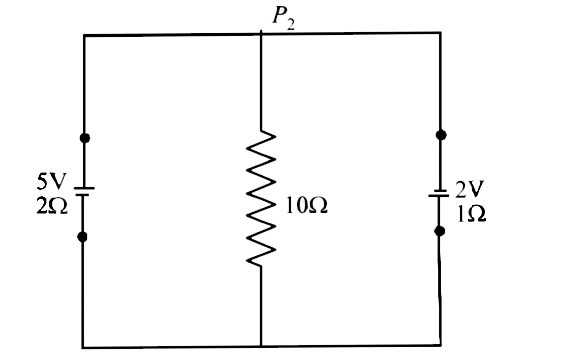
The current in the 10Ω resistor is
View SolutionThree resistors 2Ω,4Ωand5Ω are combined in parallel. This combination is connected to a battery of emf 20V and negligible internal Resistance. The total current drawn from the battery is
View Solution
