A conical pendulum of length L makes an angle θ with the vertical. The time period will be

2π√lcosθg
2π√Lgcosθ
2π√Ltanθγ
2π√Lgtanθ
The correct Answer is:A
Tcosθ=mg,Tsinθ=mRω2
or tanθ=Rω2g=R(2π/T)2g
T=2π√Rgtanθ
But R=Lsinθ
∴ T=2π√Lcosθg
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Match the columns
3 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
- CIRCULAR MOTIONBOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Check point
45 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
A charged particle having its charge to mass ratio as β goes in a conical pendulum oflength L making an angle θ with vertical and angular velocity ω. If a magnetic field B is directed vertically downwards (see figure-4.238):
View SolutionA simple pendulum of length l ils pulled aside to make an angle θ with the vertical. Find the magnitude of the torque of the weitht w of the bob about the point of suspension. When is the torque zero?
View Solution
Knowledge Check
A simple pendulum of length l is set in motion such that the bob, of mass m, moves along a horizontal circualar path , and the string makes a constant angle θ with the vertical. The time period of rotation of the bob is l and the tension in the thread is T
At=2π√l/gBt=2π√lcosθ/gCT=4π2mlt2DThe bob is in equilibriumA heavy small sized sphere is suspended by a string of length l . The sphere rotates uniformly in a horizontal circle with the string making an angle θ with the vertical. Then the time period of this conical pendulum is-
AT = 2πBT=2π√(lsinθg)CT=2π√(lcosθg)DT=2π√(lg)A heavy small sized sphere is suspended by a string of length 2. The sphere is rotated uniformly in a horizontal circle with the string making an angle θwith the vertical. The time period of this conical pendulum is
A2π√ltanθgB2π√lcosθgC2π√1gD2π√lcosθg
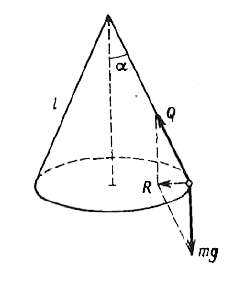
Find the period of revolution of a conical simple pendulum whose thread of length l makes an angle α with the vertical (Fig).
View SolutionIf the string of a conical pendulum makes an angle θ with horizontal, then square of its time period is proportional to
View SolutionA simple pendulum is constructed by attaching a bob of mas m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View SolutionWhen the string of a conical pendulum makes an angle of 45∘ with the vertical , its time is T1 when the string makes an angle of 60∘ with the vertical , its time period is T2 then T21/T22is
View SolutionA heavy & big sphere is hang with a string of length l. This sphere move in a horizontal circular path making an angle θ with vertical then its time period is
View Solution
