x2+y2+4x−2y=−1
The equation of a circle in the xy-plane is shown above. What is the radius of the circle?
2
3
4
9
To find the radius of the circle given by the equation x2+y2+4x−2y=−1, we need to convert this equation into the standard form of a circle's equation, which is (x−h)2+(y−k)2=r2, where (h,k) is the center of the circle and r is the radius.
1. Rearrange the Equation: Start with the given equation:
x2+y2+4x−2y=−1
Rearranging gives:
x2+4x+y2−2y=−1
2. Complete the Square for x:
- Take the x terms: x2+4x.
- To complete the square, take half of the coefficient of x (which is 4), square it, and add/subtract it:
x2+4x=(x+2)2−4
3. Complete the Square for y:
- Take the y terms: y2−2y.
- Similarly, take half of the coefficient of y (which is -2), square it, and add/subtract it:
y2−2y=(y−1)2−1
4. Substitute Back into the Equation: Now substitute the completed squares back into the equation:
(x+2)2−4+(y−1)2−1=−1
5. Simplify the Equation: Combine the constants on the left side:
(x+2)2+(y−1)2−5=−1
Add 5 to both sides:
(x+2)2+(y−1)2=4
6. Identify the Radius: Now, the equation is in the standard form:
(x−(−2))2+(y−1)2=22
Here, r2=4, so the radius r is:
r=2
Final Answer:
The radius of the circle is 2.
x2+y2+4x−2y=−1
Rearranging gives:
x2+4x+y2−2y=−1
- Take the x terms: x2+4x.
- To complete the square, take half of the coefficient of x (which is 4), square it, and add/subtract it:
x2+4x=(x+2)2−4
- Take the y terms: y2−2y.
- Similarly, take half of the coefficient of y (which is -2), square it, and add/subtract it:
y2−2y=(y−1)2−1
(x+2)2−4+(y−1)2−1=−1
(x+2)2+(y−1)2−5=−1
Add 5 to both sides:
(x+2)2+(y−1)2=4
(x−(−2))2+(y−1)2=22
Here, r2=4, so the radius r is:
r=2
The radius of the circle is 2.
Similar Questions
x2+6x+y2−8y=171
The equation of a circle in the xy-plane is shown above. What is the positive difference between the x-and y-coordinates of the center of the circle ?
View Solutionx2+20x+y2+16y=−20
The equation above defines a circle in the xy-plane. What are the coordinates of the centre of the circle ?
View Solution
Knowledge Check
x2+y2−6x+8y=−9
The equation of the circle in xy-plane is shown above. What is the radius of the circle?A3B4C9D16In the xy-plane , a circle with center (6,0) is tangent to the line y=x. what is the radius of the circle ?
A2√6B2√3C3√2D3x2+y2−6x+8y=56
What is the area of a circle whose equation is given above?A25πB81πC162πD6,561π
The equation of a circle in the xy-plane is x2+4x+y2−10y=20. If the line x=k intersects the circle in exactly one point, what is the possible value of k?
View SolutionIn the xy-plane, the graph of 2x2−6x+2y2=45 is a circle . What is the radius of the circle ?
View Solution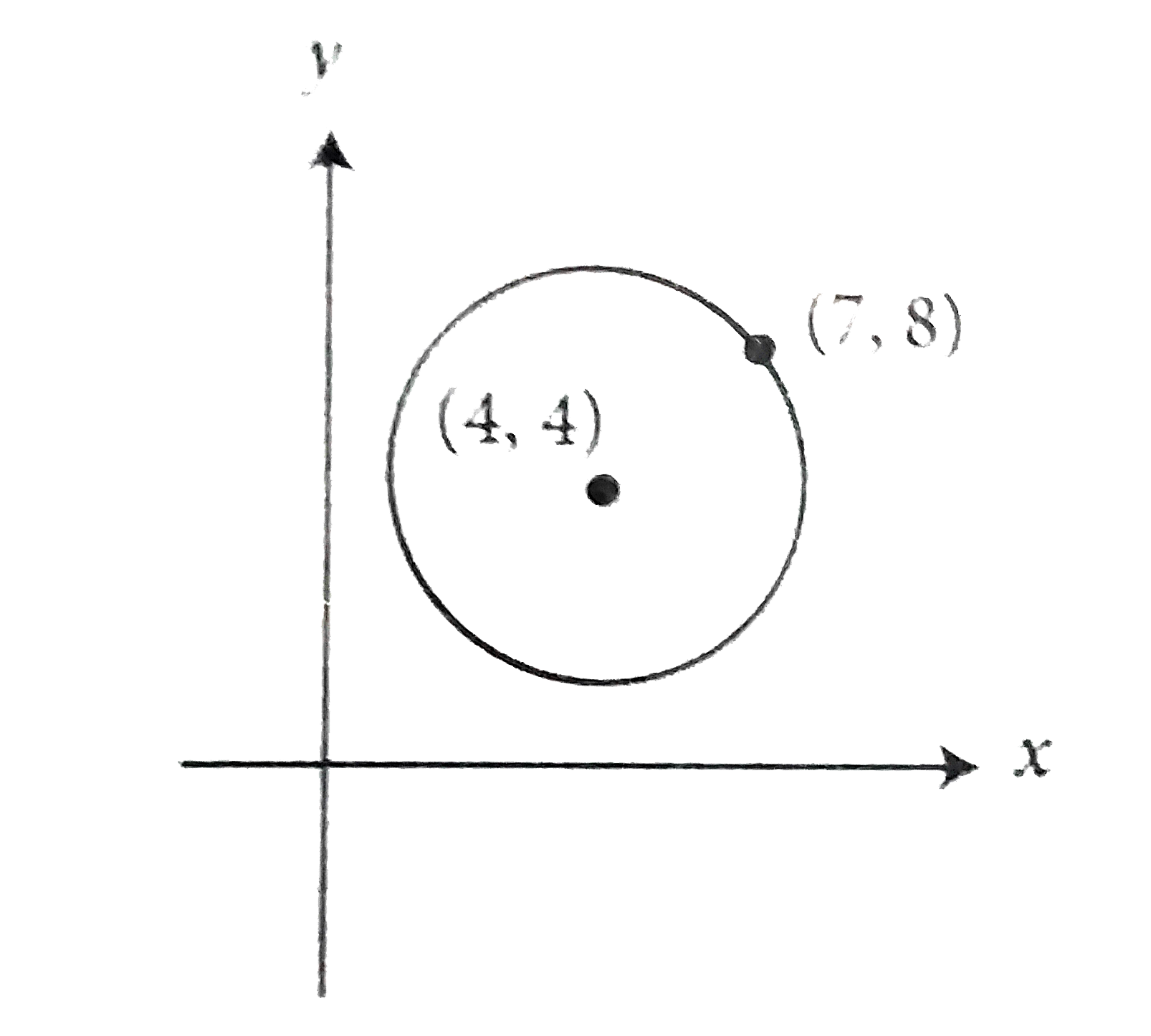
the circle defined by the equation (x−4)2+(y−4)2=25 has its centre at point (4,4) and includes point (7,8) on the circle. This is shown in the figure above. What is the area of the circle shown?
View Solutionx2+y2+8x−20y=28
What is the diameter of the circle given by the equation above ?
View Solution(x−2)2+(y+5)2=36
IF a circle in the xy-plane has the equation above. Which of the following does NOT lie on the exterior of the circle?
View Solution
