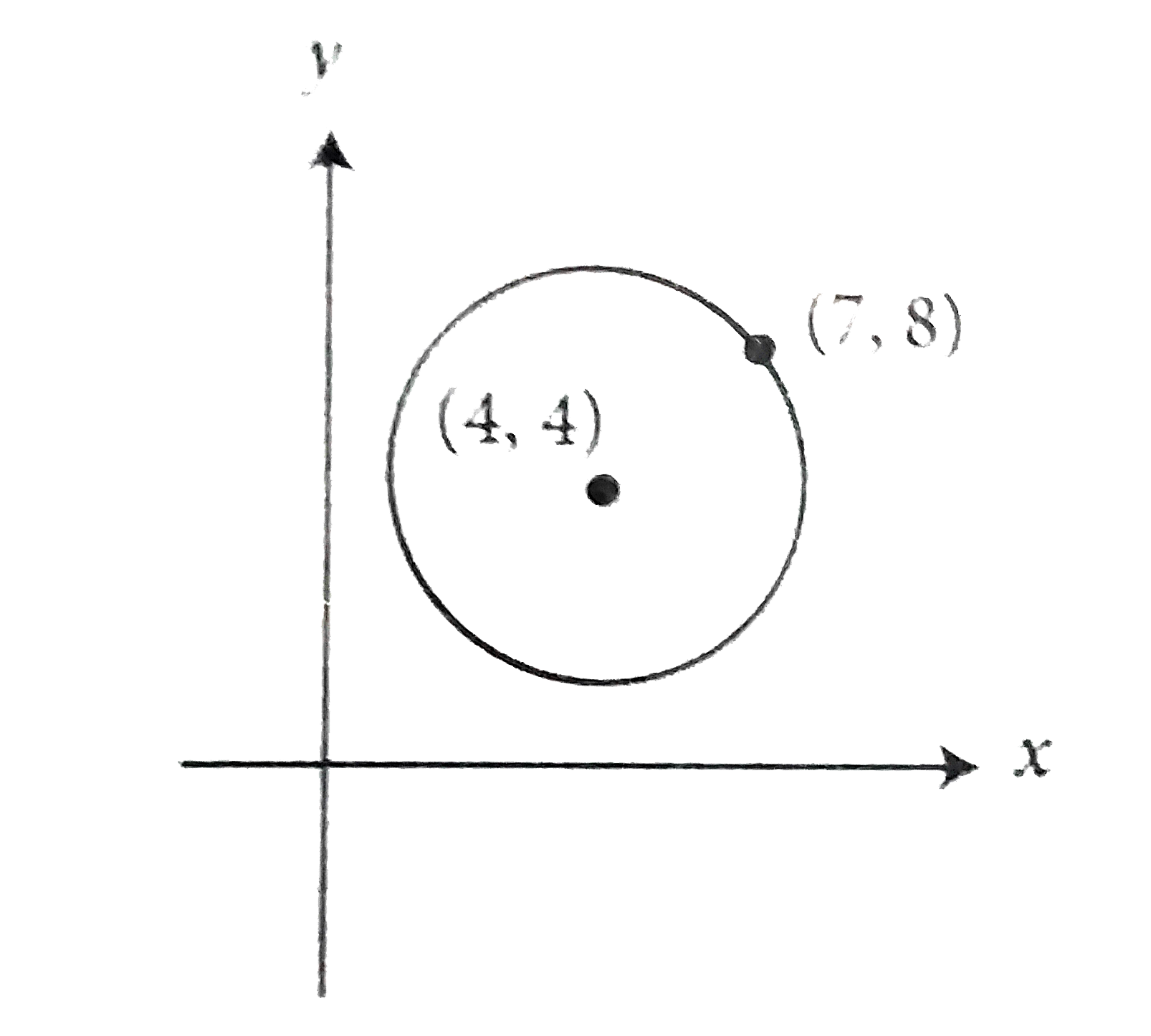
the circle defined by the equation ${(x - 4)}^{2} + {(y - 4)}^{2} = 25$ has its centre at point (4,4) and includes point (7,8) on the circle. This is shown in the figure above. What is the area of the circle shown?

$5 π$

$10 π$

$16 π$

$25 π$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

Answer

Step by step video, text & image solution for the circle defined by the equation (x-4)^2+(y-4)^2=25 has its centre at point (4,4) and includes point (7,8) on the circle. This is shown in the figure above. What is the area of the circle shown? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS GEOMETRY

Topper's Solved these Questions

Knowledge Check

$x^{2} + y^{2} - 6 x + 8 y = - 9$
The equation of the circle in xy-plane is shown above. What is the radius of the circle?
A $3$
B $4$
C $9$
D $16$
$x^{2} + y^{2} + 4 x - 2 y = - 1$
The equation of a circle in the xy-plane is shown above. What is the radius of the circle?
A2
B3
C4
D9
A circle in the xy-plane is defined by the equation ${(x - 4)}^{2} + {(y + 2)}^{2} = 100 .$ Which of the following points is located on the circumference of the circle ?
A $(- 3, 5)$
B $(0, 9)$
C $(4, - 2)$
D $(4, 8)$

PRINCETON-GEOMETRY-EXAMPLES

Home
Ask Doubt
Profile

the circle defined by the equation (x−4)2+(y−4)2=25 has its centre at point (4,4) and includes point (7,8) on the circle. This is shown in the figure above. What is the area of the circle shown?

The correct Answer is:D

Topper's Solved these Questions

Similar Questions

Knowledge Check

x2+y2−6x+8y=−9 The equation of the circle in xy-plane is shown above. What is the radius of the circle?

x2+y2+4x−2y=−1 The equation of a circle in the xy-plane is shown above. What is the radius of the circle?

A circle in the xy-plane is defined by the equation (x−4)2+(y+2)2=100. Which of the following points is located on the circumference of the circle ?

PRINCETON-GEOMETRY-EXAMPLES

the circle defined by the equation ${(x - 4)}^{2} + {(y - 4)}^{2} = 25$ has its centre at point (4,4) and includes point (7,8) on the circle. This is shown in the figure above. What is the area of the circle shown?

$x^{2} + y^{2} - 6 x + 8 y = - 9$
The equation of the circle in xy-plane is shown above. What is the radius of the circle?

$x^{2} + y^{2} + 4 x - 2 y = - 1$
The equation of a circle in the xy-plane is shown above. What is the radius of the circle?

A circle in the xy-plane is defined by the equation ${(x - 4)}^{2} + {(y + 2)}^{2} = 100 .$ Which of the following points is located on the circumference of the circle ?