Question

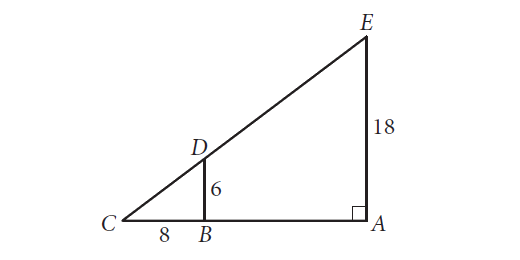
In the figure above, $\overline{BD}$ is parallel to $\overline{AE}$. What is the length of $\overline{CE}$ ?

Answer

C

Explanation

In the figure given , since `bar(BD)` is parallel to `bar(AE)` are both segments are intersected by `bar(CE)` , then angle BDC and angle BDC and angle AEC are corresponding angles and therefore congruent. Angle BCD and angle ACE are similar because if two angles of one triangle are congruent to two angles of another triangle , the triangles are similar . Since triangles BCD and triangle ACE are similar, their corresponding sides are proportional . So in triangle BCD and triangle ACE , `bar(BD)` corresponds to `bar(AE)` and `bar(CD)` corresponds to `bar(CE)` . Therefore `(BD)/(CD)=(AE)/(CE)` . Since triangle BCD is a right triangle , the Pythagorean theorem can be used to give the value of CD : `6^2+8^2=CD^2`. Taking the square root of each side gives CD=10. Substituting the values in the proportion `(BD)/(CD)=(AE)/(CE)` yields `6/10=18/(CE)` . Multiplying each side by CE, and then multiplying by `10/6` yields CE = 30. Therefore , the length of `bar(CE)` is 30.