If y=a+ bx^(2), where a, b are constants, then

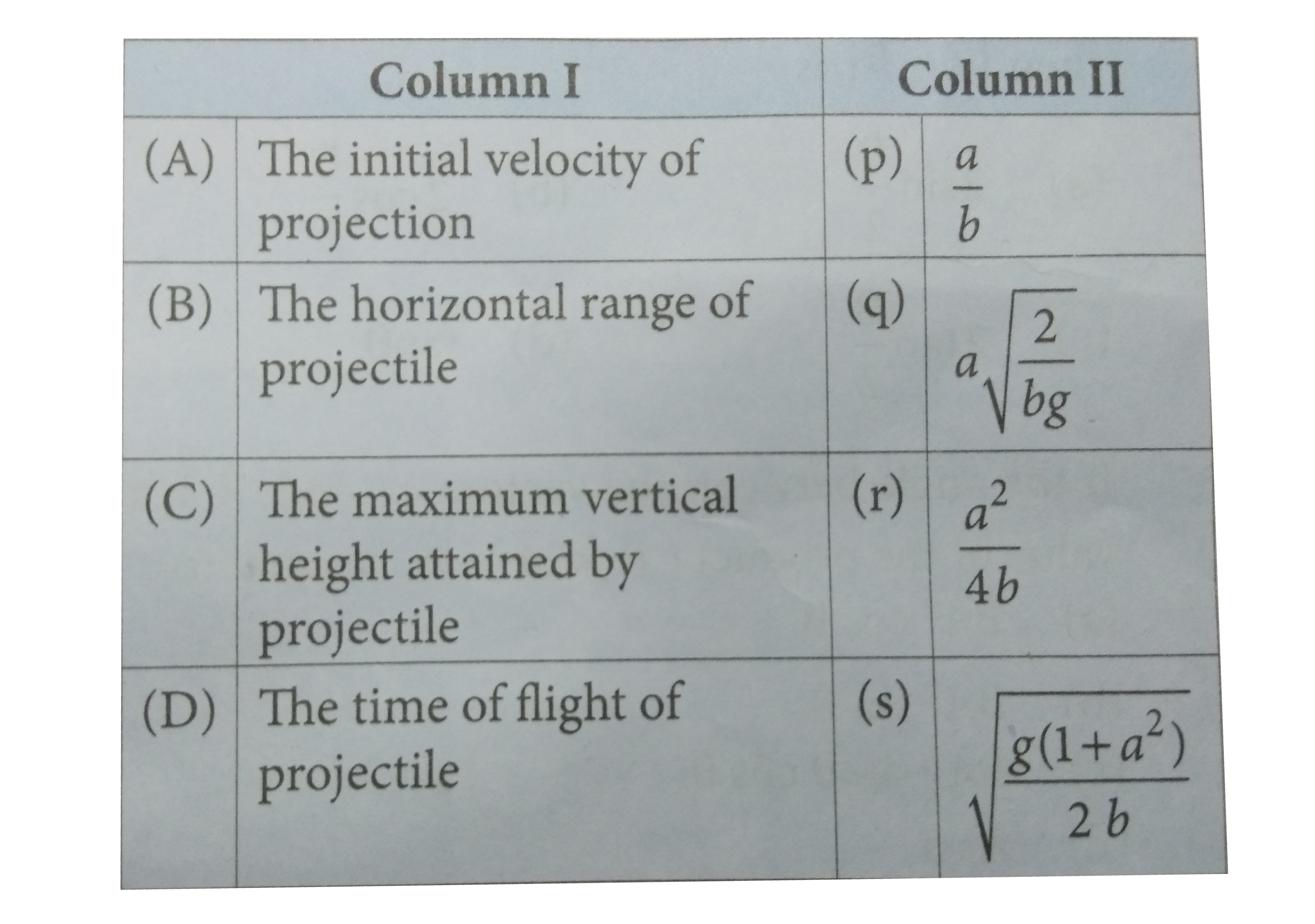
The trajectory of a projectile in a vertical plane is $y = a x - b x^{2}$ , where a and b are constants and x and y are respectively horizontal and vertical distances of the projectile from the point of projection. The maximum height attained by the particle and the angle of projection form the horizontal are:
A $\frac{b^{2}}{2 a}, {tan}^{- 1} (b)$
B $\frac{a^{2}}{b}, {tan}^{- 1} (2 b)$
C $\frac{a^{2}}{4 b}, {tan}^{- 1} (a)$
D $\frac{2 a^{2}}{b}, {tan}^{- 1} (a)$
A particle moves in the $x y$ plane with a constant acceleration 'g' in the negative-direction. Its equqaiton of motion is $y = a x - b x^{2}$ , where $a$ and $b$ are constants. Which of the following are correct?
AThe x-compound of its velocity is constant.
BAt the origin, the y-compound of its velocity is a $\sqrt{\frac{g}{2 b}}$ .
CAt the origin, its velocity makes an angle ${tan}^{- 1}$ (a) with yeh x-axis.
DThe particle moves exactly like a projectile.
The trajectory of a projectile in a vertical plane is $y = a x - b x^{2}$ , where $a and b$ are constant and $x and y$ are, respectively, horizontal and vertical distances of the projectile from the point of projection. The maximum height attained by the particle and the angle of projectile from the horizontal are.
A $\frac{b^{2}}{2 a}, {tan}^{- 1} (b)$
B $\frac{a^{2}}{b}, {tan}^{- 1} (2 b)$
C $\frac{a^{2}}{4 b}, {tan}^{- 1} (a)$
D $\frac{2 a^{2}}{b}, {tan}^{- 1} (a)$

If $y = a + b x^{2}$ , where a, b are constants, then