A solid sphere of radius r is floating at the interface of two immiscible liquids of densities ρ1 and ρ2(ρ2>ρ1), half of its volume lying in each. The height of the upper liquid column from the interface of the two liquids is h. The force exerted on the sphere by the upper liquid is (atmospheric pressure =p0 and acceleration due to gravity is g):
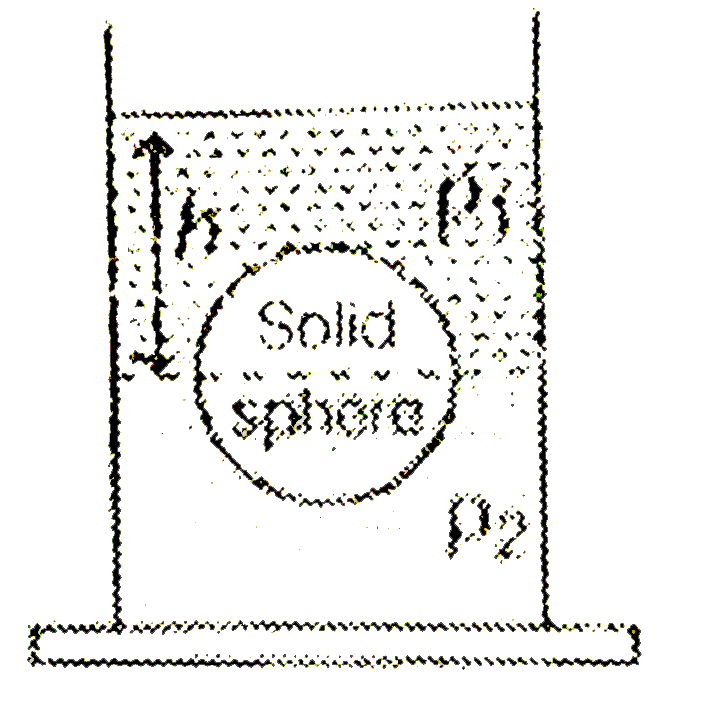
p0πr2+(h−23r)πr2ρ1g
(h−23r)πr2ρ1g
23πr2ρ1g
p0×πr2
The correct Answer is:A
Step by step video, text & image solution for A solid sphere of radius r is floating at the interface of two immiscible liquids of densities rho_(1) and rho_(2)(rho_(2) gt rho_(1)), half of its volume lying in each. The height of the upper liquid column from the interface of the two liquids is h. The force exerted on the sphere by the upper liquid is (atmospheric pressure = p_(0) and acceleration due to gravity is g): by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct21videos
PROPERTIES OF MATTER
- BOOK - DC PANDEYCHAPTER - PROPERTIES OF MATTEREXERCISE - Comprehension32videos
PROPERTIES OF MATTER
- BOOK - DC PANDEYCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer8videos
PROPERTIES OF MATTER
- BOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective10videos
PROJECTILE MOTION
- BOOK - DC PANDEYCHAPTER - RAY OPTICSEXERCISE - Integer type q.15videos
RAY OPTICS
Similar Questions
A uniform sphere of density ρ floats at the interface of two liquids of densities ρ1 and ρ2(>ρ1) The sphere has 23 rd of vertical diameter in denser liquid and remaining part in the other liquid.If aρ=bρ1+cρ2 then b+c-a=_______.
View Solution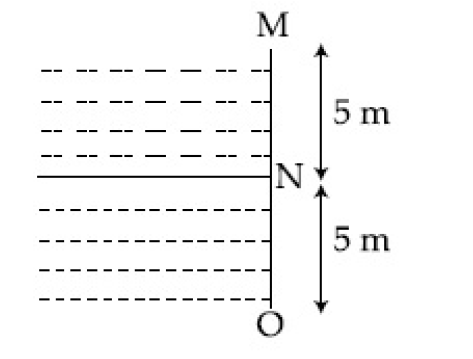 Tow liquids of desities ρ1 and ρ2(ρ2=2ρ1) are filled up behind a square wall of side 10 m as shown in the figure. Each liquids has a height of 5m. The ratio of the forces due to these liqyuids exeted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing):View Solution
Tow liquids of desities ρ1 and ρ2(ρ2=2ρ1) are filled up behind a square wall of side 10 m as shown in the figure. Each liquids has a height of 5m. The ratio of the forces due to these liqyuids exeted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing):View Solution
Knowledge Check
- Question 1 - Select One
A solid sphere having volume V and density ρ floats at the interface of two immiscible liquids of densityes ρ1 and ρ2 respectively. If ρ1<ρ<ρ2, then the ratio of volume of the parts of the sphere in upper and lower liquid is
A(rho-rho_(1))/(rho_(2)-rho)Bρ2−ρρ−ρ1C(rho+rho_(1))/(rho+rho_(2))D(rho+rho_(2))/(rho+rho_(1)) - Question 2 - Select One
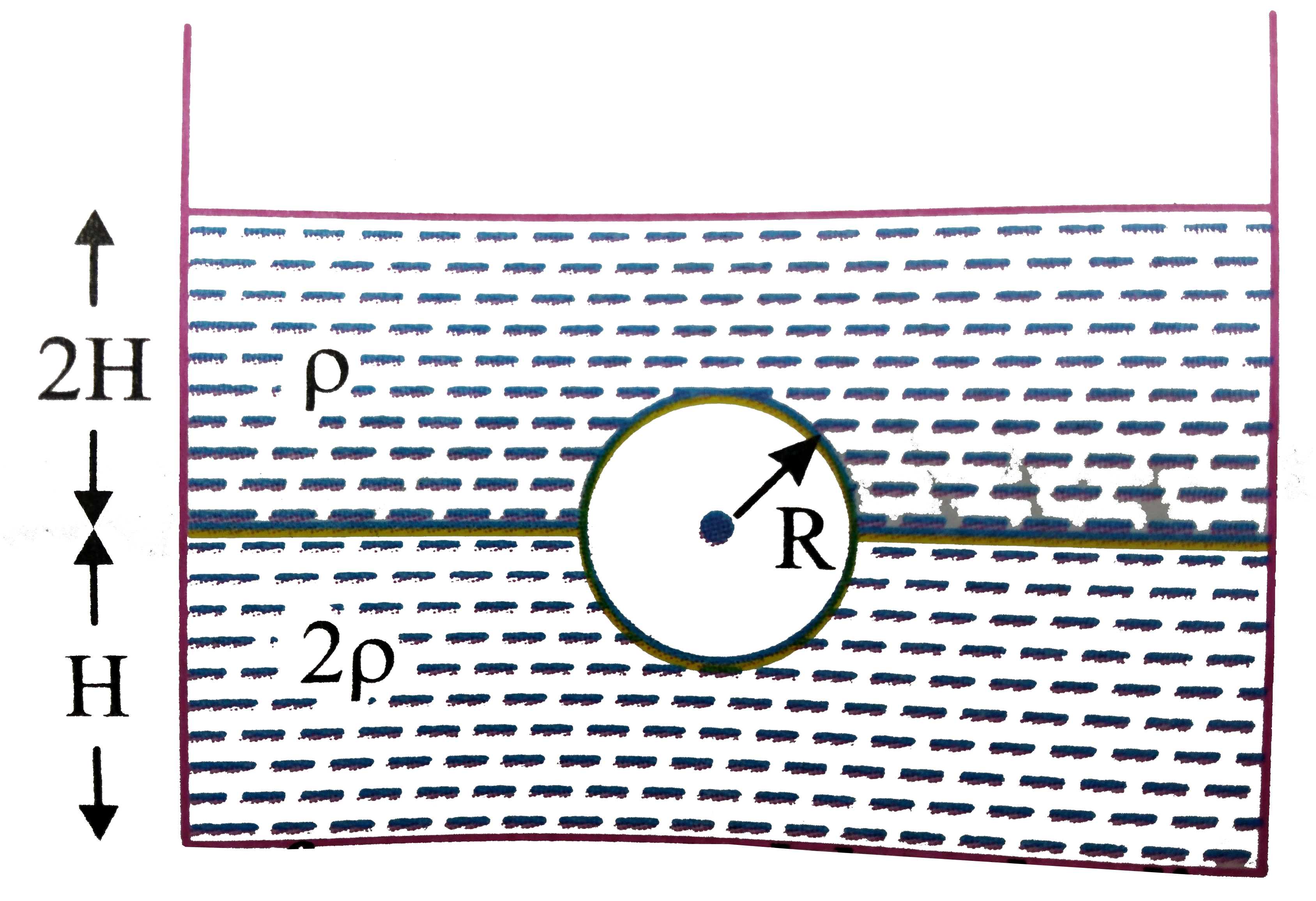
A spherical ball of radius R is floating at the interface of two liquids with densities ρ and 2ρ. The volumes of the ball immersed in two liquids are equal. Answer the following questions:
Find the force exerted by the liquid with density 2ρ on the ballAπR2ρg(H+2R3)B23πR2ρgC43πR2ρgD2πR2ρg(H+2R3) - Question 3 - Select One
The absolute pressure at a depth h below the surface of a liquid of density ρ is [Given Pa = atmospheric pressure, g = acceleration due to gravity]
AρghBPa+ρghCPa−ρghDPa2ρgh
A vertical pole of length l , density ρ , area of cross section A floats in two immiscible liquids of densities ρ1and ρ2. In equuilibrium possition the bottom end is at the interface of the liquids. When the cylinder is displaced vertically, find the time period of oscillation.
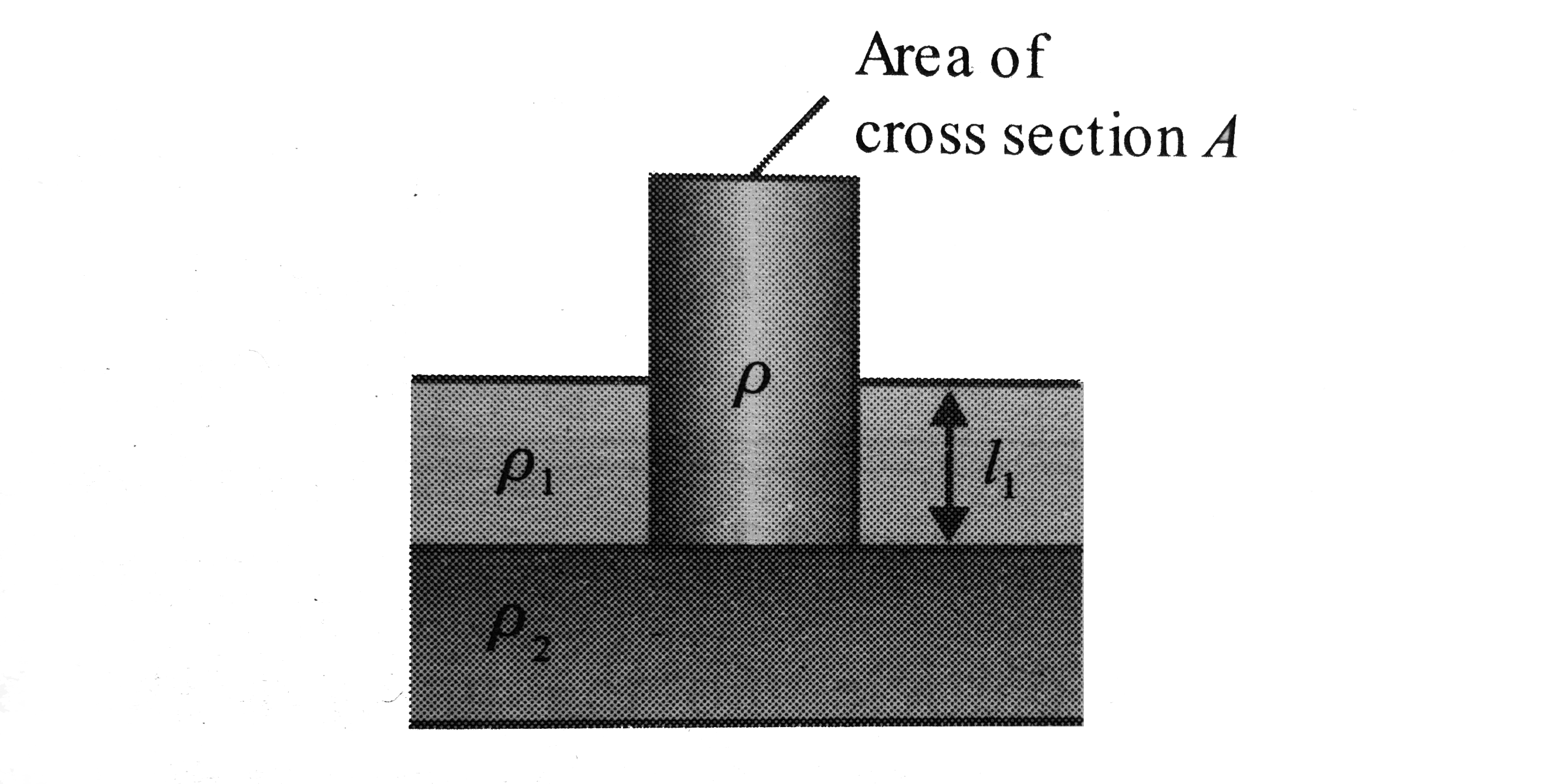 View Solution
View SolutionA thin uniform tube is bent into a circle of radius r in the vertical plane. Equal volumes of two immiscible liquids, whose densities are ρ1 and ρ2(ρ1>ρ2) fill half the circle. The angle θ between the radius vector passing though the common interface and the vertical is :
View Solution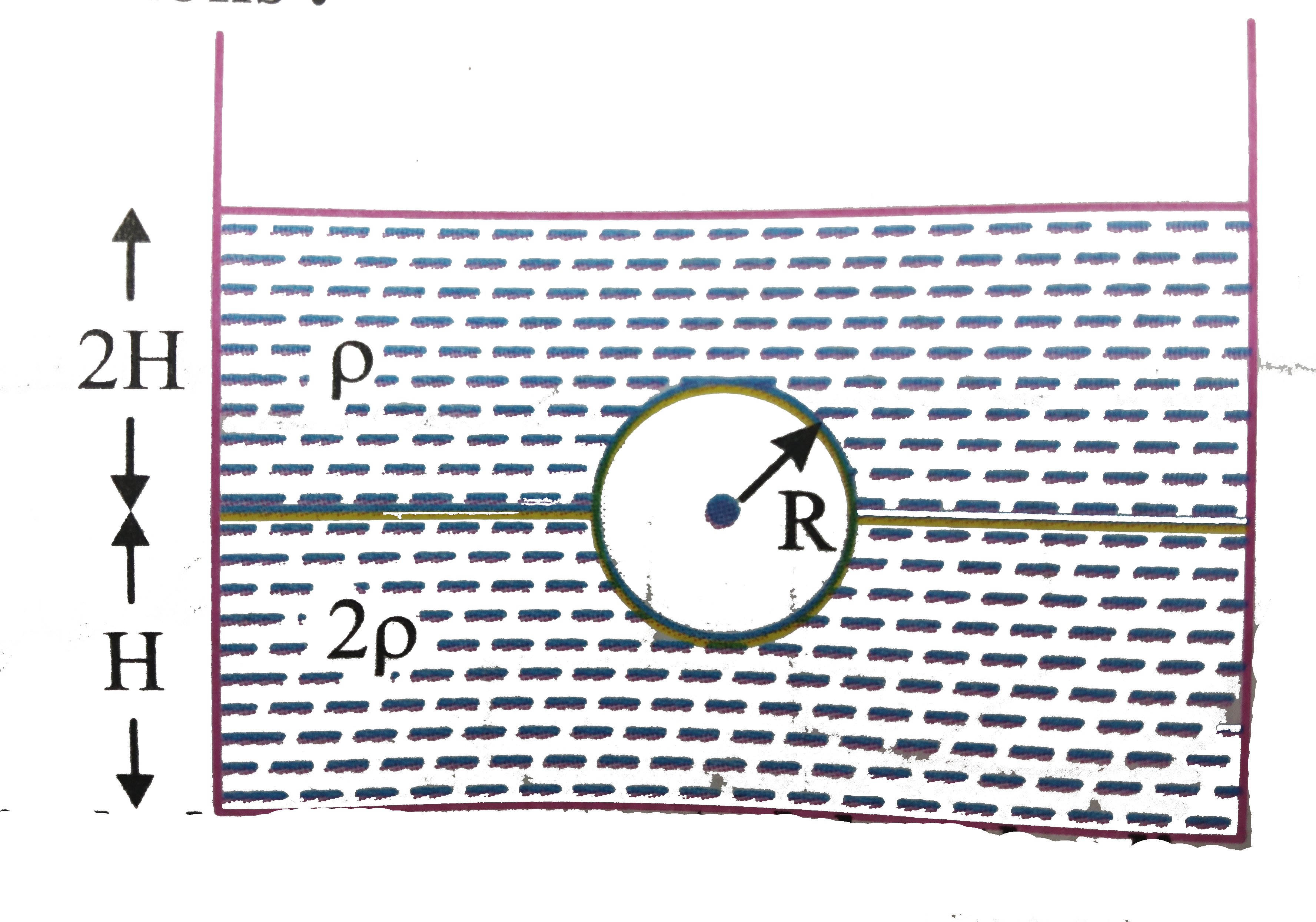
A spherical ball of radius R is floating at the interface of two liquids with densities ρ and 2ρ. The volumes of the ball immersed in two liquids are equal. Answer the following questions:
If a hole is drilled at the bottom of the vessel then volume of the ball immersed inliquid with density ρ willView Solution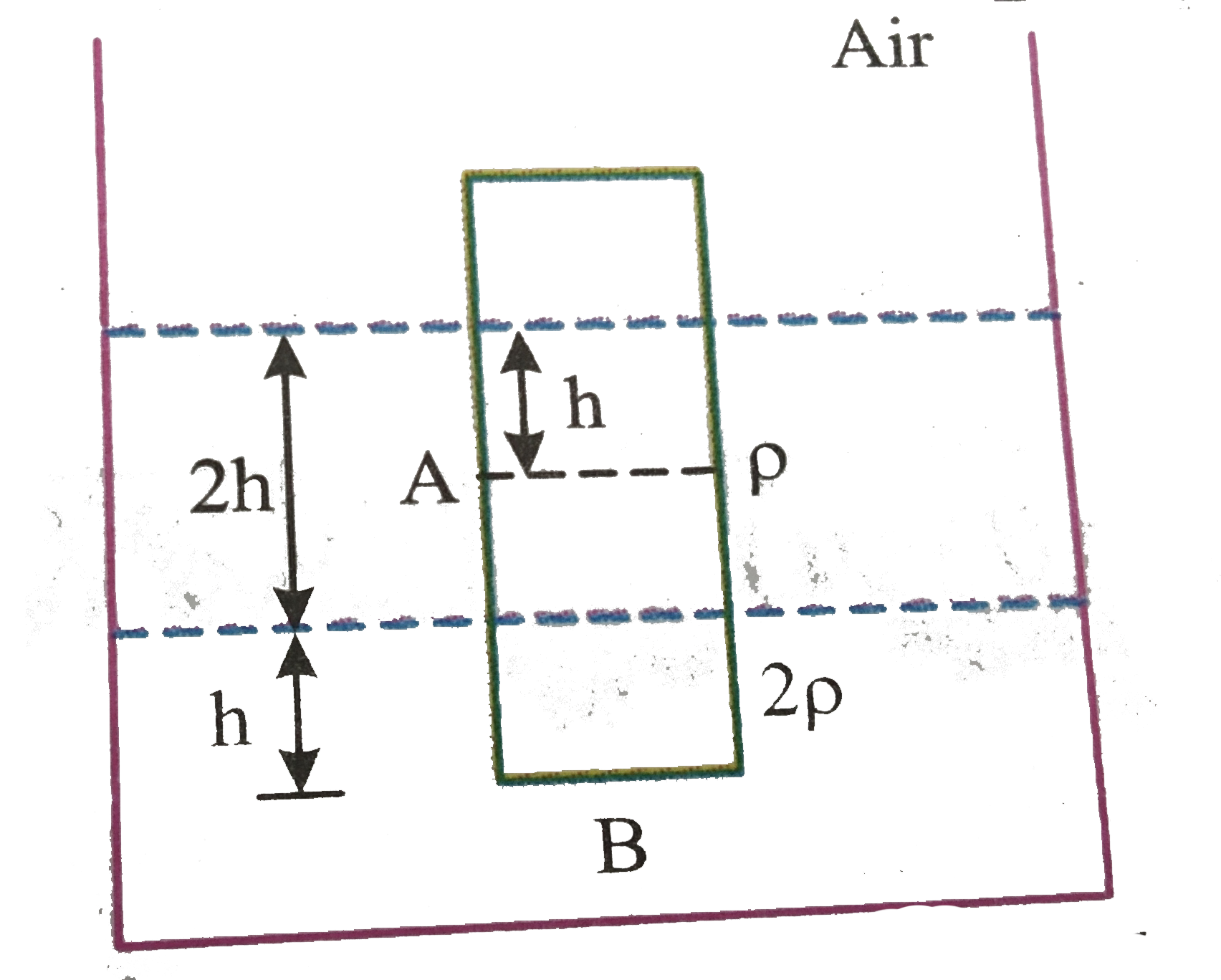
A cylinder stands vertical in two immiscible liquids of densities ρ and 2ρ as shown. Find the difference in pressure at point A and B:View SolutionA Solid uniform ball of volume V floats on the interface of two immiscible liquids . [The specific gravity of the upper liquid is γ/2 and that of the lower liquid is 2γ, where γ is the specific gravity of the solid ball.] The fraction of the volume of the ball that will be in upper liquid is
View Solution

