A particle of mass m moves around the origin in a potential 12mω2r2, where r is the distance from the origin. Applying the Bohr model in this case, the radius of the particle in its nth orbit in terms of a=√h/2πmω is
a√n
an
an2
an√n
The correct Answer is:A
Step by step video, text & image solution for A particle of mass m moves around the origin in a potential (1)/(2)momega^(2)r^(2), where r is the distance from the origin. Applying the Bohr model in this case, the radius of the particle in its n^(th) orbit in terms of a=sqrt(h//2pimomega) is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
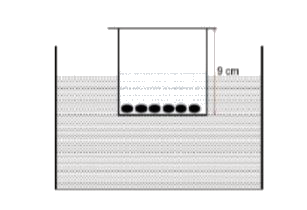
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
View SolutionA small particle of mass m move in such a way the potential energy U=12m2ω2r2 when a is a constant and r is the distance of the particle from the origin Assuming Bohr's model of quantization of angular momentum and circular orbits , show that radius of the nth allowed orbit is proportional to in
View SolutionA small particle of mass m moves in such a way that the potential energy U=12mω2r2, where ω is a constant and r is the distance of the particle from the origin. Assuming Bohr's model of quantisation of angular momentum and circular orbits. Find the radius of the nth orbit.
View SolutionA small particle of mass m moves in such a way that the potential energy U=ar^(2) where a is a constant and r is the distance of the particle from the origin. Assuming Bohr's model of quantization of angular momentum and circular orbits, find the radius of n^(th) allowed orbit.
View Solutionsuppose an electron is attracted towards the origin by a force k/r, where k is a constant and r is the distance of the electron form the origin. By applying bohr model to this system, the radius of nth orbit of the electron is found to be rn and
View SolutionA particle of mass m moves in circular orbits with potential energy N(r)=Fr, wjere F is a positive constant and r its distance from the origin. Its energies are calculated using the Bohr model. If the radius of the the nth orbit (here h is the Planck's constant)
View SolutionA small particle of mass m move in such a way the potential energy (U=12m2ω2r2) when a is a constant and r is the distance of the particle from the origin Assuming Bohr's model of quantization of angular momentum and circular orbits , show that radius of the nth allowed orbit is proportional to in
View SolutionA small particle of mass m move in such a way the potential energy U=12m2ω2r2 when a is a constant and r is the distance of the particle from the origin Assuming Bohr's model of quantization of angular momentum and circular orbits , show that radius of the nth allowed orbit is proportional to in
View SolutionA small piece of mass m moves in such a way the P.E. = -(1)/(2)mkr^(2) . Where k is a constant and r is the distance of the particle from origin. Assuming Bohr's model of quantization of angular momentum and circular orbit, r is directly proportional to : (a) n^(2) (b)n (c) sqrt(n) (d)none of these
View SolutionSuppose an electron is attracted toward the origin by a forcekr where k is a constant and r is the distance of the electron from the origin .By applying Bohr model to this system the radius of the nth orbital of the electron is found to be rn and the kinetic energy of the electron to be Tn , Then which of the following is true ?
View SolutionA small particle of mass m moves in such a way that the potential energy U = ar2 where a is a constant and r is the distance of the particle from the origin. Assuming Bohr's model of quantization of angular momentum and circular orbits, find the radius of nth allowed orbit.
View SolutionA small particle of mass m moves in such a way that the potential energy U = ar2 where a is a constant and r is the distance of the particle from the origin. Assuming Bohr's model of quantization of angular momentum and circular orbits, find the radius of nth allowed orbit.
View SolutionSuppose an electron is attracted toward the origin by a forcekr where k is a constant and r is the distance of the electron from the origin .By applying Bohr model to this system the radius of the nth orbital of the electron is found to be rn and the kinetic energy of the electron to be Tn , Then which of the following is true ?
View Solutionsuppose an electron is attracted towards the origin by a force k//r , where k is a constant and r is the distance of the electron form the origin. By applying bohr model to this system, the radius of n^(th) orbit of the electron is found to be r_n and
View SolutionSuppose an electron is attracted towards the origin by a force (k)/(r ) where k is a constant and r is the distance of the electron from the origin. By applying Bohr model to this system, the radius of the n^(th) orbital of the electron is found to be r_(n) and the kinetic energy of the electron to be T_(n) . Then which of the following is true?
View Solution
