To calculate the size of a hydrogen anion using the Bohr model, we assume that its two electrons move in an orbit such that they are always on diametrically opposite sides of the nucleus. With each electron having the angular momentum h=h/2π, and taking electron interaction into account the radius of the orbit in terms of the Bohr radius of hydrogen atom aB=4πε0h2me2 is
aB
43aB
23aB
32aB
The correct Answer is:B
Step by step video, text & image solution for To calculate the size of a hydrogen anion using the Bohr model, we assume that its two electrons move in an orbit such that they are always on diametrically opposite sides of the nucleus. With each electron having the angular momentum h=h//2pi, and taking electron interaction into account the radius of the orbit in terms of the Bohr radius of hydrogen atom a_(B)=(4piepsilon_(0)h^(2))/(me^(2)) is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
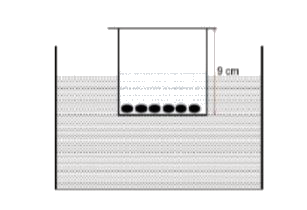
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
View SolutionCalculate the radius of the third Bohr orbit of hydrogen atom and the energy of electron in that orbit.
View SolutionThe ratio of the radius of the Bohr orbit for the electron orbiting the hydrogen nucleus that of the electron orbiting the deuterium nucleus is approximately
View SolutionThe electric current I created by the electron in the ground state of H atom bohr model in terms of bohr radius (a0) and velocity of electron in first orbit v0 is
View Solutionहाइड्रोजन परमाणु के बोहर मॉडल में इलेक्ट्रॉन का दिव्तीय कक्षा में कोणीय संवेग कितना होगा ?
View SolutionThe radius of the first Bohr orbit of the hydrogen atom is 0.53 Å and angular momentum of the electron in that orbit is 1.055×10−34 Js. The linear speed of the electron in the second orbit of the H-atom is
View SolutionCalculate the velocity of an electron in the first Bohr obit. Also calculate the de Broglie wavelength (in 'm') of the electron. Find the orbital angular momentum (in h2π units) of 3p orbital of hydrogen atom.
View SolutionThe angular momentum of an electron in He+ moving in an orbit is h/π. The debrogile wavelength associated with electron is : [ a0 is radius of first bohr's orbit of H - atom]
View SolutionThe kinetic energy of an electron in the second Bohr orbit of a hydrogen atom is [ a_(0) is Bohr radius] :
View SolutionCalculate the following:
(i) Velocity of electron in first Bohr orbit of H-atom (r=a0)
(ii) De Broglie wavelengt of electron in first Bohr orbit of H- atom.
(iii) Orbit angular momentum 2p-orbitals in terms of h2π unit.
View SolutionCalculate the radius of the third Bohr orbit of hydrogen atom and the energy of electron in that orbit.
View SolutionThe ratio of the radius of Bohr first orbit for the electron orbiting the hydrogen nucleus to that of the electron orbiting the deuterium nucleus (mass nearly twice that of H nucleus) is approximately
View SolutionThe kinetic energy of an electron in the second Bohr orbit of a hydrogen atom is [ a_(0) is Bohr radius]
View SolutionThe electric current I created by the electron in the ground state of H atom bohr model in terms of bohr radius (a0) and velocity of electron in first orbit v0 is
View SolutionCalculate angular momentum of an electron in the third Bohr orbit of hydrogen atom.
View Solution