An impulsive force gives an initial velocity of- 1.0ms−1 to the mass in the unstretched spring position [sec Fig. 16.2]. What is the amplitude of motion ? Give x as a function of time t for the oscillating mass. Given m=3 kg and k=1200Nm−1.
To solve the problem, we need to determine the amplitude of motion and the displacement as a function of time for a mass attached to a spring after being given an initial velocity.
Given:
- Mass, m=3kg
- Spring constant, k=1200N/m
- Initial velocity, v0=−1.0m/s
Step 1: Calculate the angular frequency ω
The angular frequency ω for a mass-spring system is given by the formula:
ω=√km
Substituting the values:
ω=√1200N/m3kg=√400=20rad/s
Step 2: Determine the amplitude A
The amplitude can be determined using the initial conditions. The total mechanical energy in the system when the mass is at the unstretched position of the spring (where potential energy is zero) is entirely kinetic energy given by:
E=12mv20
Substituting the values:
E=12×3kg×(−1.0m/s)2=12×3×1=1.5J
The total mechanical energy in the system can also be expressed in terms of amplitude A:
E=12kA2
Setting the two expressions for energy equal to each other:
1.5=12×1200N/m×A2
1.5=600A2
Solving for A2:
A2=1.5600=0.0025
Taking the square root:
A=√0.0025=0.05m=5cm
Step 3: Write the equation of motion x(t)
The equation of motion for a mass-spring system can be expressed as:
x(t)=Acos(ωt+ϕ)
Since the mass is released from the unstretched position with an initial velocity, we can determine the phase constant ϕ. At t=0:
x(0)=0andv(0)=−1.0m/s
Using the cosine function:
x(0)=Acos(ϕ)=0⟹cos(ϕ)=0⟹ϕ=π2 or 3π2
Since the velocity is negative, we take:
ϕ=3π2
Thus, the equation of motion becomes:
x(t)=Acos(ωt+3π2)=0.05cos(20t+3π2)
Using the identity cos(θ+3π2)=−sin(θ):
x(t)=−0.05sin(20t)
Final Answers:
1. Amplitude of motion: A=0.05m or 5cm
2. Displacement as a function of time:
x(t)=−0.05sin(20t)
- Mass, m=3kg
- Spring constant, k=1200N/m
- Initial velocity, v0=−1.0m/s
The angular frequency ω for a mass-spring system is given by the formula:
ω=√km
Substituting the values:
ω=√1200N/m3kg=√400=20rad/s
The amplitude can be determined using the initial conditions. The total mechanical energy in the system when the mass is at the unstretched position of the spring (where potential energy is zero) is entirely kinetic energy given by:
E=12mv20
Substituting the values:
E=12×3kg×(−1.0m/s)2=12×3×1=1.5J
E=12kA2
Setting the two expressions for energy equal to each other:
1.5=12×1200N/m×A2
1.5=600A2
Solving for A2:
A2=1.5600=0.0025
Taking the square root:
A=√0.0025=0.05m=5cm
The equation of motion for a mass-spring system can be expressed as:
x(t)=Acos(ωt+ϕ)
Since the mass is released from the unstretched position with an initial velocity, we can determine the phase constant ϕ. At t=0:
x(0)=0andv(0)=−1.0m/s
Using the cosine function:
x(0)=Acos(ϕ)=0⟹cos(ϕ)=0⟹ϕ=π2 or 3π2
Since the velocity is negative, we take:
ϕ=3π2
Thus, the equation of motion becomes:
x(t)=Acos(ωt+3π2)=0.05cos(20t+3π2)
Using the identity cos(θ+3π2)=−sin(θ):
x(t)=−0.05sin(20t)
1. Amplitude of motion: A=0.05m or 5cm
2. Displacement as a function of time:
x(t)=−0.05sin(20t)
Topper's Solved these Questions
- OSCILLATIONSBOOK - SL ARORACHAPTER - OSCILLATIONSEXERCISE - Type C Based on Oscillations of a Simple Pendulum
7 Videos
- OSCILLATIONSBOOK - SL ARORACHAPTER - OSCILLATIONSEXERCISE - Type D Based on Other Examples of SHM
2 Videos
- OSCILLATIONSBOOK - SL ARORACHAPTER - OSCILLATIONSEXERCISE - Exercise
233 Videos
- MOTION IN ONE DIMENSIONBOOK - SL ARORACHAPTER - MOTION IN ONE DIMENSIONEXERCISE - problems for self practice
66 Videos
- Physical worldBOOK - SL ARORACHAPTER - Physical worldEXERCISE - Exercise
49 Videos
Similar Questions
In example 34, let us take the position of the mass, when the spring is unstretched, as x=0, and the direction from left to right as the positive direction of X-axis. Give x as a function of time t for the oscillating mass, if at the moment we start the stop watch (t=0), the mass is (i) at the mean position (ii) at the maximum stretched position (iii) at the maximum compressed position.
In what do these different functions of SHM differ ? Frequency, amplitude or initial phase ?
View SolutionIn exercise 14.9 let us take the position of mass when the spring is unstretched as x = 0, and the direction from left to right as the positive direction of X-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t = 0), the mass is
(a) at the mean position.
(b) at the maximum stretched position, and
( c ) at the maximum compressed position.
IN what way do these functions for SHM differ from each other, in frequency in amplitude or the initial phase ?
View Solution
Knowledge Check
A 0.20kg object mass attached to a spring whose spring constant is 500N/m executes simple harmonic motion. If its maximum speed is 5.0m/s, the amplitude of its oscillation is
A0.0020mB0.10mC0.20mD25mA mass m=8kg is attached to a spring as shown in figure and held in position so that the spring remains unstretched. The spring constant is 200N/m. The mass m is then released and begins to undergo small oscillations. The maximum velocity of the mass will be (g=10m/s2)
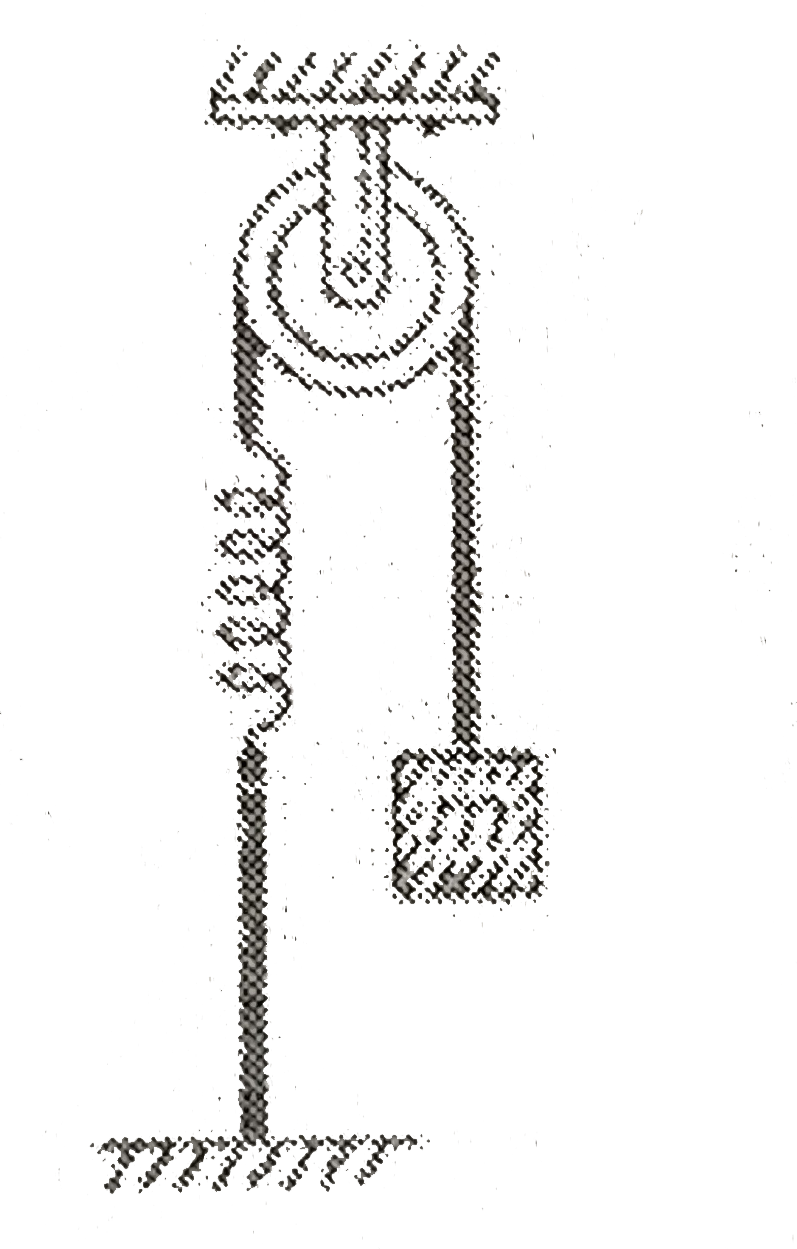 A1 m/sB2 m/sC4 m/sD5 m/s
A1 m/sB2 m/sC4 m/sD5 m/sA ball of mass 0.2 kg moves with a velocity of 20m/sec and it stops in 0.1 sec , then the force on the ball is
A40 NB20 NC4 ND2 N
An object of mass 10 kg is moving with an initial velocity of 10ms−1. A constant force acts for 4 s on the object giving it a speed of 2 m s−1 in the opposite direction. Find the acceleration and force.
View SolutionTwo particles of mass m1 and m2 are connected by a spring of natural length L and force constant k. The masses are brought close enough so as to compress the spring completely and a string is used to tie the system. Assume that length of spring in this position is close to zero. The system is projected with a velocity V0 along the positive x direction. At the instant it reaches origin at time t = 0, the string snaps and the spring starts opening.
(a) Show that the mass m1(orm2) will are perform SHM in the reference frame attached to the centre of mass of the system. Find the time period of oscillation.
(b) Write the amplitude of m1 and m2 as a function of time. lt( c) Write the X co ordinates of m1 and m2 as a function of time

View SolutionAn object of mass 10 kg is moving with an initial velocity of 10ms−1. A constant force acts for 4 s on the object giving it a speed of 2 m s−1 in the opposite direction. Find the acceleration and force.
View SolutionThe velocity of the particle of mass m as a function of time t is given by v=Aω.cos[√Kmt] , where A is amplitude of oscillation. The dimension of AK is
View SolutionA mass m attached to a light spring oscillates with a period of 2 s. If the mass is increased by 2 kg, the period increases by 1 s. Then the value of m is
View Solution
