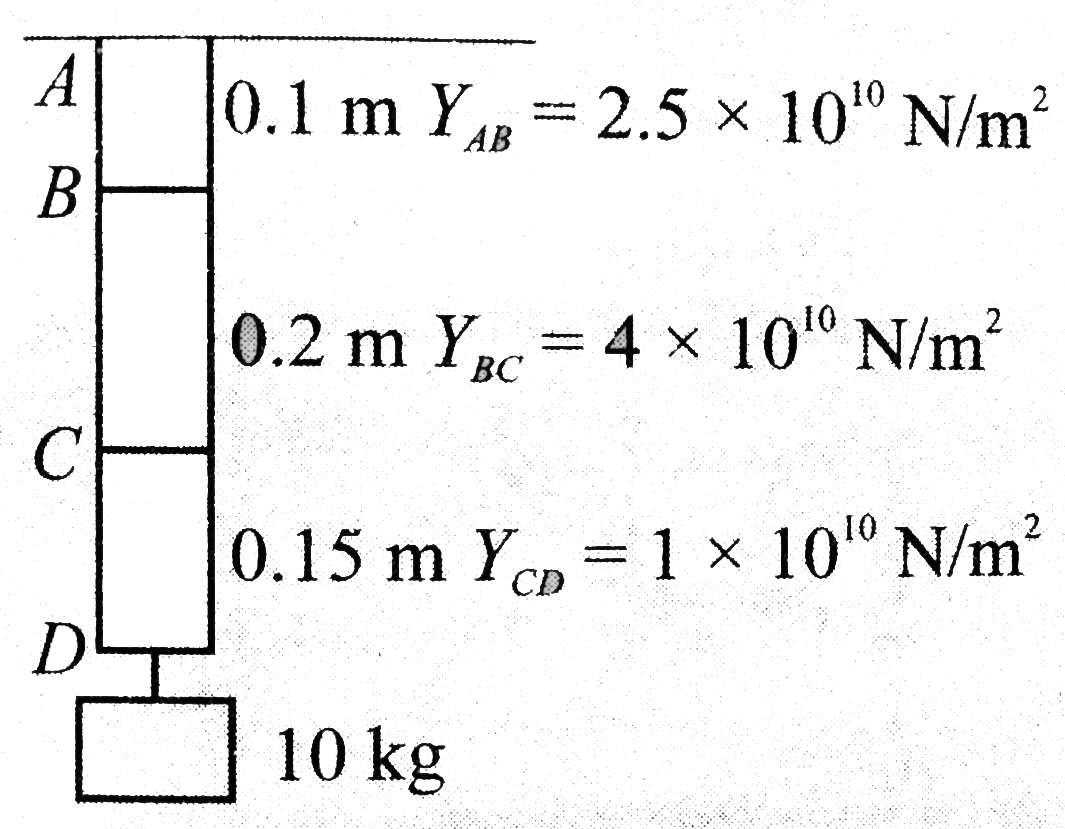
A rod AD consisting of three segments AB, BC and CD joined together is hanging vertically from a fixed support at A. The lengths of the segments are respectively : 1 m, 0.2 m and 0.15 m. The cross section of the rod is uniformly $10^{- 4} m^{2}$ . A weight of 10 kg is hung from D. Calculate the displacements of point of B, C and D using the data of Young's moduli given below (neglect the weight of the rod).
$Y_{A B} = 2.5 \times 10^{10} N / m^{2}$ ,
$Y_{B C} = 4.0 \times 10^{10} N / m^{2}$ ,
$Y_{C D} = 1.0 \times 10^{10} N / m^{2}$

Video Solution

Answer

Step by step video & image solution for A rod AD consisting of three segments AB, BC and CD joined together is hanging vertically from a fixed support at A. The lengths of the segments are respectively : 1 m, 0.2 m and 0.15 m. The cross section of the rod is uniformly 10^(–4) m^(2). A weight of 10 kg is hung from D. Calculate the displacements of point of B, C and D using the data of Young's moduli given below (neglect the weight of the rod). Y_(AB) =2.5xx10^(10) N//m^(2), Y_(BC)=4.0xx10^(10) N//m^(2), Y_(CD) =1.0 xx10^(10) N//m^(2) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS PROPERTIES OF SOLIDS AND FLUIDS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
A wire of length L is hanging from a fixed support. The length changes to $L_{1} and L_{2}$ when masses $M_{1} and M_{2}$ are suspended respectively from its free end. Then L is equal to
A $\frac{L_{1} + L_{2}}{2}$
B $\sqrt{L_{1} L_{2}}$
C $\frac{L_{1} M_{2} + L_{2} M_{1}}{M_{1} + M_{2}}$
D $\frac{L_{1} M_{2} - L_{2} M_{1}}{M_{2} - M_{1}}$
Question 2 - Select One
A unifrom steel rod of length $1 m$ and area of cross-section $20 c m^{2}$ is hanging from a fixed support. Find the increases in the length of the rod.
A $1.923 \times 10^{- 5} c m$
B $2.923 \times 10^{- 5} c m$
C $1.123 \times 10^{- 5} c m$
D $3.123 \times 10^{- 5} c m$
Question 3 - Select One
A brass of length $2 m$ and cross-sectional area $2.0 c m^{2}$ is attached end to end to a steel rod of length and cross-sectional area $1.0 c m^{2}$ . The compound rod is subjected to equal and oppsite pulls of magnitude $5 \times 10^{4} N$ at its ends. If the elongations of the two rods are equal, the length of the steel rod $(L)$ is
{ $Y_{B r a s s} = 1.0 \times 10^{11} N / m^{2}$ and $Y_{s t e e l} = 2.0 \times 10^{11} N / m^{2}$
A $1.5 m$
B $1.8 m$
C $1 m$
D $2 m$

SL ARORA-PROPERTIES OF SOLIDS-All Questions

Home
Ask Doubt
Profile

Topper's Solved these Questions

Similar Questions

Knowledge Check

A wire of length L is hanging from a fixed support. The length changes to L1andL2 when masses M1andM2 are suspended respectively from its free end. Then L is equal to

A unifrom steel rod of length 1m and area of cross-section 20cm2 is hanging from a fixed support. Find the increases in the length of the rod.

SL ARORA-PROPERTIES OF SOLIDS-All Questions

A wire of length L is hanging from a fixed support. The length changes to $L_{1} and L_{2}$ when masses $M_{1} and M_{2}$ are suspended respectively from its free end. Then L is equal to

A unifrom steel rod of length $1 m$ and area of cross-section $20 c m^{2}$ is hanging from a fixed support. Find the increases in the length of the rod.