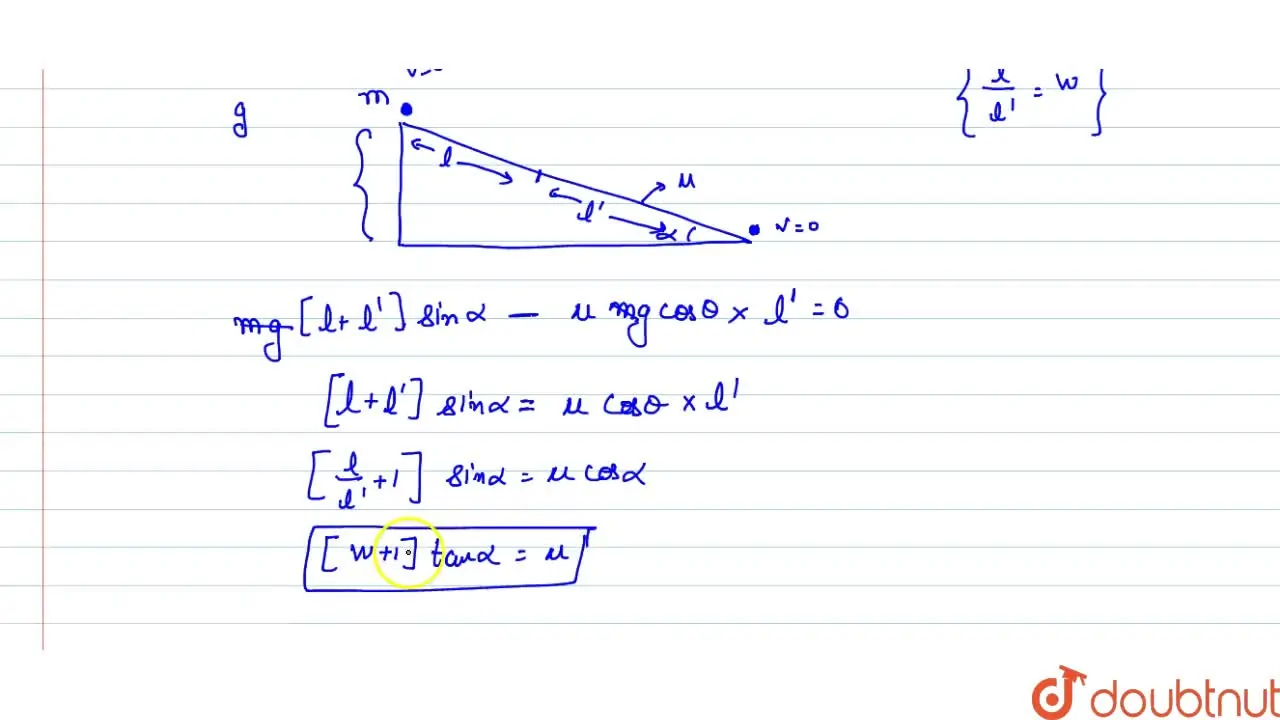
The upper portion of an inclined plane of inclination α is smooth and the lower portion is rough. A particle slides down from rest from the top and just comesto rest at the foot.If the ratio ofthe smooth length to rough length is w : find the coefficient of friction.
The correct Answer is:[m+nn]
Step by step video, text & image solution for The upper portion of an inclined plane of inclination alpha is smooth and the lower portion is rough. A particle slides down from rest from the top and just comesto rest at the foot.If the ratio ofthe smooth length to rough length is w : find the coefficient of friction. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Advance MCQs17videos
NEWTONS LAWS OF MOTION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - exercise 1.33videos
LINEAR MOMENTUM & ITS CONSERVATION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Unsolved Numerical63videos
RIGID BODIES AND ROTATIONAL MOTION
Similar Questions
The upper portion of an incline plane of inclination α is smooth and the lower portion is rough. A particle slides down from rest from the top and just comes to rest at the fool. If the ratio of the smooth length to rough length is 2:1 and coefficient of frection is n tanα, then write the value of n.
View SolutionThe upper portion of an inclined plane of inclination a is smooth and the lower portion is rough. A partical slide down from rest the top and just comes to rest at the foot . If the ratio of smooth length to rough length is m:n, find the coefficient of friction .
View SolutionThe upper half of an inclined plane with inclination ϕ is perfectly smooth while the lower half is rough. A body starting from rest at the top will again come to rest at the bottom if the coefficient of friction for the lower half is given by
View SolutionThe upper half of an inclined plane of inclination 45∘ is perfectly smooth while the lower half is rough. A block starting from rest at the top comes back to rest at the bottom. The coefficient of friction for the lower half is
View SolutionA block of mass 5.0kg slides down from the top of an inclined plane of length 3m. The first 1m of the plane is smooth and the next 2m is rough. The block is released from rest and again comes to rest at the bottom of the plane. If the plane is inclined at 30∘ with the horizontal, find the coefficient of friction on the rough portion.
 View Solution
View SolutionThe upper half of an inclined plane of inclination 'θ' is perfectly smooth while the lower half is rough. A block starting from rest at the top of the plane will again come to rest at the bottom. The coefficient of friction between the block and the lower half of the plane is given by
View SolutionThe upper half of an inclined plane of inclination θ is perfectly smooth while lower half is rough . A block starting from rest at the top of the plane will again comes to rest at the bottom , if the coefficient of friction between the block and lower hald of the plane is given by
View SolutionThe upper half of an inclined plane of inclination θ is perfectly smooth while lower half is rough. A block starting from rest at the top of the plane will again come to rest at the bottom, if the coefficient of friction between the block and lower half of the plane is given by
View SolutionA particle is resting on a rough inclined plane with inclination α The angle of friction is λ. The particle will be at rest if and only if
View SolutionA body is released from rest from the top of an inclined plane of length L and angle of inclination θ ,.The top of plane of length LN (N>1) is smooth and the remaining part is rough.If the body comes to rest on reaching the bottom of the plane, then the value of coefficient of friction of rough surface is ?
View Solution