A body of mass 0.42kg oscillates about a horizontal xis distant 0.25mm frim its CG. If the length of am equivalent simple pendulum be 0.35m, find its moment of inertia about its axis of suspension, and its period of oscillation. (g=9.8ms−2)
[HintL=k2l+l,l=mk2+ml2.]
The correct Answer is:0.03675kgm2,1.1874s
Step by step video, text & image solution for A body of mass 0.42kg oscillates about a horizontal xis distant 0.25mm frim its CG. If the length of am equivalent simple pendulum be 0.35m, find its moment of inertia about its axis of suspension, and its period of oscillation. (g=9.8ms^(-2)) "[Hint" L=(k^(2))/(l)+l,l=mk^(2)+ml^(2)."]" by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE AND COMPOUND PENDULUMBook:NN GHOSHChapter:SIMPLE AND COMPOUND PENDULUMExercise:Exercise
Explore 24 Videos
- SECOND LAW OF THERMODYNAMICSBook:NN GHOSHChapter:SECOND LAW OF THERMODYNAMICSExercise:All Questions
Explore 7 Videos
- SIMPLE HARMONIC MOTIONBook:NN GHOSHChapter:SIMPLE HARMONIC MOTIONExercise:Eexercies
Explore 39 Videos
Similar Questions
A body of mass 0.2 kg oscillates about a horizental axis at a distance 20 cm from its centre ofr gravity. Ifd the length of the equivalent simple pendulem be 0.35 m, find the moment of inertia about the axis of suspension.
View SolutionA body of mass 200g oscillates about a horizontal axis at a distance of 20cm from its centre of gravity . If the length of the equivalent simple pendulum is 35cm, find its moment of inertia about the point of suspension.
View Solution
Knowledge Check
A thin wire of mass M and length L is turned into a ring. The moment of inertia about its axis will be:
A14π2ML2B112ML2C13π2ML2D1π2ML2A solid cube of side l is made to oscillate about a horizontal axis passing through one of its edges. Its time period will be
A2π√2√23lgB2π√23lgC2π√√32lgD2π√2√3lgThe mass of a solid body is 200 gm and oscillates about a horizontal axis at a distance of 20 cms from the centre of gravity. If length equivalent to simple pendulum is 40 cms, its M.I. through the centre of gravity will be :
A8×104gm−cm2B8×104kg−cm2C8×104gm−m2D8×104kg−cm2
If the length of a simple pendulum is decreased by 2%, find the percentage decrease in its period T, where T=2π√lg
View SolutionA wire of mass m and length l is bent in the form of circular ring. The moment of inertia of the ring about its axis is
View SolutionA wire of mass m and length l is bent in the form of circular ring. The moment of inertia of the ring about its axis is
View SolutionA thin wire of length l and mass m is turned into the form of a semicircle. Its moment of inertia about an axis joining its free ends will be
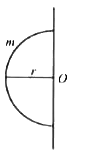
View SolutionA thin wire of length l and mass m is bent in the form of a semicircle as shown in the figure. Its moment of inertia about an axis joining its free ends will be

View Solution