the base of cylindrical vessel is hemesphericl in shape with rdius R . If the vessel is filled with liquid of density p up to a height h. calculate the thrust on the base.
The correct Answer is:2πR2pg(h4−R3)
Step by step video, text & image solution for the base of cylindrical vessel is hemesphericl in shape with rdius R . If the vessel is filled with liquid of density p up to a height h. calculate the thrust on the base. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A long cylindrical vessel is half filled with a liquid. When the vessel is rotated about its own vertical axis, the liquid rises up near the wall. If the radius of vessel is 5 cm and its rotational speed is 2 rotations per second, then the difference in the heights between the centre and the sides, in cm, will be:
View SolutionTwo identical cylindrical vessels with their bases at the same level each, contain a liquid of density ρ. The area of either base is A but in one vessel the liquid heigth is h1 and in the other liquid height is h2(h2<h1). If the two vessel are connected, the work done by gravity in equalizing the level is
View Solution
Knowledge Check
A liquid of density ρ is filled in two indentical cylindrical vessels with base area A and height of the liquid in one vessel is h1 and that in the other vessel is h2. If both the vesels are connected with their base at the same level then calculate the work done by gravity in equalizing the levels.
A(h1−h2)gρB(h1−h2)gAρC12(h1−h2)2gAρD14(h1−h2)2gAρA uniformly tapering vessel of height h whose lower and upper radii are r and R is completely filled with a liquid of density ρ The force that acts on the base of the vessel due of the liquid is
AπR2hρgBπr2hρgCπ(R+π2)2hρgD13π(R2−r2)hρgA cylindrical vessel 16 cm high and 9 cm as the radius of the base, is filled with sand. This vessel is emptied on the ground and a conical heap of sand is formed. If the height of the conical heap is 12 cm, the radius of its base is
A12 cmB18 cmC36 cmD48 cm
A cylindrical vessel of radius R=1m and height H=3m is filled with an ideal liquid up to a height of h=2 m. the contaienr with liquid is rotated about its central vertical axis such that the liquid just rises to the brim. Calculate the angular speed (ω) of the container.
View SolutionA liquid has been poured into a cylindrical vessel of mass M (the mass of the vessel bottom can be ighored) and height H. The linear density of the liquid, that is, the ratio of the mass of the liquid column to its height, is δ. What is the height x of the column of liquid at which the common center of gravity of the liquid plus the vessel is in the lowest position?
View SolutionA conical vessel wihout a bottom stands on a table. A liquid is poured with the vessel & as soon as the level reaches h, the pressure of the liquid raises the vessel. The radius of the base of the vessel is R and half angle of the cone α and the weight of the vessel is W. What is the density of the liquid ?
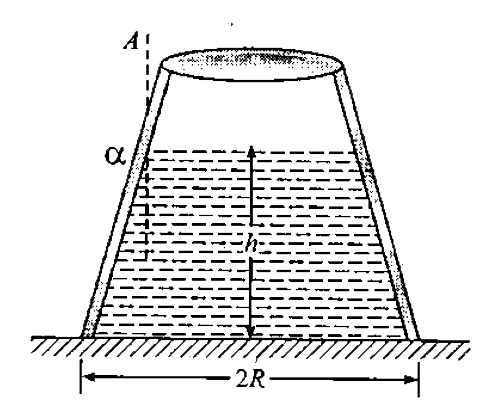
View SolutionThere is a hole of area 125cm2 in the bottom of a cylindrical vessel containing fluid up to height h. The liquid flows out in time t. If the liquid were filled in the vessel up to height 4h, then it would flow out in time
View SolutionTo what height h should a cylindrical vessel of diameter d be filled with a liquid so that the total force on the vertical surface of the vessel be equal to the force on the bottom-
View Solution
