Two particles A and B move anticlockwise with the same speed v in a circle of radius R and are diametrically opposite to each other. At , A is given a constant accelerartion (tangential) . Calculate the time in which a collides with B, the angle traced by A, its angular velocity and radial acceleration at the time of collision.
The correct Answer is:
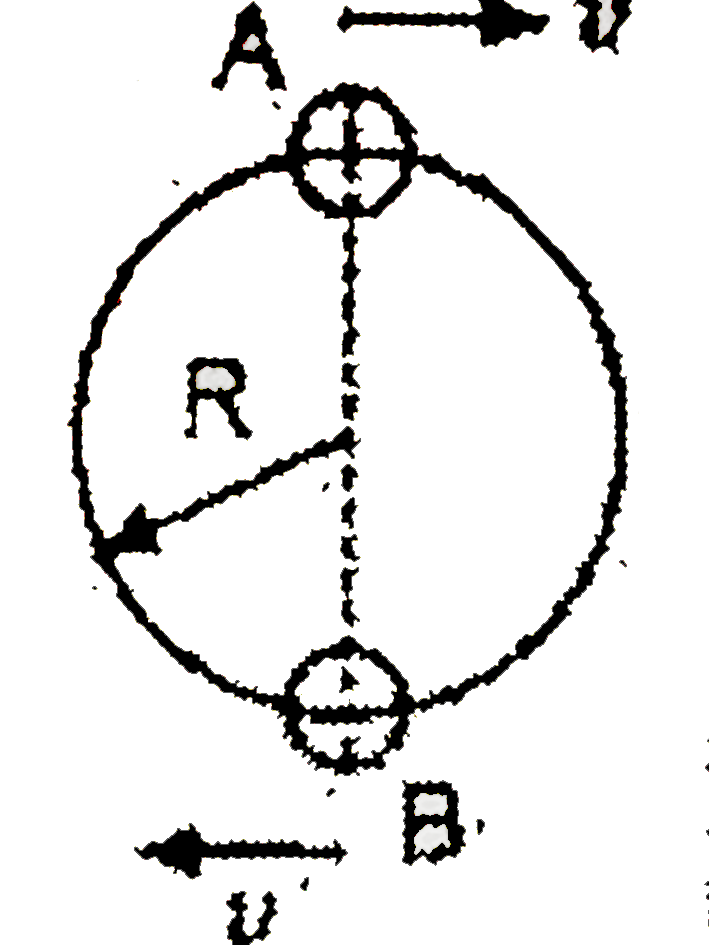
Step by step video, text & image solution for Two particles A and B move anticlockwise with the same speed v in a circle of radius R and are diametrically opposite to each other. At t=0, A is given a constant accelerartion (tangential) a_(t)=(72v^(2))/(25 pi R). Calculate the time in which a collides with B, the angle traced by A, its angular velocity and radial acceleration at the time of collision. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CENTRIPITAL AND CENTRIFUGAL FORCESBOOK - NN GHOSHCHAPTER - CENTRIPITAL AND CENTRIFUGAL FORCESEXERCISE - Exercises (A)
18 Videos
- CALORIMETRYBOOK - NN GHOSHCHAPTER - CALORIMETRYEXERCISE - All Questions
28 Videos
- COMPOSITION AND RESOLUTION OF VELOCITIESBOOK - NN GHOSHCHAPTER - COMPOSITION AND RESOLUTION OF VELOCITIESEXERCISE - Exercise
25 Videos
Similar Questions
The speed (v) of a particle moving in a circle of radius R varies with distance s as v=ks where k is a positive constant.Calculate the total acceleration of the particle
View SolutionTwo particles AandB move on a circle Initially, particles AandB are diagonally opposite to each other. Particle A moves with angular velocity πrads−1 and angular acceleration π/2rads−2 and particle B moves with constant angular velocity 2πrads−2. Find the time after which both the particlsd AnadB will collide.
View Solution
Knowledge Check
A particle with the constant speed in a circle of radius r and time period T.The centripetal acceleration of a particle is
A‘r2‘TB‘T2‘rC(2πT)2rD(2πT)2rIf the body is moving in a circle of radius r with a constant speed v , its angular velocity is
Av2/rBvrCv/rDr/vTwo particles A and B separated by a distance 2R are moving counter clockwise along the same circular path of radius R each with uniform speed v. At time t=0, A is given a tangential acceleration of magnitude a=32v225πR in the same direction of initial velocity
AThe time lapse for the two bodies to collide is 6πR5VBThe angle coverd by A is 9π4CAngular velocity of A is 11V5RDRadial acceleration of A is 289υ25R
A particle moves with constant speed v along a circular path of radius r and completes the circle in time T. The acceleration of the particle is
View SolutionA particle of mass M is moving in a horizontal circle of radius R with uniform speed V. When it moves from one point to a diametrically opposite point, its
View SolutionA particle of mass M is moving in a horizontal circle of radius R with uniform speed V. When it moves from one point to a diametrically opposite point , its
View SolutionTwo bodies are moving with constant speed v clockwise and are initially diagonally opposite. The particle B now acheives a tangential acceleration of a m/s2. Then :
View SolutionA particle moves in circle of radius R with a constant speed v. Then, find the magnitude of average
acceleration during a time interval πR2v.
View Solution
