A simple pendulum consists of a heavy mass supported by a light, thin rod. It is held inverted in the position of unstable equilibrium and then released. Find the angle defining the position of its downward fall at which the axial force in the rod changes from compression to tension.
The correct Answer is:48∘11'
Step by step video, text & image solution for A simple pendulum consists of a heavy mass supported by a light, thin rod. It is held inverted in the position of unstable equilibrium and then released. Find the angle defining the position of its downward fall at which the axial force in the rod changes from compression to tension. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CENTRIPITAL AND CENTRIFUGAL FORCESBOOK - NN GHOSHCHAPTER - CENTRIPITAL AND CENTRIFUGAL FORCESEXERCISE - Exercises (A)
18 Videos
- CALORIMETRYBOOK - NN GHOSHCHAPTER - CALORIMETRYEXERCISE - All Questions
28 Videos
- COMPOSITION AND RESOLUTION OF VELOCITIESBOOK - NN GHOSHCHAPTER - COMPOSITION AND RESOLUTION OF VELOCITIESEXERCISE - Exercise
25 Videos
Similar Questions
AS simple pendulum consists of a 50 cm long string connected to a 100 g ball. The ball is pulled aside so that the string makes an angel of 370 with the verticl and is then released. Find the tension in the string when the bob is at its lowest position.

View SolutionIf a simple pendulum of mass m is released from a horizontal position, find the tension in the string as a function of the angle θ with the horizontal.
View Solution
Knowledge Check
A simple pendulum A and a homogeneous rod B hinged at its enda are released from horizontal position as shown in figure.
 AThe times period of swing of simple pendulum is greater than that of rod If L=lBThe times period of swing of simple pendulum is equal to that of rod If L=lCThe times period of swing of simple pendulum is greater than that of rod If L=2l3DThe times period of swing of swing of simle pendulum is equal to that of rod if L=2l3
AThe times period of swing of simple pendulum is greater than that of rod If L=lBThe times period of swing of simple pendulum is equal to that of rod If L=lCThe times period of swing of simple pendulum is greater than that of rod If L=2l3DThe times period of swing of swing of simle pendulum is equal to that of rod if L=2l3A simple pendulum consists of a ball of mass m suspended from the ceiling using a string of length L. The ball is displaced from its equilibrium position by a small angle θ. What is the magnitude of the restoring force that moves the toward its equilibrium position and produces simple harmonic motion?
AkxBmgCmg(cosθ)Dmg(sinθ)A rod of mass m and length l is hinged at 'its' end and released from rest in position shown. Find the force exerted by the hinge when the rod becomes horizontal
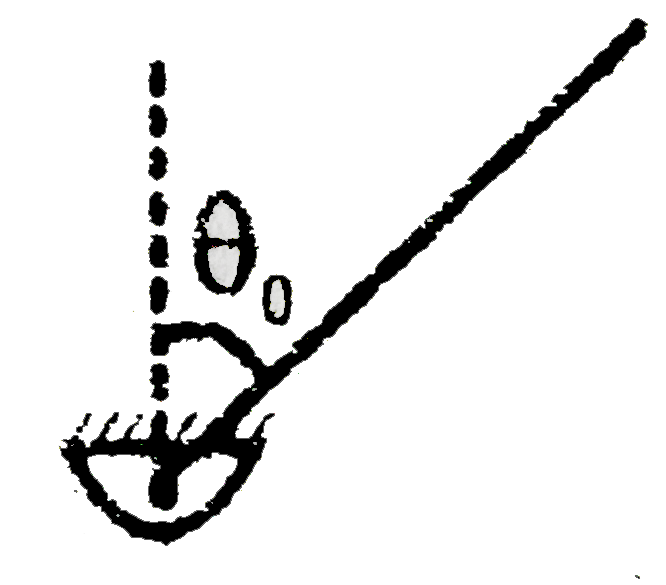 Amg4B3mg2cosθCmg4√9cos2θ+14Dmg2√9cos2θ+14
Amg4B3mg2cosθCmg4√9cos2θ+14Dmg2√9cos2θ+14
As shown in the figure, two blocks, each of mass m, suspended from the ends of a rigid light rod of length L. The rod is held horizontally on the fulcrum and then released.
(a) Find the initial angular acceleration of the rod.
(b) If the mass of rod is also m uniformly distributed. find the tension in the strings attached to blocks.
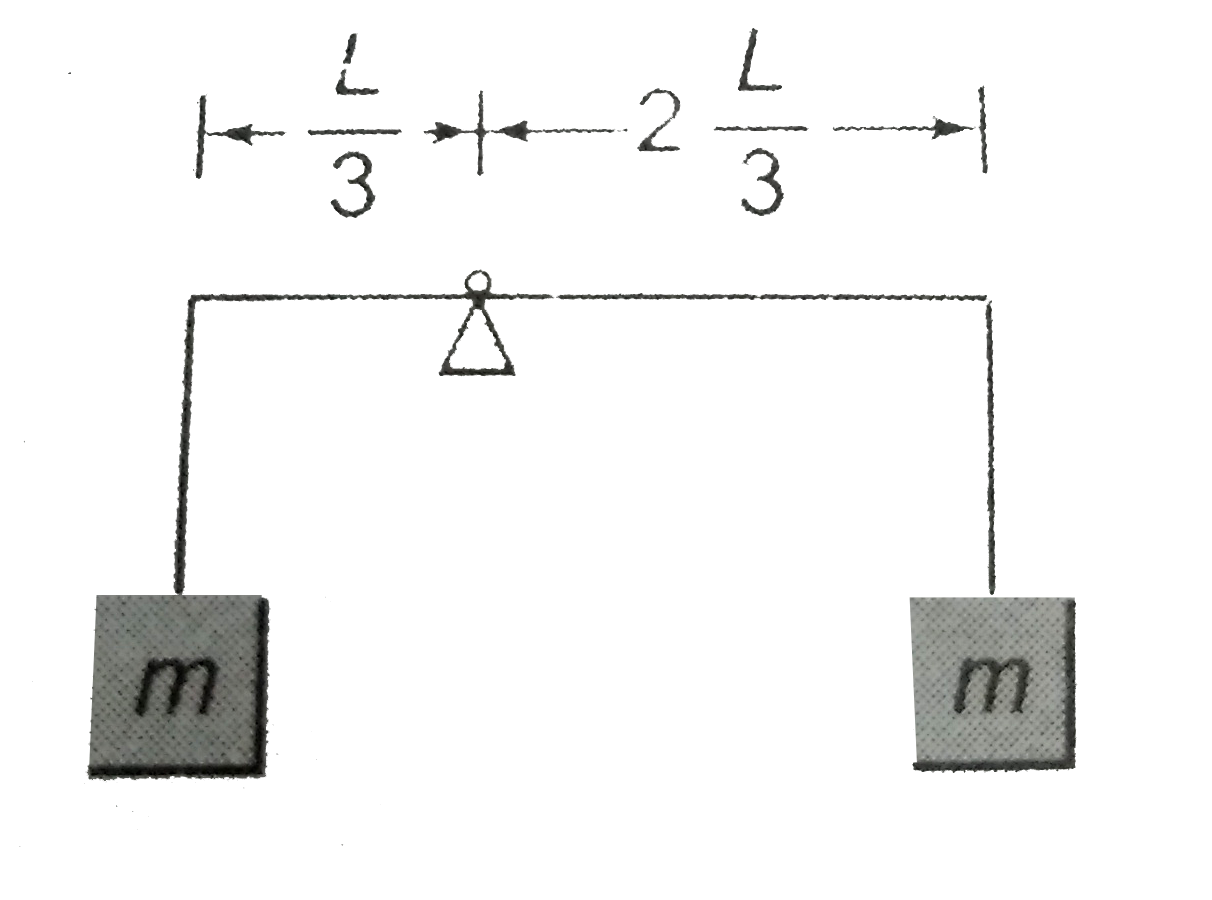
View SolutionThe bob of pendulum of length l is pulled aside from its equilibrium position through an angle θ and then released. Find the speed v with which the bob passes through the equilibrium position.
View SolutionA simple pendulum consists of a small sphere of mass m suspended by a thread of length l. The sphere carries a positive charge q. The pendulum is placed in a uniform electric field of strength E directed vertically downwards. Finds the period of oscillation of the pendulum due to the electrostatic force acting on the sphere, neglecting the effect of the gravitational force.
View SolutionA rod of mass m and length l is held vertically on a smooth horizontal floor. Now it is released from this position, find the speed of its centre of mass when it makes an angle θ with the vertical.
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View Solution
