Question

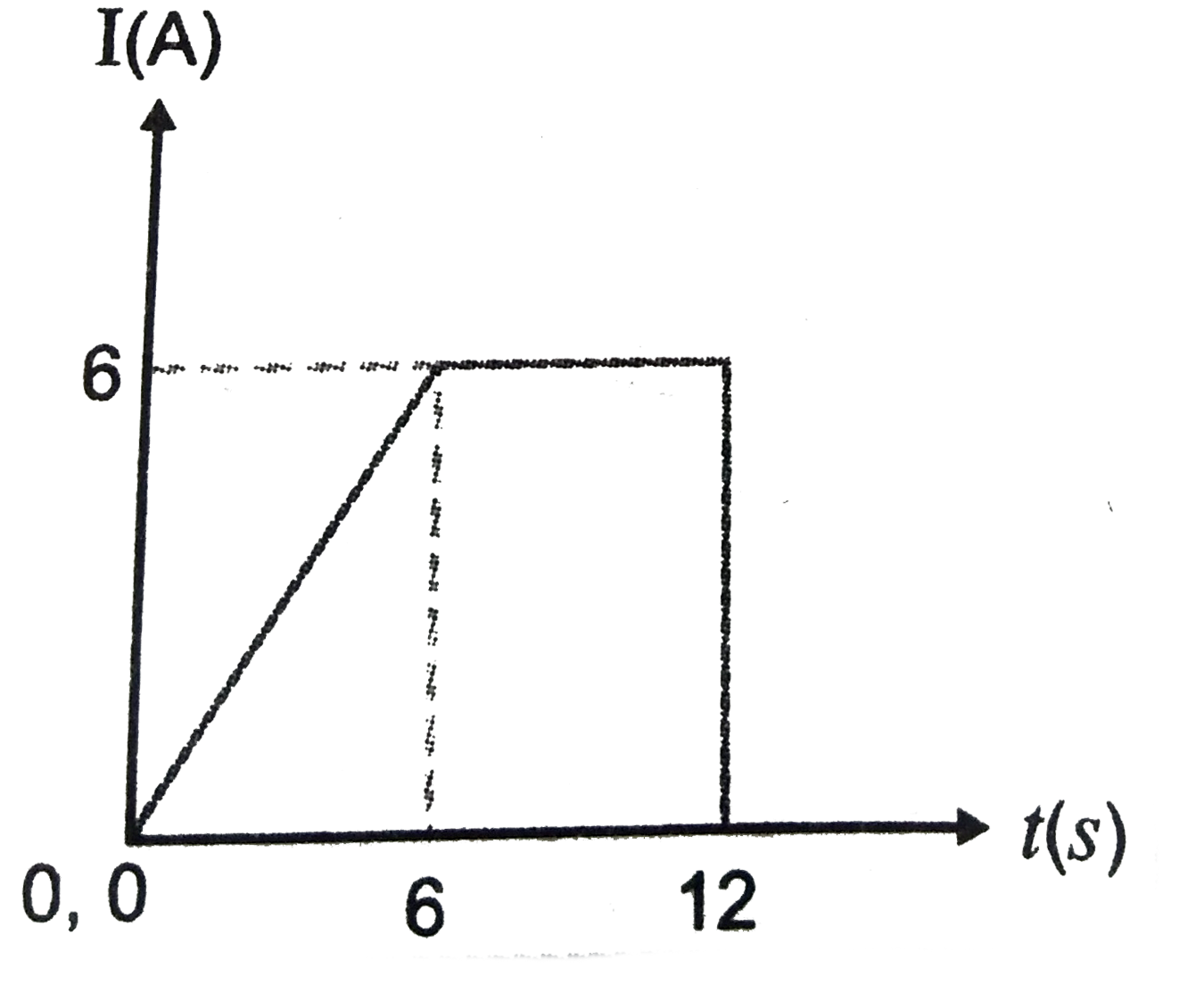
With t taken in seconds and I taken in amp, the variation of I follows the equation $t^2+I^2=25$
What amount of Ag will be electrodeposited with this current flowing in the interval 0-5 second? (Ag=108)

Options

A. 22 mg
B. 66 mg
C. 77 mg
D. 88 mg

Answer

A

Explanation

To solve the problem, we need to find the amount of silver (Ag) that will be deposited when a current varies according to the equation $$t^2+I^2=25$$ over the interval from 0 to 5 seconds.

1. Understand the Equation:
The equation \( t^2 + I^2 = 25 \) describes a relationship between time \( t \) and current \( I \). We can rearrange this to express \( I \) in terms of \( t \):
\( I = \sqrt{25 - t^2} \)

2. Determine the Current Over Time:
We need to calculate the total charge \( Q \) that flows through the circuit from \( t = 0 \) to \( t = 5 \) seconds. The charge can be calculated using the formula:
\( Q = \int_{0}^{5} I(t) \, dt = \int_{0}^{5} \sqrt{25 - t^2} \, dt \)

3. Evaluate the Integral:
The integral \( \int \sqrt{25 - t^2} \, dt \) can be solved using trigonometric substitution. Let \( t = 5 \sin(\theta) \), then \( dt = 5 \cos(\theta) \, d\theta \). The limits change from \( t = 0 \) to \( t = 5 \) which corresponds to \( \theta = 0 \) to \( \theta = \frac{\pi}{2} \).
\( Q = \int_{0}^{\frac{\pi}{2}} \sqrt{25 - (5 \sin(\theta))^2} \cdot 5 \cos(\theta) \, d\theta \)
Simplifying inside the square root:
\( Q = \int_{0}^{\frac{\pi}{2}} \sqrt{25(1 - \sin^2(\theta))} \cdot 5 \cos(\theta) \, d\theta = \int_{0}^{\frac{\pi}{2}} 5 \cdot 5 \cos^2(\theta) \, d\theta = 25 \int_{0}^{\frac{\pi}{2}} \cos^2(\theta) \, d\theta \)

4. Use the Integral of Cosine Squared:
The integral \( \int \cos^2(\theta) \, d\theta \) can be evaluated using the identity:
\( \int \cos^2(\theta) \, d\theta = \frac{1}{2} \left( \theta + \frac{1}{2} \sin(2\theta) \right) \)
Evaluating from \( 0 \) to \( \frac{\pi}{2} \):
\( \int_{0}^{\frac{\pi}{2}} \cos^2(\theta) \, d\theta = \frac{\pi}{4} \)
Therefore,
\( Q = 25 \cdot \frac{\pi}{4} = \frac{25\pi}{4} \)

5. Calculate the Amount of Silver Deposited:
According to Faraday's law, the amount of substance deposited is given by:
\( m = \frac{Q \cdot M}{F} \)
where \( M \) is the molar mass of silver (Ag = 108 g/mol) and \( F \) is Faraday's constant (\( F = 96500 \, C/mol \)):
\( m = \frac{\frac{25\pi}{4} \cdot 108}{96500} \)

6. Final Calculation:
Calculate \( m \):
\( m = \frac{25 \cdot 108 \cdot \pi}{4 \cdot 96500} \)
This can be simplified to find the numerical value.