Two identical billiard balls are in contact on a table. A third identical ball strikes them symmetrically and comes to rest after impact. The coefficient of restitution is :
The correct Answer is:A
To solve the problem, we need to determine the coefficient of restitution (e) for the scenario described. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Problem
We have three identical billiard balls. Two balls are in contact and stationary, while a third ball strikes them symmetrically and comes to rest after the impact. We need to find the coefficient of restitution.
Step 2: Define the Variables
- Let the mass of each billiard ball be m.
- Let the initial velocity of the third ball (the one that strikes) be v0.
- After the collision, the two balls will move with a certain velocity v.
Step 3: Analyze the Collision
Since the third ball strikes the two stationary balls symmetrically, we can assume that the two balls will move off at equal angles after the collision. The collision is perfectly elastic, and we can apply the conservation of momentum.
Step 4: Apply Conservation of Momentum
Before the collision:
- Total initial momentum = mv0 (only the third ball is moving)
After the collision:
- Let the velocity of each of the two balls after the collision be v.
- The total momentum after the collision = 2mv (both balls are moving)
Setting the initial momentum equal to the final momentum:
mv0=2mv
Cancelling m from both sides gives:
v0=2v(1)
Step 5: Determine the Velocity Components
Since the balls move symmetrically, they will move at an angle of 30∘ to the original direction of the incoming ball. The velocity components can be expressed as:
- The horizontal component of the velocity for each ball after the collision is vcos(30∘).
Step 6: Calculate the Coefficient of Restitution
The coefficient of restitution (e) is defined as:
e=Relative velocity of separationRelative velocity of approach
Relative Velocity of Separation
After the collision, the relative velocity of separation of one of the balls moving away from the third ball (which comes to rest) is:
vsep=vcos(30∘)
Relative Velocity of Approach
The relative velocity of approach before the collision is:
vapp=v0
Step 7: Substitute Values
Using the values from step (1) and the cosine of 30∘:
cos(30∘)=√32
Thus, we can express e as:
e=vcos(30∘)v0=v⋅√32v0
From equation (1), we know v=v02:
e=(v02)⋅√32v0=v0√34v0=√34
Step 8: Final Calculation
Thus, the coefficient of restitution is:
e=23
Conclusion
The coefficient of restitution for the collision is 23.
---
We have three identical billiard balls. Two balls are in contact and stationary, while a third ball strikes them symmetrically and comes to rest after the impact. We need to find the coefficient of restitution.
- Let the mass of each billiard ball be m.
- Let the initial velocity of the third ball (the one that strikes) be v0.
- After the collision, the two balls will move with a certain velocity v.
Since the third ball strikes the two stationary balls symmetrically, we can assume that the two balls will move off at equal angles after the collision. The collision is perfectly elastic, and we can apply the conservation of momentum.
Before the collision:
- Total initial momentum = mv0 (only the third ball is moving)
- Let the velocity of each of the two balls after the collision be v.
- The total momentum after the collision = 2mv (both balls are moving)
mv0=2mv
Cancelling m from both sides gives:
v0=2v(1)
Since the balls move symmetrically, they will move at an angle of 30∘ to the original direction of the incoming ball. The velocity components can be expressed as:
- The horizontal component of the velocity for each ball after the collision is vcos(30∘).
The coefficient of restitution (e) is defined as:
e=Relative velocity of separationRelative velocity of approach
After the collision, the relative velocity of separation of one of the balls moving away from the third ball (which comes to rest) is:
vsep=vcos(30∘)
The relative velocity of approach before the collision is:
vapp=v0
Using the values from step (1) and the cosine of 30∘:
cos(30∘)=√32
Thus, we can express e as:
e=vcos(30∘)v0=v⋅√32v0
e=(v02)⋅√32v0=v0√34v0=√34
Thus, the coefficient of restitution is:
e=23
The coefficient of restitution for the collision is 23.
Topper's Solved these Questions
- IMPULSE AND MOMENTUMBOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - L-2
21 Videos
- IMPULSE AND MOMENTUMBOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - L-3,(P-1)
5 Videos
- IMPULSE AND MOMENTUMBOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - Example
50 Videos
- FORCE ANALYSISBOOK - ANURAG MISHRACHAPTER - FORCE ANALYSISEXERCISE - Matching type
13 Videos
- RIGID BODY MOTIONBOOK - ANURAG MISHRACHAPTER - RIGID BODY MOTIONEXERCISE - MATCH THE COLUMN
12 Videos
Similar Questions
Two billiard balls of same size and mass are in contact on a billiard table. A third ball of same mass and size strikes them symmetrically and remains at rest after the impact. Find the coefficient of restitution between the balls?
View SolutionTwo billiard balls of same size and mass are in contact on a billiard table. A third ball of same mass and size strikes them symmetrically and remains at rest after the impact. Find the coefficient of restitution between the balls?
View Solution
Knowledge Check
Two equal spheres of mass m are in contact on a smooth horizontal table. A third identical sphere impinges symmetrically on them and reduces to rest. Then:
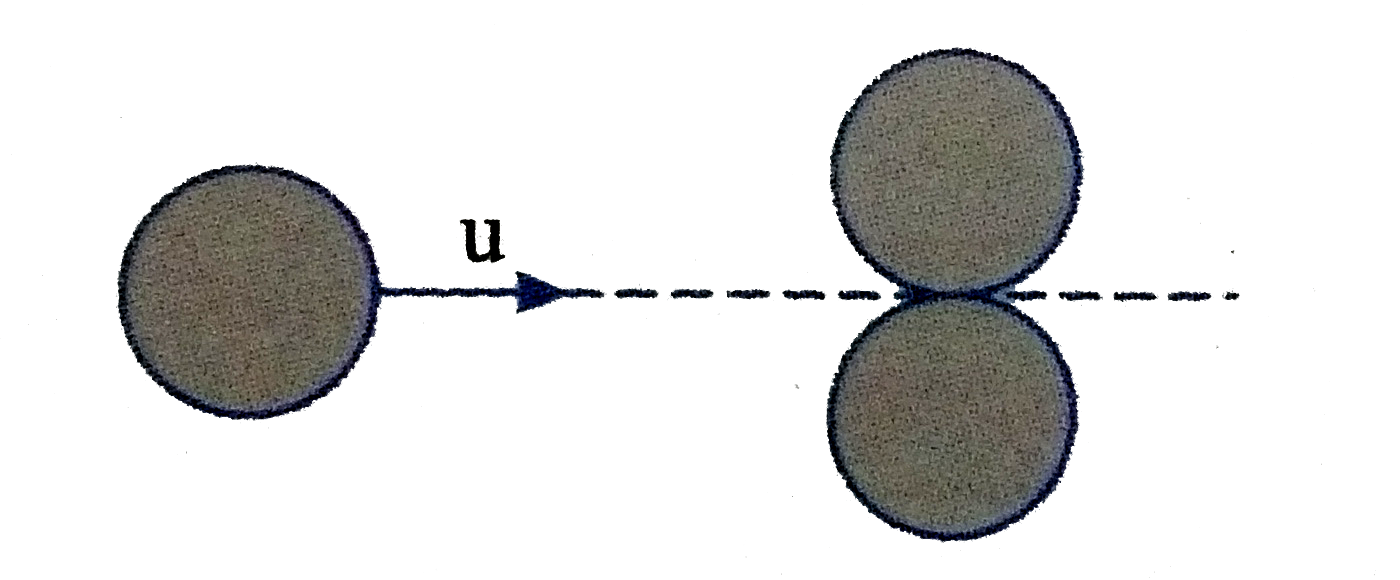 ACoefficient of restitution is e=23BLoss of kinetic energy 16mu2 where u is velocity before impactCAfter the collision, velocity of equal mass sphere is u√3DLoss of kinetic energy 13mu2
ACoefficient of restitution is e=23BLoss of kinetic energy 16mu2 where u is velocity before impactCAfter the collision, velocity of equal mass sphere is u√3DLoss of kinetic energy 13mu2A ball of mass m moving at a speed v collides with another ball of mass 3m at rest. The lighter block comes to rest after collisoin. The coefficient of restitution is
A(a) 12B(b) 23C(c) 14D(d) None of theseA ball impinges directly on a similar ball at rest. If 14th of the kinetic energy is lost by the impact, the value of coefficient of restitution is:-
A12√2B1√3C1√2D12
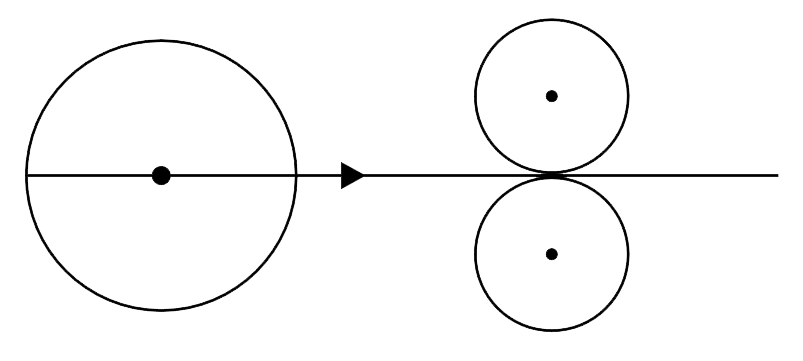
Two identical discs are initially at rest in contact on a horizontal table. A third disc of same mass but of double radius strikes them symmetrically and comes to rest after the impact.
(a) Find the coefficient of restitution for the impact.
(b) Find the minimun kinetic energy of the system (as a percentage of original kinetic energy before collision) during the process of collision.
Treat the collision to be instantaneous
View SolutionTwo equal sphere of mass m are in contact o a smooth horizontal table. A third identical sphere impinges symmetrically on them and is reduced to rest. Find e and the loss KE.

View SolutionTwo equal spheres of mass m are in contact on a smooth horizontal table. A third identical sphere impinges symmetrically on the and is reduced to rest. Prove that e=23 and find the loss in KE.
View SolutionA ball of mass m moving with velocity u strikes another identical ball at rest. Find the velocities of the balls after the collision if the coefficient of restitution is e and the collision is head -on. Also , calculate the loss in K.E.
View SolutionA ball collides with an inclined plane of inclination θ after falling through a distance h. if it moves horizontal just after the impact, the coefficient of restitution is
View Solution
