The probability density function of the random variable X is given by
f(x)={16xe−4xfor x>00for x≤0 find the mean and variance of XSolution in Tamil
The correct Answer is:Mean = 12, Variance = 18
Step by step video, text & image solution for The probability density function of the random variable X is given by f(x)={{:(16xe^(-4x), "for " x gt 0), (0, "for " x le 0):} find the mean and variance of X by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITY DISTRIBUTIONSBOOK - SURA PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONSEXERCISE - EXERCISE 11.5
13 Videos
- PROBABILITY DISTRIBUTIONSBOOK - SURA PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONSEXERCISE - EXERCISE 11.6
19 Videos
- PROBABILITY DISTRIBUTIONSBOOK - SURA PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONSEXERCISE - EXERCISE 11.3
14 Videos
- ORDINARY DIFFERENTIAL EQUATIONSBOOK - SURA PUBLICATIONCHAPTER - ORDINARY DIFFERENTIAL EQUATIONSEXERCISE - ADDITIONAL QUESTIONS
24 Videos
- THEORY OF EQUATIONSBOOK - SURA PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - 4 MARKS
5 Videos
Similar Questions
Compute P(X=k) for the binominal distribution, B(n,p) where
n=6,p=13,k=3
View SolutionLet X be random variable with probability density function
f(x)={2x3x≥10x<1
Which of the following statement is correct
View SolutionThe probability density function of X is given by f(x)={kxe−2xfor x>00for x≤0 Find the value of k.
View SolutionThe order and degree of the differential equation [(d2ydx2)+(dydx)2]12=d3ydx3 are
View SolutionIf the sum of the roots of the quadratic equation ax2+bx+c=0 ( abc ≠ 0) is equal to the sum of the squares of their reciprocals, the sum of the squares of their reciprocals, then ac,ba,cb are in H.P.
View SolutionX is continuous random variable with probability density function f(x)=x28,0≤x≤1. Then, the value of P(0.2≤X≤0.5) is
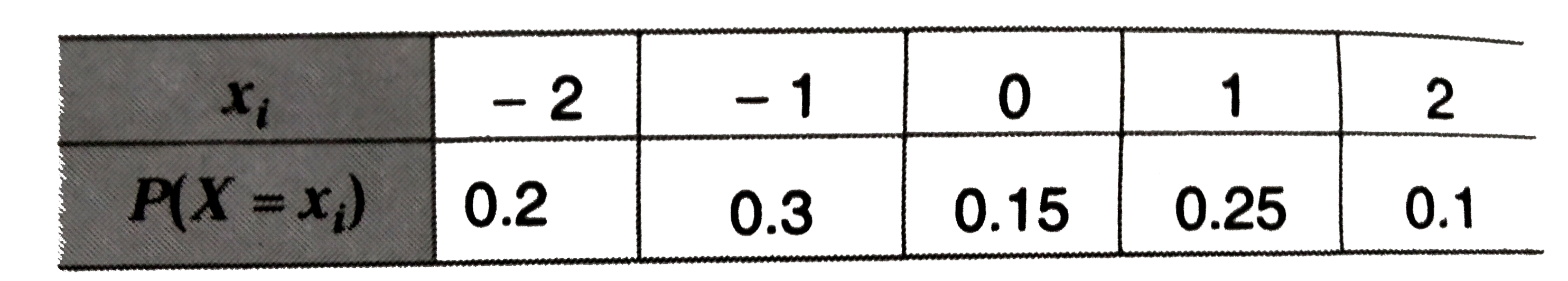
View SolutionIf the probability density function of a random variable X is given as
then F(0) is equal to
View SolutionIf the probability density function of a random variable X is f(x)=x2 in 0≤x≤2, then P(X>1.5∣X>1) is equal to
View SolutionThe probability density function of X is given by f(x)={kxe−2xfor x>00for x≤0 Find the value of k.
View SolutionThe probability density function of X is given by f(x)={ke−x3for x>00for x≤0
Find
the value of k
View SolutionThe probability density function of X is given by f(x)={ke−x3for x>00for x≤0
Find
the distribution function
View SolutionThe probability density function of X is given by f(x)={ke−x3for x>00for x≤0
Find
P(X≤4)
View SolutionIf X is the random variable with probability density function f(x) is given by
f(x)=⎧⎪⎨⎪⎩x+1−1≤x<0−x+10≤x<10otherwise
then find (i) the distribution function F(x) (ii) P(−0.5≤X≤0.5)
View SolutionFor the random variable X with the given probability mass function as below, find the mean and variance.
F(x)={12e−x2for x>00otherwise
View SolutionIf f(x)={2x0≤x≤a0otherwise is a probability density function of a random variable, then the value of a is
View Solution
