A pyramid has a height of 12 centimeters and a square base. If the valume of the pyramid is 256 cubic centimeters, how many centimeters are in the length aof one side of its base?
8
16
32
8√3
The correct Answer is:A
To find the length of one side of the base of the pyramid, we can use the formula for the volume of a pyramid. The volume V of a pyramid is given by the formula:
V=13×Area of base×Height
Given:
- Height h=12 cm
- Volume V=256 cm³
We need to find the area of the base first. Since the base is a square, let the length of one side of the base be s. The area of the base A can be expressed as:
A=s2
Now substituting the known values into the volume formula:
256=13×A×12
Substituting A=s2:
256=13×s2×12
Now, simplify the equation:
256=4s2
Next, we can solve for s2:
s2=2564
Calculating the right-hand side:
s2=64
Now, to find s, we take the square root of both sides:
s=√64
Calculating the square root:
s=8 cm
Thus, the length of one side of the base of the pyramid is 8 centimeters.
- Height h=12 cm
- Volume V=256 cm³
Topper's Solved these Questions
- ADDITIONAL TOPICS IN MATHBook:ENGLISH SATChapter:ADDITIONAL TOPICS IN MATHExercise:Grib-In
Explore 32 Videos
- ADDITIONAL TOPICS IN MATHBook:ENGLISH SATChapter:ADDITIONAL TOPICS IN MATHExercise:EXERCISE
Explore 45 Videos
- ADDITIONAL TOPICS IN MATHBook:ENGLISH SATChapter:ADDITIONAL TOPICS IN MATHExercise:EXERCISE
Explore 45 Videos
- ADDITIONAL TOPICS IN MATH Book:ENGLISH SATChapter:ADDITIONAL TOPICS IN MATH Exercise:PRACTICE TEST
Explore 24 Videos
- COMPLEX NUMBERSBook:ENGLISH SATChapter:COMPLEX NUMBERSExercise:EXERCISE
Explore 6 Videos
Similar Questions
An oil tank has the shape of a right circular cylinder is 12 centimeters in height and has a volume of 108π cubic centimeters. What is the diameter of the base of the cylinder, in centimeters?
View SolutionA rectangular solid has square base, with each side of the base measuring 4 meters. If the volume of the solid is 112 cubic meters, what is the surface area of the solid?
View Solution
Knowledge Check
The vertex angle of an isosceles triangle is 35∘. The length of the base is 10 centimeters. How many centimeters are in the perimeter?
A16.6B17.4C20.2D43.3A rectangular box with an open top is constructed from cardboard to have a square base of area x2 and height h. If the volume of this box is 50 cubic units, how many square units of cardboard in terms of x, are needed to build this box ?
A5x2B6x2C200x+x2D200x+2x2If 10 centimeters of blood contains 1.2 grams of hemoglobin, how many grams of hemoglobin are contained in 35 cubic centimeters of same blood?
A2.7B3.0C3.6D4.2
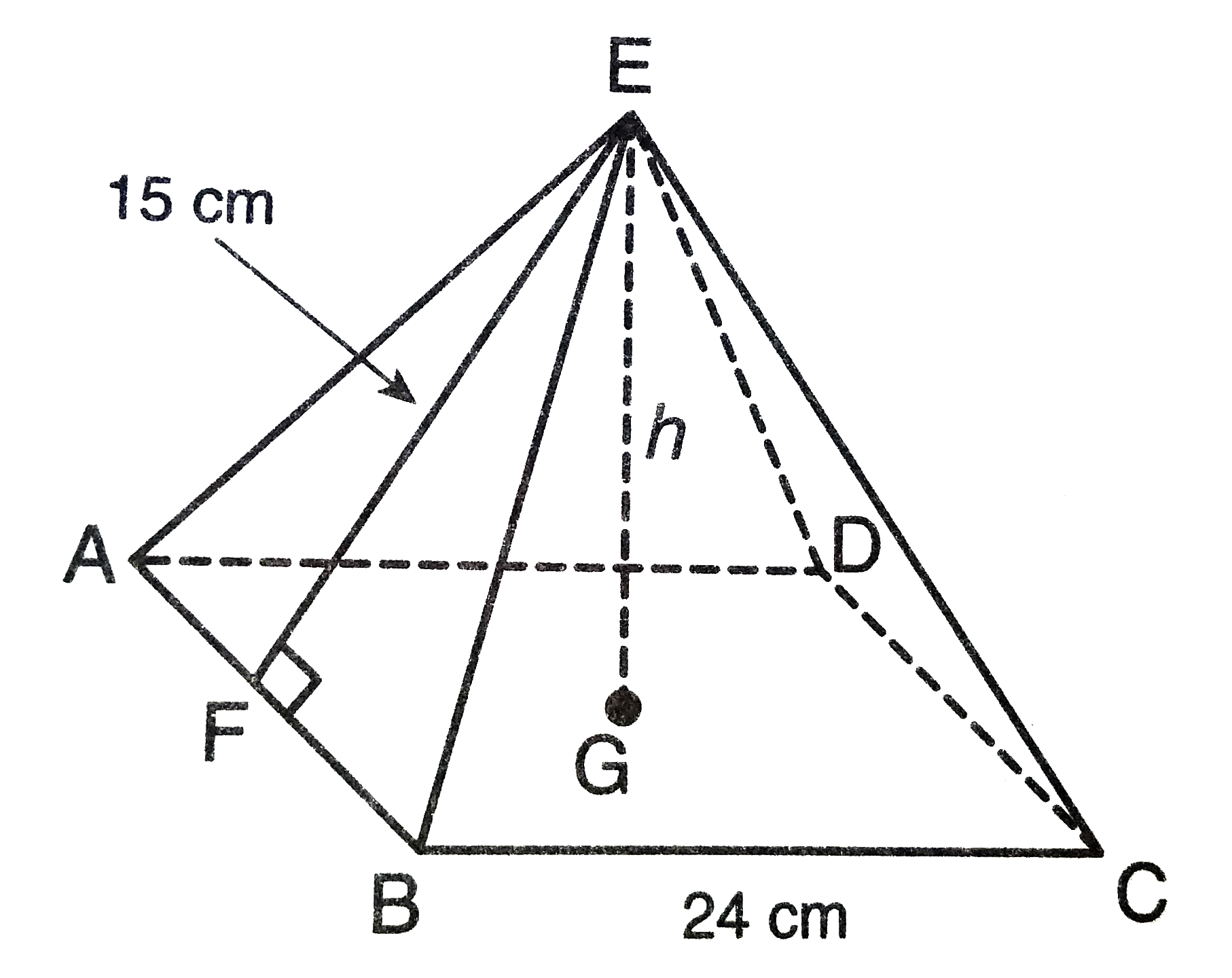
The slant height of a pyramid is the perpendicular distance from the vertex of the pyramid to the base of a figure above, the height is labeled h, the length of a side of the square base is 24 cm, and the slant height is 15 cm, What is the volume , in cubic centimeters, of the pyramid?
View SolutionWhat is the volume, in cubic centimeters, of a right rectangular prism that has a length of 4 centimeters, a width of 9 centimeters, and a height of 10 centimeters?
View SolutionDesiree is making apple juice from concentrate. The cylindrical container of concentrate has a diameter of 7 centimmeters ans a height of 12 centimeters. To make the juice, the concentrate must be diluted with water so that the mix is 75 percent water and 25 percent concentrate.
What is the total volume of juice, rounded to the nearest cubic centimeter, that will be produced if Desiree follows the direction exactly ?
View SolutionIn a square close packing pattern, one atom is in contact with how many atom in the 2D plane base ?
View SolutionJeff tests how the total volume occupied by a fluid contained in a graduated cylinder changes when round marbles of various size are added. He found that the total volume occupied by the fluid, V, in cubic centimeters, can be found using the equation below, where x equals the number of identical marbles Jeff added,one at a time, to the cylinder , and r is the radius of one of the marbles .
V=24π+x(43πr3)
If the volume of the graduated cylinder is 96π cubic centimeters ,then , what is the maximum number of marbles with a radius of 3 centimeters that Jeff can add without the volume of the fluid exceeding that of the graduated cylinder?
View Solution

