A rectangular box with length 22 inches , width 5 inches , and height 5 inches is to be packed with steel balls of radius 2 inches in such a way that the centers of the balls are collinear. What is the maximum number of balls that can fit into the box, provided that no balls should protrude from the box ?
0
5
6
10
The correct Answer is:B
To determine the maximum number of steel balls that can fit into the rectangular box, we will follow these steps:
Step 1: Understand the dimensions of the box and the balls
- The dimensions of the box are:
- Length = 22 inches
- Width = 5 inches
- Height = 5 inches
- The radius of each steel ball is 2 inches.
Step 2: Calculate the diameter of the balls
- The diameter D of each ball is given by the formula:
D=2×radius=2×2=4 inches
Step 3: Determine how many balls can fit along the length of the box
- Since the balls are placed in a collinear manner (in a single line), we will first see how many balls can fit along the length of the box.
- The length of the box is 22 inches.
- Each ball occupies 4 inches (its diameter).
- The number of balls that can fit along the length is calculated by:
Number of balls along length=Length of the boxDiameter of one ball=224=5.5
- Since we cannot have a fraction of a ball, we take the whole number:
Maximum balls along length=5
Step 4: Determine how many balls can fit along the width and height of the box
- The width and height of the box are both 5 inches.
- Each ball occupies 4 inches (its diameter).
- The number of balls that can fit along the width is:
Number of balls along width=Width of the boxDiameter of one ball=54=1.25
- Again, we take the whole number:
Maximum balls along width=1
- The same calculation applies for the height since it is also 5 inches:
Number of balls along height=54=1.25⟹Maximum balls along height=1
Step 5: Calculate the total number of balls that can fit in the box
- The total number of balls that can fit in the box is the product of the maximum number of balls that can fit along the length, width, and height:
Total number of balls=(Balls along length)×(Balls along width)×(Balls along height)=5×1×1=5
Final Answer
The maximum number of steel balls that can fit into the box is 5.
---
- The dimensions of the box are:
- Length = 22 inches
- Width = 5 inches
- Height = 5 inches
- The radius of each steel ball is 2 inches.
- The diameter D of each ball is given by the formula:
D=2×radius=2×2=4 inches
- Since the balls are placed in a collinear manner (in a single line), we will first see how many balls can fit along the length of the box.
- The length of the box is 22 inches.
- Each ball occupies 4 inches (its diameter).
- The number of balls that can fit along the length is calculated by:
Number of balls along length=Length of the boxDiameter of one ball=224=5.5
- Since we cannot have a fraction of a ball, we take the whole number:
Maximum balls along length=5
- The width and height of the box are both 5 inches.
- Each ball occupies 4 inches (its diameter).
- The number of balls that can fit along the width is:
Number of balls along width=Width of the boxDiameter of one ball=54=1.25
- Again, we take the whole number:
Maximum balls along width=1
Number of balls along height=54=1.25⟹Maximum balls along height=1
- The total number of balls that can fit in the box is the product of the maximum number of balls that can fit along the length, width, and height:
Total number of balls=(Balls along length)×(Balls along width)×(Balls along height)=5×1×1=5
The maximum number of steel balls that can fit into the box is 5.
Topper's Solved these Questions
Similar Questions
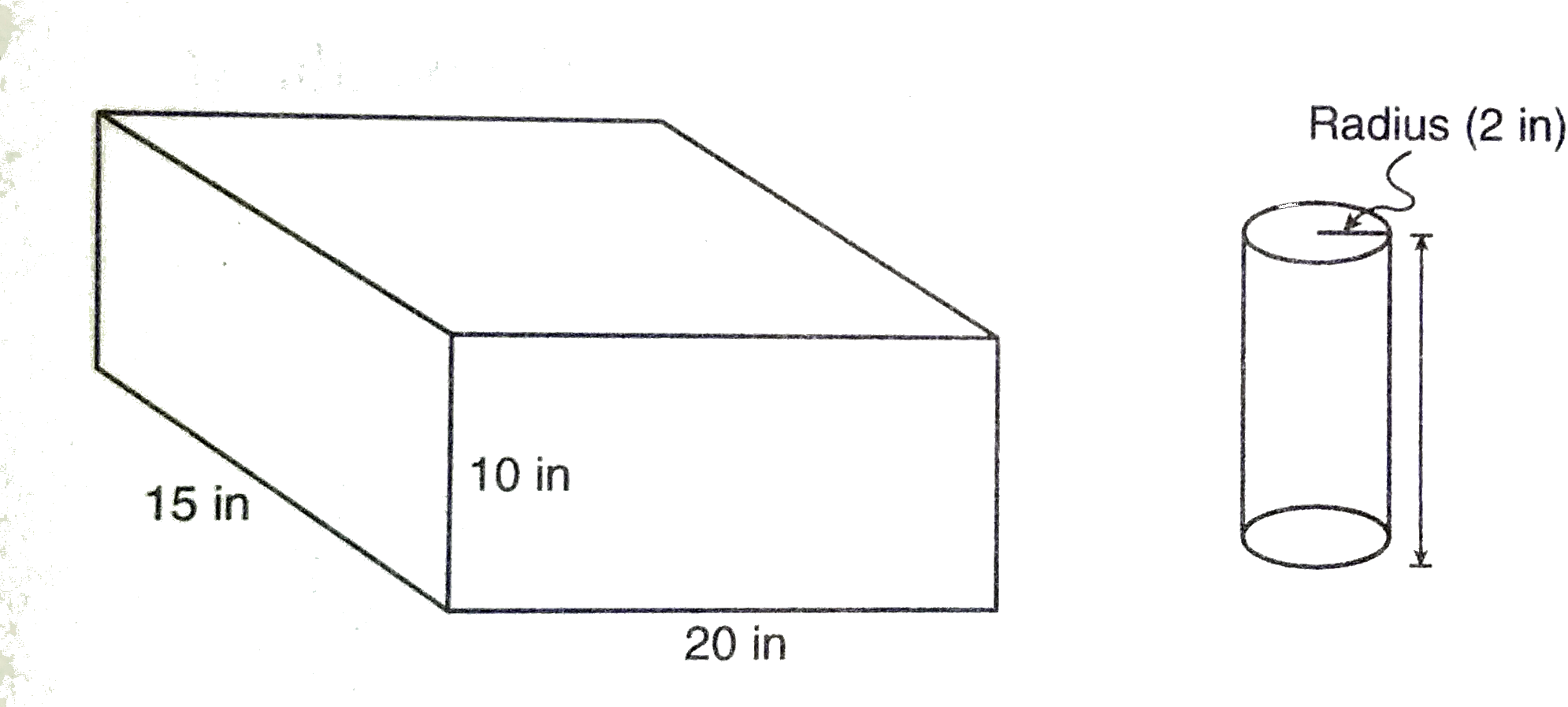
In the figure above, a rectangular container with the dimensions 10 inches by 15 inches by 20 inches is to be filled with water, using a cylindrical cup whose radius is 2 inches and whose height is 5 inches. What is the maximum number of full cups of water that can be placed into the container without the water overflowing the container?
View SolutionThe number of ways in which n distinct balls can be put into three boxes, is
View Solution
Knowledge Check
A company ships notepads in rectangular boxes that each have inside dimensions measuring 9 inches long, 9 inches wide, and 12 inches tall. Each notepad is in the shape of a cube with an edge length of 3 inches. What is the maximum number of natepads that will fit in 1 closed box?
A10B11C12D36Sophie has a hard rubber ball whose circumference measures 13 inches. She wants to store it in a box. What is the number of cubic inches in the volume of the smallest cube shaped box with integer dimensions that she can use?
A64B81C125D216
Find the total number of ways in which 20 balls can be put into 5 boxes so that first box contains just one ball.
View SolutionThere are 5 different colour balls and 5 boxes of colours same as those of the balls. The number of ways in which one can place the balls into the boxes, one each in a box, so that no ball goes to a box of its own colour is
View SolutionA box contains 7 red balls, 8 green balls and 5 white balls. A ball is drawn at random from the box. Find the probability that the ball is white.
View SolutionIn how many ways 5 different balls can be distributed into 3 boxes so that no box remains empty?
View SolutionThe number of ways in which 9 identical balls can be placed in three identical boxes is
View SolutionFind the number of ways in which 4 distinct balls can be put into 3 distinct boxes so that no remains empty
View Solution
