A stone is thrown horizontally with the velocity vx=15m/s. Determine the normal and tangential accelerations of the stone in I second after begins to move.
To solve the problem of determining the normal and tangential accelerations of a stone thrown horizontally with a velocity of vx=15m/s after 1 second, we can follow these steps:
Step 1: Identify the Components of Motion
The stone is thrown horizontally, which means its initial vertical velocity (vy0) is 0 m/s. The only acceleration acting on the stone is due to gravity, which acts downward.
Step 2: Calculate the Vertical Velocity After 1 Second
Using the formula for vertical velocity under constant acceleration:
vy=vy0+g⋅t
where:
- vy0=0m/s (initial vertical velocity)
- g=10m/s2 (acceleration due to gravity)
- t=1s
Substituting the values:
vy=0+10⋅1=10m/s
Step 3: Determine the Resultant Velocity
After 1 second, the stone has a horizontal velocity (vx) of 15 m/s and a vertical velocity (vy) of 10 m/s. The resultant velocity (v) can be calculated using the Pythagorean theorem:
v=√v2x+v2y=√(15)2+(10)2=√225+100=√325=5√13m/s
Step 4: Calculate the Angle of the Velocity Vector
The angle θ of the resultant velocity with respect to the horizontal can be found using:
tanθ=vyvx=1015=23
Step 5: Determine the Normal and Tangential Accelerations
The normal and tangential accelerations can be derived from the gravitational acceleration g and the angle θ:
- Normal acceleration (an):
an=g⋅cosθ
- Tangential acceleration (at):
at=g⋅sinθ
Using the values:
- cosθ=3√13
- sinθ=2√13
Substituting these into the equations:
an=10⋅3√13=30√13m/s2
at=10⋅2√13=20√13m/s2
Final Answer
Thus, the normal and tangential accelerations of the stone after 1 second are:
- Normal acceleration: 30√13m/s2
- Tangential acceleration: 20√13m/s2
The stone is thrown horizontally, which means its initial vertical velocity (vy0) is 0 m/s. The only acceleration acting on the stone is due to gravity, which acts downward.
Using the formula for vertical velocity under constant acceleration:
vy=vy0+g⋅t
where:
- vy0=0m/s (initial vertical velocity)
- g=10m/s2 (acceleration due to gravity)
- t=1s
vy=0+10⋅1=10m/s
After 1 second, the stone has a horizontal velocity (vx) of 15 m/s and a vertical velocity (vy) of 10 m/s. The resultant velocity (v) can be calculated using the Pythagorean theorem:
v=√v2x+v2y=√(15)2+(10)2=√225+100=√325=5√13m/s
The angle θ of the resultant velocity with respect to the horizontal can be found using:
tanθ=vyvx=1015=23
The normal and tangential accelerations can be derived from the gravitational acceleration g and the angle θ:
- Normal acceleration (an):
an=g⋅cosθ
- Tangential acceleration (at):
at=g⋅sinθ
- cosθ=3√13
- sinθ=2√13
an=10⋅3√13=30√13m/s2
at=10⋅2√13=20√13m/s2
Thus, the normal and tangential accelerations of the stone after 1 second are:
- Normal acceleration: 30√13m/s2
- Tangential acceleration: 20√13m/s2
Topper's Solved these Questions
- FORCE ANALYSISBOOK - ANURAG MISHRACHAPTER - FORCE ANALYSISEXERCISE - level 1
103 Videos
- FORCE ANALYSISBOOK - ANURAG MISHRACHAPTER - FORCE ANALYSISEXERCISE - Level-2
73 Videos
- FORCE ANALYSISBOOK - ANURAG MISHRACHAPTER - FORCE ANALYSISEXERCISE - Matching type
13 Videos
- DESCRIPTION OF MOTIONBOOK - ANURAG MISHRACHAPTER - DESCRIPTION OF MOTIONEXERCISE - Level-3
34 Videos
- IMPULSE AND MOMENTUMBOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - matching
3 Videos
Similar Questions
A stone is thrown in a vertically upward direction with a velocity of 5ms-1.If the acceleration of the stone during its motion is 10ms-2 in the downward direction,what will be the height attained by the stone and how much time will it take to reach there?
View SolutionA boy whirls a stone of small mass in a horizontal circle of radius 1.5m and at height 2.9m above level ground. The string breaks and the stone flies off horizontally and strikes the ground after travelling a horizontal distance of 10m . What is the magnitude of the centripetal acceleration of the stone while in circular motion ?
View Solution
Knowledge Check
A stone is thrown horizontally with velocity u. The velocity of the stone 0.5 s later is 3u/2. The value of u is
A2.2ms−1B3.3ms−1C4.4ms−1D1.1ms−1A stone is thrown horizontally with a velocity of 10m/sec. Find the radius of curvature of it’s trajectory at the end of 3s after motion began. (g=10m/s2)
A10√10mB100√10mC√10mD100mA stone is thrown horizontally with a velocity of 10m/s at h=0. The radius of curvature of the stone's trajectory at t=3s is : [Take g=10m/s2]
A10√10mB100mC100√10mD1000m
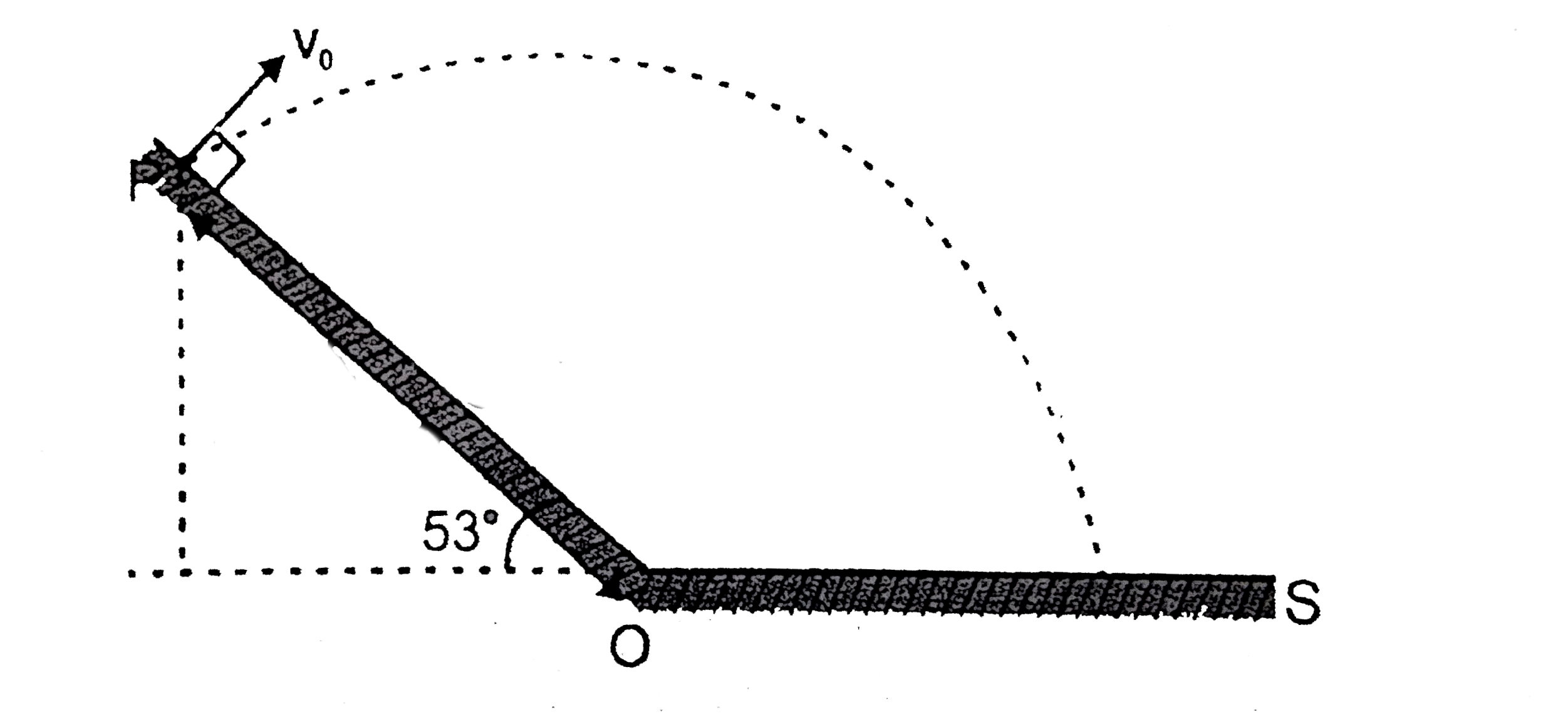
A stone is projected from point P on the inclined plane with velocity v0=10m/sec directed perpendicular to the plane. The time taken (in second) by the stone to strike the horizontal ground S is (Given PO=l=10meter)(Take g=10m/s2)
View SolutionA body is thrown horizontally with a velocity u from the top of a tower.The displacement of the stone when the horizontal and vertical velocities are equal is
View SolutionA stone is thrown in a vertically upward direction with a velocity of 5m/s. If the acceleration of the stone during its motion is 10m/s2 in the downward direction, what will be the height attained by the stone and how much time will take to reach there ?
View SolutionTwo stones are thrown vertically upwards with the same velocity of 49m/s. If they are thrown one after the other with a time lapse of 3 second, height at which they collide is
View SolutionWhen we release a stone from a balloon which ascends with a velocity v and acceleration a, the velocity and acceleration of the stone just after release are :
View Solution
