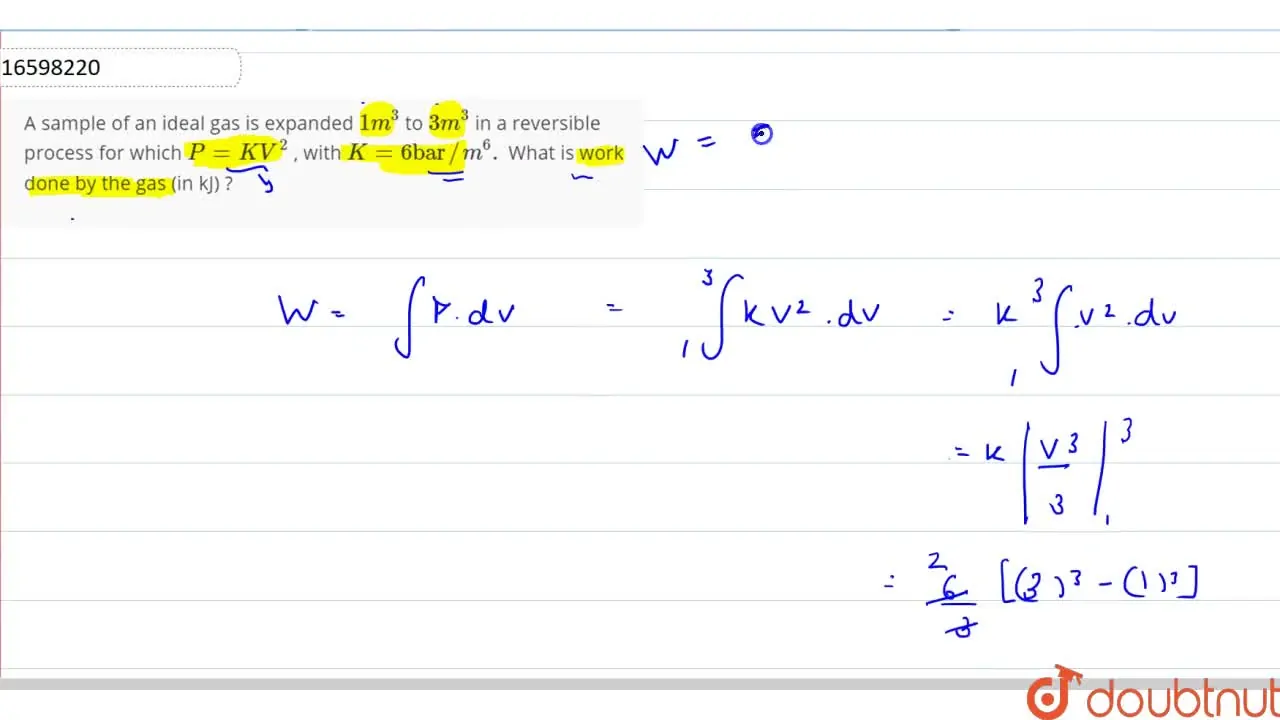
A sample of an ideal gas is expanded to in a reversible process for which , with What is work done by the gas (in kJ) ?
The correct Answer is:5200
Step by step video, text & image solution for A sample of an ideal gas is expanded 1 m^(3) to 3 m^(3) in a reversible process for which P=KV^(2) , with K=6 "bar"//m^(6). What is work done by the gas (in kJ) ? by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
A sample of an ideal gas is expanded from 1dm3 to 3 in a reversible process for which P=KV3, with K=1/5(atm/dm3), what is work done by gas (Latm)
View SolutionA sample of an ideal gas is expanded to twice its original volume of 1m3 in a reversible process for which P=αV2, where α=5atmm−6. If CV,m=20Jmol−1K−1, determine moalr change in entropy (ΔSm) for the process.
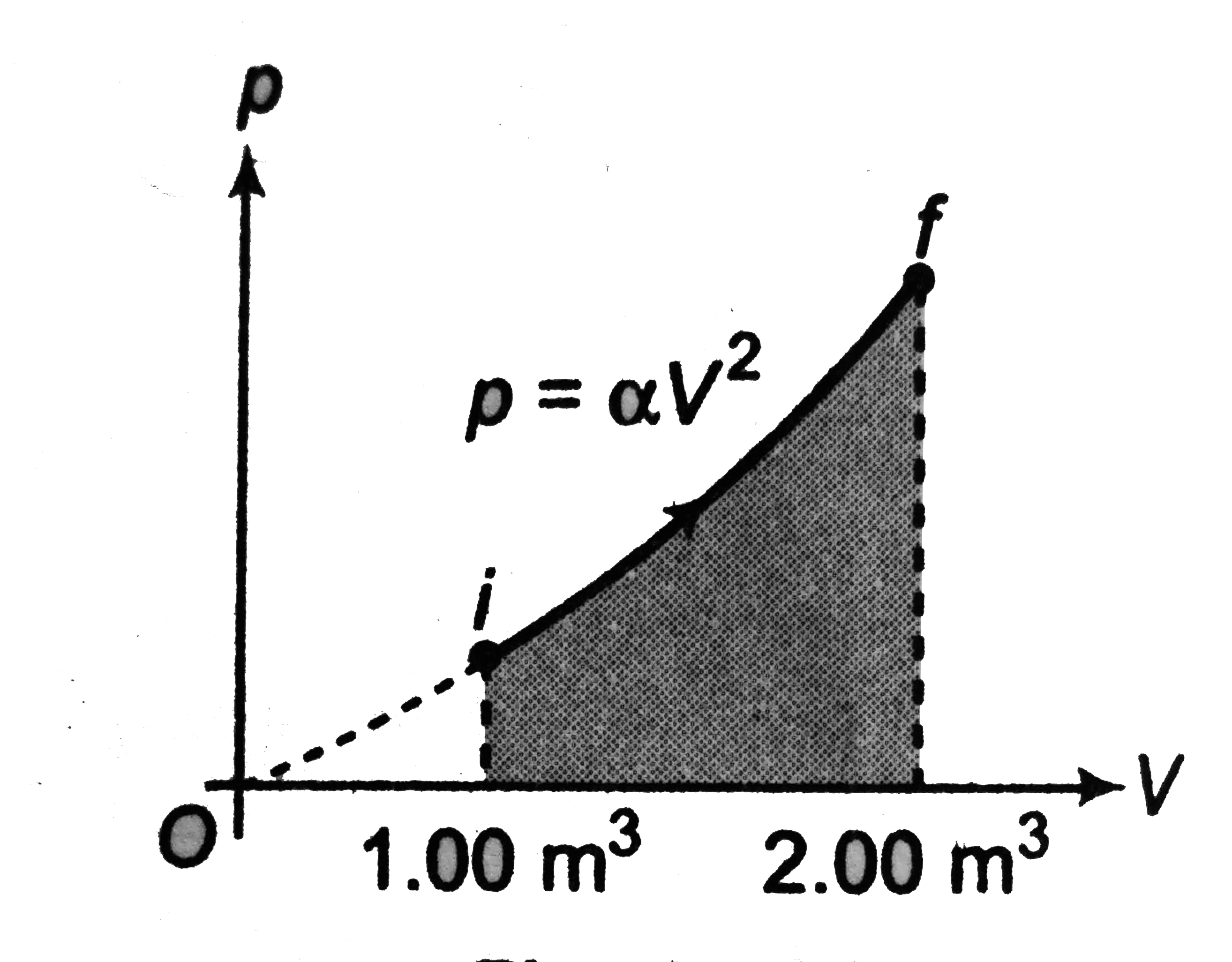
View SolutionA sample of ideal gas is expanded to twice its original volume of 1.00m3 in a quasi-static process for which p=αV2, with α=5.00atm/m6, as shown in Fig. How much work is done by the expanding gas?
 View Solution
View SolutionA sample of ideal gas is expanded to twice of its original volume of 1m^(3) in a quasi-static process for which P=alpha v^(2),alpha=3*10^(5)Pa/m^(6)as shown in fig. Work done by expanding gas is
View SolutionA gas expands from a volume of 1m3 to a volume of 2m3 against an external pressure of 105Nm−2. The work done of the gas will be
View SolutionA gas expands from a volume of 1m3 to a volume of 2m3 against an external pressure of 105Nm−2. The work done of the gas will be
View Solution2 moles of an ideal gas is expanded isothermally from 2.5×10−3m3 to 8×10−3m3 against a constant external pressure of 5×105Nm−2. The work done in the process is ________ .
View Solution1 moles of an ideal gas expanded spontaneously into vaccum. The work done will be
View Solution1 mole of an ideal gas expands from 5dm3 to25dm3 isothermally and irreversibly at 27∘ C. Find work done in the process [R=8.3 J/mol/K]
View Solution6 moles of an ideal gas expand isothermally and reversibly from a volume of 1 litre to a volume of 10 litres at 27∘C. What is the maximum work done.
View Solution