Two positively charged spheres of masses m1, and m2 are suspended from a common point at the ceiling by identical insulating massless strings of length l. Charged on the two spheres are q1 and q2, respectively. At equilibrium both strings make the same angle θ with the vertical. Then
q1m1=q2m2
m1=m2
m1=m2sinθ
q2m1=q1m2
The correct Answer is:B
To solve the problem, we will analyze the forces acting on each of the charged spheres and use the equilibrium conditions to derive the required relationships.
1. Identify Forces Acting on Each Sphere:
Each sphere experiences three forces:
- The gravitational force acting downward: Fg=mig (where i=1,2 for spheres 1 and 2 respectively).
- The tension in the string acting along the string: Ti.
- The electrostatic force of repulsion between the two charged spheres: Fe=kq1q2r2, where r is the distance between the two spheres.
2. Geometry of the Setup:
Since both strings make the same angle θ with the vertical, we can express the horizontal distance between the two spheres in terms of L and θ:
r=2Lsin(θ)
3. Equilibrium Conditions:
For each sphere to be in equilibrium, the net force in both the vertical and horizontal directions must be zero.
- Vertical Forces:
For sphere 1:
T1cos(θ)=m1g
For sphere 2:
T2cos(θ)=m2g
- Horizontal Forces:
The horizontal component of the tension must balance the electrostatic force:
For sphere 1:
T1sin(θ)=Fe=kq1q2(2Lsin(θ))2
For sphere 2 (similar equation):
T2sin(θ)=Fe=kq1q2(2Lsin(θ))2
4. Express Tensions:
From the vertical force equations, we can express the tensions:
T1=m1gcos(θ)
T2=m2gcos(θ)
5. Substituting Tensions into Horizontal Force Equations:
Substitute T1 and T2 into the horizontal force equations:
m1gcos(θ)sin(θ)=kq1q2(2Lsin(θ))2
m2gcos(θ)sin(θ)=kq1q2(2Lsin(θ))2
6. Equating Forces:
Since both equations equal kq1q2(2Lsin(θ))2, we can set them equal to each other:
m1gsin(θ)cos(θ)=m2gsin(θ)cos(θ)
7. Final Relationship:
This leads to the conclusion:
m1=m2
Each sphere experiences three forces:
- The gravitational force acting downward: Fg=mig (where i=1,2 for spheres 1 and 2 respectively).
- The tension in the string acting along the string: Ti.
- The electrostatic force of repulsion between the two charged spheres: Fe=kq1q2r2, where r is the distance between the two spheres.
Since both strings make the same angle θ with the vertical, we can express the horizontal distance between the two spheres in terms of L and θ:
r=2Lsin(θ)
For each sphere to be in equilibrium, the net force in both the vertical and horizontal directions must be zero.
For sphere 1:
T1cos(θ)=m1g
For sphere 2:
T2cos(θ)=m2g
The horizontal component of the tension must balance the electrostatic force:
For sphere 1:
T1sin(θ)=Fe=kq1q2(2Lsin(θ))2
For sphere 2 (similar equation):
T2sin(θ)=Fe=kq1q2(2Lsin(θ))2
From the vertical force equations, we can express the tensions:
T1=m1gcos(θ)
T2=m2gcos(θ)
Substitute T1 and T2 into the horizontal force equations:
m1gcos(θ)sin(θ)=kq1q2(2Lsin(θ))2
m2gcos(θ)sin(θ)=kq1q2(2Lsin(θ))2
Since both equations equal kq1q2(2Lsin(θ))2, we can set them equal to each other:
m1gsin(θ)cos(θ)=m2gsin(θ)cos(θ)
This leads to the conclusion:
m1=m2
Topper's Solved these Questions
Similar Questions
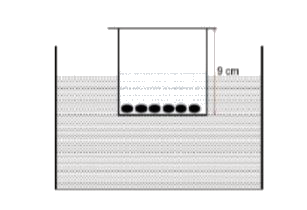
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
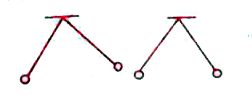
View SolutionTwo identical small charged spheres, each having a mass m, hang in equilibrium as shown in fig. the ligth of each string is l, and the angle made by any string with the vertical is theta . Find the magnitude of the charge on each sphere.
View SolutionTwo small spheres of masses M_(1) and M_(2) are suspended by weightless insulating threads of lengths L_(1) and L_(2) . The speres carry charges Q_(1) and Q_(2) respectively. The spheres are suspended such that they are in level with one another adn the threads are inclined to the verticle at angles theta_(1) and theta_(2) respectively . Which one of the following conditions is essential for theta_(1) = theta_(2) ?
View SolutionTwo positively charged sphere of masses m_(1) and m_(2) are suspended from a common point at the ceiling by identical insulating massless strings of length l . Charges on the two spheres are q_(1) and q_(2) respectively. Equilibrium both strings make the same angle theta with the vertical. then
View SolutionTwo small spheres with mass m_(1) and m_(2) hang from massless insulating threads of length l_(1) and l_(2) . The two spheres carry charges q_(1) and q_(2) respectively. The spheres are hung such that they are on the same horizontal level and the threads are inclined to the vertical at angles theta_(1) and theta_(1) . Which of the condition is required if theta_(1)=theta_(2) ?
View SolutionTwo small metal balls of different mass m_(1) and m_(2) are connected by strings of equal length to a fixed point. When the balls are given equal charges, the angles that the two strings make with the vertical are 30^(@) and 60^(@) , respectively. The ratio m_(1)//m_(2) is close to -
View SolutionTwo identical spheres of mass m and charge q are suspended from a common point by two threads of the same length l and it is found that threads make an angle theta with the vertical when the spheres are in equilibrium . Calculate the value of q.
View SolutionTwo small spheres of masses M_(1) and M_(2) are suspended by weightless insulating threads of lengths L_(1) and L_(2) The spheres carry charges Q_(1) and Q_(2) respectively. The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of theta_(1) and theta_(2) as shown. Which one of the following conditions is essential, if theta_(1)=theta_(2) ?
View Solutionदो समरूप चालक गेंद A व B पर क्रमशः धनात्मक आवेश q1वq2 है | किन्तु q1≠q2 है | दोनों बॉल को परस्पर स्पर्श किया जाता है तथा फिर उन्हें पूर्व स्थिति में ही रख दिया जाता हैं | आवेशों के मध्य बल होगा
View SolutionTwo metal sphers of masses m1andm2 are suspended from a common point by a light insulting strings of same length. The length of each string is same. The spheres are given positive charges q1andq2. Figure A shows angles made by the strings with vertical are different where as for figure B same. Then, which of the following is possible
View Solutionm1 तथा m2 द्रव्यमान के दो धनात्मक आवेशित गोले l लम्बाई की एक समान कुचालक द्रव्यमानरहित डोरी की सहायता से छत के एक उभयनिष्ठ बिन्दू से लटके हुऐं है। दोनो गोलो पर आवेश क्रमशः q1 तथा q2 है। साम्य अवस्था पर दोनो डोरियाँ उर्ध्वाधर से समान कोण θ बनाती है तब :
View SolutionTwo small spheres of masses M_(1) and M_(2) are suspended by weightless insulating threads of lengths L_(1) and L_(2) . The spheres carry charges Q_(1) and Q_(2) respectively. The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles theta_(1) and theta_(2) respectively . Which one of the following conditions is essential for theta_(1) = theta_(2) ?
View SolutionTwo identical small charged spheres, each having a mass m, hang in equilibrium the length of each string is l, and the angle made by any string with the vertical is theta . Find the magnitude of the charge on each sphere.
View SolutionTwo spheres, each having mass m are suspended from a common point by two insulating strings of length l. The spheres are identically charged and the separation between the spheres is 'd' . Find the charge on each sphere.
View SolutionTwo positively charged sphere of masses m_(1) and m_(2) are suspended from a common point at the ceiling by identical insulating massless strings of length l . Charges on the two spheres are q_(1) and q_(2) respectively. Equilibrium both strings make the same angle theta with the vertical. then
View Solution
