A standing wave in a pipe with a length L=1.2m is described by
y(x,t)=y0sin[(2π/L)x]sin[(2π/L)x+π/4]
Based on above information, which one of the following statements is incorrect. (Speed of sound in air is 300ms−1)–
The pipe is closed at both ends
The wavelength of the wave could be 1.2 m
There could be a node at x = 0 and antinode at x=L/2
The frequency of the fundamental mode of vibrations is 137.5 Hz
The correct Answer is:D
Step by step video, text & image solution for A standing wave in a pipe with a length L = 1.2 m is described by y(x,t) = y_(0)sin[(2pi//L)x]sin[(2pi//L)x+pi//4] Based on above information, which one of the following statements is incorrect. (Speed of sound in air is 300 ms^(-1))– by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
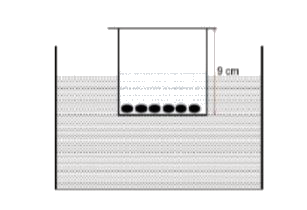
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
View SolutionThe ends of a stretched wire of length L are fixed at x = 0 and x = L . In one experiment, the displacement of the wire is y_(1) = A sin(pi//L) sin omegat and energy is E_(1) and in another experiment its displacement is y_(2) = A sin (2pix//L ) sin 2omegat and energy is E_(2) . Then
View SolutionAn open organ pipe of length l is sounded together with another open organ pipe of length l + x in their fundamental tones. Speed of sound in air is v . The beat frequency heard will be (x lt lt l) :
View SolutionAn open organ pipe of length l is sounded together with another open organ pipe of length l + x in their fundamental tones. Speed of sound in air is v. the beat frequency heard will be ( x lt lt l )
View SolutionIf two sound waves, y1=0.3sin596π[t−x/300] andy2=0.5sin604π[t−x/330] are superimposed, what will be the
(a) frequencyof resultant wave
View SolutionIf two sound waves, y1=0.3sin596π[t−x/300] and y2=0.5sin604π[t−x/330] are superimposed, what will be the
(b) frequencyat which beats are produced.
View SolutionThe wave described by y =0.25sin(10πx−2πnt) where x and y are in meters and t in seconds , is a wave travelling along the
View SolutionAn open pipe of length l is sounded together with another open organ pipe of length l + x in their fundamental tones. Speed of sound in air is V. The beat frequency heard will be (x << l).
View Solutionप्रगामी तरंग y=4sin2π(t0.02−x100) जहाँ y तथा x से.मी. में तथा t सैकण्ड में है। इन तरंग के लिए असत्य कथन है।
View SolutionThe ends of a stretched wire of length 'L' are fixed at x = 0 and x = L. In one experiment, the displacement of the wire is y_(1) = A "sin"(pi x)/(L) sin wt and the energy is E_(1) and in another experiment its displacement is y_(2)=A "sin" (2pi x)/(L) sin 2 wt and the energy E_(2) then
View SolutionConsider a situtation (i) two sound waves y_(1) = 0.2 sin 504 pi (t-x/300) and y_(2) = 0.6 sin 490 pi (t-x/300) are superimposed Consider another situation: (ii) two sound waves y_(1) = 0.2 sin 504 pi(t-x/300) and y_(2) = 0.4 sin 500 pi (t + x/300) are superimposed. Match the Column-I with Column -II
View SolutionAn open organ pipe of length l is sounded together with another open organ pipe of length l + x in their fundamental tones. Speed of sound in air is v. the beat frequency heard will be ( x lt lt l )
View SolutionA transverse wave is described by the equatiion Y = Y_(0) sin 2pi (ft -x//lambda) . The maximum particle velocity is equal to four times the wave velocity if lambda = pi Y_(0) //4 lambda = pi Y_(0)//2 lambda = pi Y_(0) lambda = 2pi Y_(0)
View SolutionThe ends of a stretched wire of length L are fixed at x = 0 and x = L, In one experiment, the displacement of wire is y1=Asin(πx/L)sinωt and energy is E1 and in another experiment its displacement is y2=Asin(2πx/L)sin2ωt and energy is E2. Then
View SolutionFind the phase difference betwee the two progressive wave. y1=Asin(2πTt−2πλx)andy2=Acos(2πTt−2πλx)
View Solution
