Draw a pair of radii OA and OB such that ∠BOA=120∘ . Draw the bisector of ∠BOA and draw lines perpendiculars to OA and OB at A and B . These lines meet on the bisector of ∠BOA at a point which is the external point and the perpendicular lines are the required tangents . Construct and justify . Solution in Telugu
The correct Answer is:PA=PB
Step by step video, text & image solution for Draw a pair of radii OA and OB such that angleBOA=120^(@) . Draw the bisector of angleBOA and draw lines perpendiculars to OA and OB at A and B . These lines meet on the bisector of angleBOA at a point which is the external point and the perpendicular lines are the required tangents . Construct and justify . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TANGENT AND SECANTS TO A CIRCLE BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - TANGENT AND SECANTS TO A CIRCLE EXERCISE - EXERCISE 9 .1 (Fill in the blanks )
5 Videos
- TANGENT AND SECANTS TO A CIRCLE BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - TANGENT AND SECANTS TO A CIRCLE EXERCISE - EXERCISE 9 .1
4 Videos
- TANGENT AND SECANTS TO A CIRCLE BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - TANGENT AND SECANTS TO A CIRCLE EXERCISE - DO THIS
14 Videos
- SUMMATIVE ASSESSMENTBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - SUMMATIVE ASSESSMENTEXERCISE - SUMMATIVE ASSESSMENT
61 Videos
- TANGENTS AND SECANTS TO A CIRCLEBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - TANGENTS AND SECANTS TO A CIRCLEEXERCISE - EXERCISE
201 Videos
Similar Questions
A tangent to a circle intersects it in ………. Point (s) .
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q so that OQ = 12cm . Find length of PQ .
View SolutionDraw a circle with any radius . Draw four tangents at different points . How many tangents can you draw to this circle ?
View SolutionRamu says, "If log10x=0, value of x=0". Do you agree with him ? Give reason.
View SolutionDraw a circle with any radius . Draw four tangents at different points . How many tangents can you draw to this circle ?
View Solution6.0 सेमी लम्बाई का एक रेखाखण्ड खींचिए और इसके लम्ब समद्विभाजक की रचना कीजिए।
View SolutionOA and OB are two perpendicular straight lines. A straight line AB is drawn in such a manner that OA+OB=8. Find the locus of the mid point of AB.
View Solution/_BOA=120^(0) Draw the radii of OA and OB, respectively. /_BOA Draw an isosceles line to and draw perpendiculars to OA and OB at A and B. These lines /_BOA Intersect the line of intersection at the outer point. These can be taken as the tangents we want. Build. Justify.
View SolutionDraw a pair of radii OA and OB in a circle such that ∠BOA=120∘. Draw the bisector of ∠BOA and draw lines perpendiculars to OA and OB at A and B. These lines meet on the bisector of ∠BOA at a point which is the external point and the perpendicular lines are the required tangents. Construct and Justify.
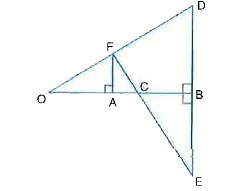
View SolutionIn the Fig. given below, OB is the perpendicular bisector of the line segment DE, FA⊥OB and FE intersects OB at the point C. Prove that 1OA+1OB=2OC
View SolutionDraw a pair of radii OA and OB in a circle such that ∠BOA=120∘. Draw the bisector of ∠BOA and draw lines perpendiculars to OA and OB at A and B. These lines meet on the bisector of ∠BOA at a point which is the external point and the perpendicular lines are the required tangents. Construct and Justify.
View SolutionIn figure 6.13, OB is the perpendicular bisector of the line segment DE, FA _|_ OB And FE intersects line segment OB at point C. Prove that (1)/(OA)+(1)/(OB)=(2)/(OC) is.
View Solution−−→OAand−−→BO are two vectors of magnitudes 5 and 6 respectively. If ∠BOA=60∘,then −−→OA.−−→OB is equal to
View Solution5.6 सेमी लम्बाई का एक रेखाखंड खींचिए और इसके लंब समद्विभाजक की रचना कीजिए।
View SolutionDraw an angle with vertex O. Consider point A on one of its sides and B on another side such that OA = OB. overset_(OA And overset_(OB Draw the perpendicular bisector of. Suppose they intersect at P. What is PA = PB?
View Solution
