Question

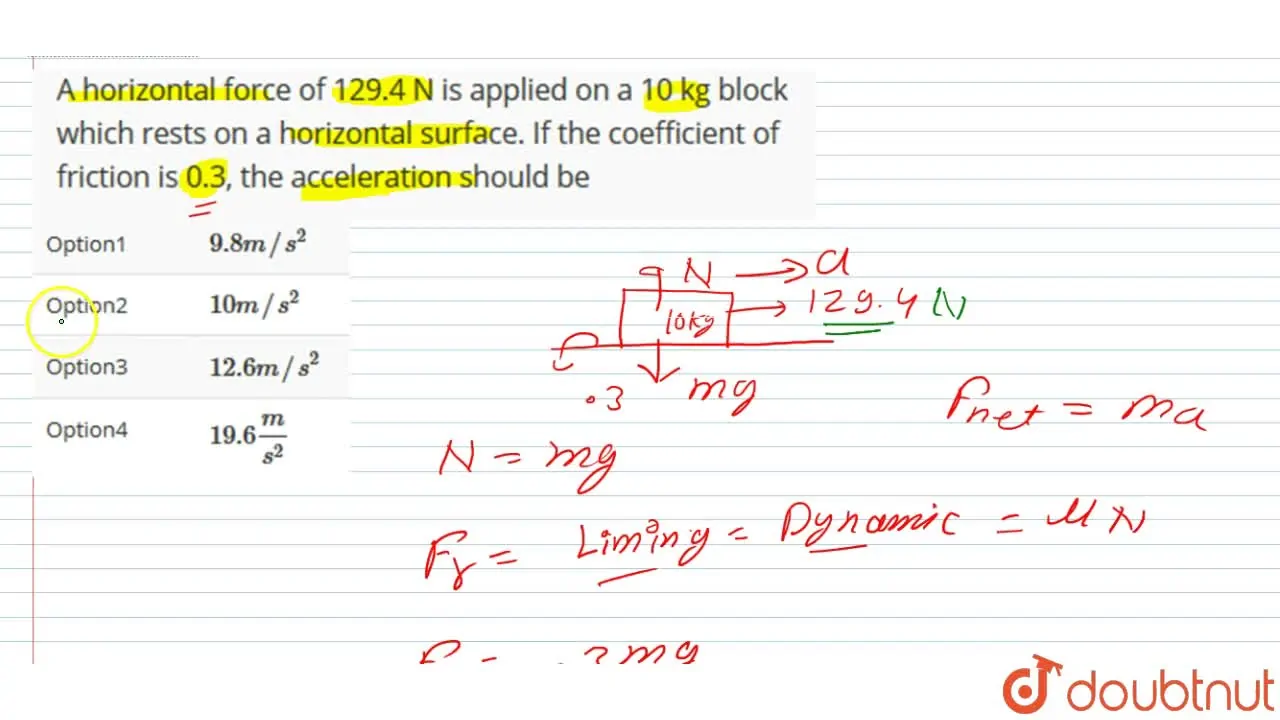
A horizontal force of 129.4 N is applied on a 10 kg block which rests on a horizontal surface. If the coefficient of friction is 0.3, the acceleration should be

Options

A. `9.8m//s^(2)`
B. `10m//s^(2)`
C. `12.6m//s^(2)`
D. `19.6m/s^(2)`

Answer

B

Explanation

To find the acceleration of the block, we can follow these steps:

Step 1: Identify the given values
- Applied force (F) = 129.4 N
- Mass of the block (m) = 10 kg
- Coefficient of friction (μ) = 0.3
- Acceleration due to gravity (g) = 9.8 m/s² (standard value)

Step 2: Calculate the normal force (N)
Since the block is resting on a horizontal surface, the normal force is equal to the weight of the block.
\( N = m \cdot g \)
\( N = 10 \, \text{kg} \cdot 9.8 \, \text{m/s}^2 = 98 \, \text{N} \)

Step 3: Calculate the frictional force (F_friction)
The frictional force can be calculated using the coefficient of friction and the normal force:
\( F_{\text{friction}} = \mu \cdot N \)
\( F_{\text{friction}} = 0.3 \cdot 98 \, \text{N} = 29.4 \, \text{N} \)

Step 4: Apply Newton's second law
According to Newton's second law, the net force acting on the block is equal to the mass of the block multiplied by its acceleration (F_net = m * a).
The net force can be calculated as:
\( F_{\text{net}} = F - F_{\text{friction}} \)
\( F_{\text{net}} = 129.4 \, \text{N} - 29.4 \, \text{N} = 100 \, \text{N} \)

Step 5: Calculate the acceleration (a)
Now we can find the acceleration using the net force:
\( F_{\text{net}} = m \cdot a \)
Rearranging gives:
\( a = \frac{F_{\text{net}}}{m} \)
Substituting the values:
\( a = \frac{100 \, \text{N}}{10 \, \text{kg}} = 10 \, \text{m/s}^2 \)

Final Answer
The acceleration of the block is 10 m/s².

---