A wide tank of cross-section area A, containing a liquid to a height H, has an orifice at its base of areaA3 . The initial velocity of top surface of liquid is
√gH4
3√gH4
√gH2
13√gH2
The correct Answer is:A
To solve the problem, we will use Bernoulli's equation and the principle of continuity. Here’s the step-by-step solution:
Step 1: Understand the Setup
We have a tank with a cross-sectional area A filled with liquid to a height H. At the bottom of the tank, there is an orifice with a cross-sectional area of A3.
Step 2: Apply Bernoulli’s Equation
We will apply Bernoulli’s equation between two points:
1. At the top surface of the liquid (Point 1).
2. At the orifice (Point 2).
The Bernoulli's equation states:
P1+ρgH+12ρv21=P2+ρgh2+12ρv22
Where:
- P1 and P2 are the pressures at points 1 and 2 respectively.
- ρ is the density of the liquid.
- g is the acceleration due to gravity.
- H is the height of the liquid column.
- v1 is the velocity of the liquid at the top surface.
- v2 is the velocity of the liquid exiting the orifice.
Step 3: Define Pressures and Velocities
At the top surface of the liquid (Point 1):
- The pressure P1=P0 (atmospheric pressure).
- The height H is the height of the liquid column.
- The velocity v1 is what we want to find.
At the orifice (Point 2):
- The pressure P2=P0 (atmospheric pressure).
- The height h2=0 (since it is at the base).
- The velocity v2 can be related to v1 using the continuity equation.
Step 4: Apply the Continuity Equation
The continuity equation states:
A1v1=A2v2
Where:
- A1=A (cross-sectional area of the tank).
- A2=A3 (cross-sectional area of the orifice).
From this, we can express v2:
v2=AA/3v1=3v1
Step 5: Substitute into Bernoulli’s Equation
Substituting the values into Bernoulli’s equation:
P0+ρgH+12ρv21=P0+0+12ρ(3v1)2
Step 6: Simplify the Equation
Cancelling P0 from both sides and simplifying gives:
ρgH+12ρv21=12ρ(9v21)
ρgH=12ρ(9v21−v21)
ρgH=12ρ(8v21)
Cancelling ρ from both sides:
gH=4v21
Step 7: Solve for v1
Rearranging gives:
v21=gH4
Taking the square root:
v1=√gH4=12√gH
Final Answer
The initial velocity of the top surface of the liquid is:
v1=12√gH
We have a tank with a cross-sectional area A filled with liquid to a height H. At the bottom of the tank, there is an orifice with a cross-sectional area of A3.
We will apply Bernoulli’s equation between two points:
1. At the top surface of the liquid (Point 1).
2. At the orifice (Point 2).
P1+ρgH+12ρv21=P2+ρgh2+12ρv22
Where:
- P1 and P2 are the pressures at points 1 and 2 respectively.
- ρ is the density of the liquid.
- g is the acceleration due to gravity.
- H is the height of the liquid column.
- v1 is the velocity of the liquid at the top surface.
- v2 is the velocity of the liquid exiting the orifice.
At the top surface of the liquid (Point 1):
- The pressure P1=P0 (atmospheric pressure).
- The height H is the height of the liquid column.
- The velocity v1 is what we want to find.
- The pressure P2=P0 (atmospheric pressure).
- The height h2=0 (since it is at the base).
- The velocity v2 can be related to v1 using the continuity equation.
The continuity equation states:
A1v1=A2v2
Where:
- A1=A (cross-sectional area of the tank).
- A2=A3 (cross-sectional area of the orifice).
v2=AA/3v1=3v1
Substituting the values into Bernoulli’s equation:
P0+ρgH+12ρv21=P0+0+12ρ(3v1)2
Cancelling P0 from both sides and simplifying gives:
ρgH+12ρv21=12ρ(9v21)
ρgH=12ρ(9v21−v21)
ρgH=12ρ(8v21)
Cancelling ρ from both sides:
gH=4v21
Rearranging gives:
v21=gH4
Taking the square root:
v1=√gH4=12√gH
The initial velocity of the top surface of the liquid is:
v1=12√gH
Topper's Solved these Questions
Similar Questions
A 10cm side cube weighing 5N is immersed in a liquid of relative density 0.8 contained in a rectangular tank of cross section a area 15cm times15cm .If the tank contained liquid to a height of 8cm before the immersion the level of the liquid surface is :
View SolutionA cylindrical massless container of cross sectional area A have a fluid filled up to height h and have a small orifice of area a in wall near its bottom. Find minimum coefficient between container and ground so that container does not move
View Solution
Knowledge Check
A tank, which is open at the top, contains a liquid up to a height H. A small hole is made in the side of the tank at a distance y below the liquid surface. The liquid emerging from the hole lands at a distance x from the tank :
 AIF y is increased from zero to H, x will first increase and then decreaseBx is maximum for y=H/2CThe maximum value of x is HDThe maximum value of x will depend on the density of the liquid
AIF y is increased from zero to H, x will first increase and then decreaseBx is maximum for y=H/2CThe maximum value of x is HDThe maximum value of x will depend on the density of the liquidA tank which is open at the top, contains a liquid up to a height H. A small hole is made in the side of the tank at a distance y below the liquid surface. The liquid emerging from the hole lands at a distance x from the tank. Choose incorrect option.
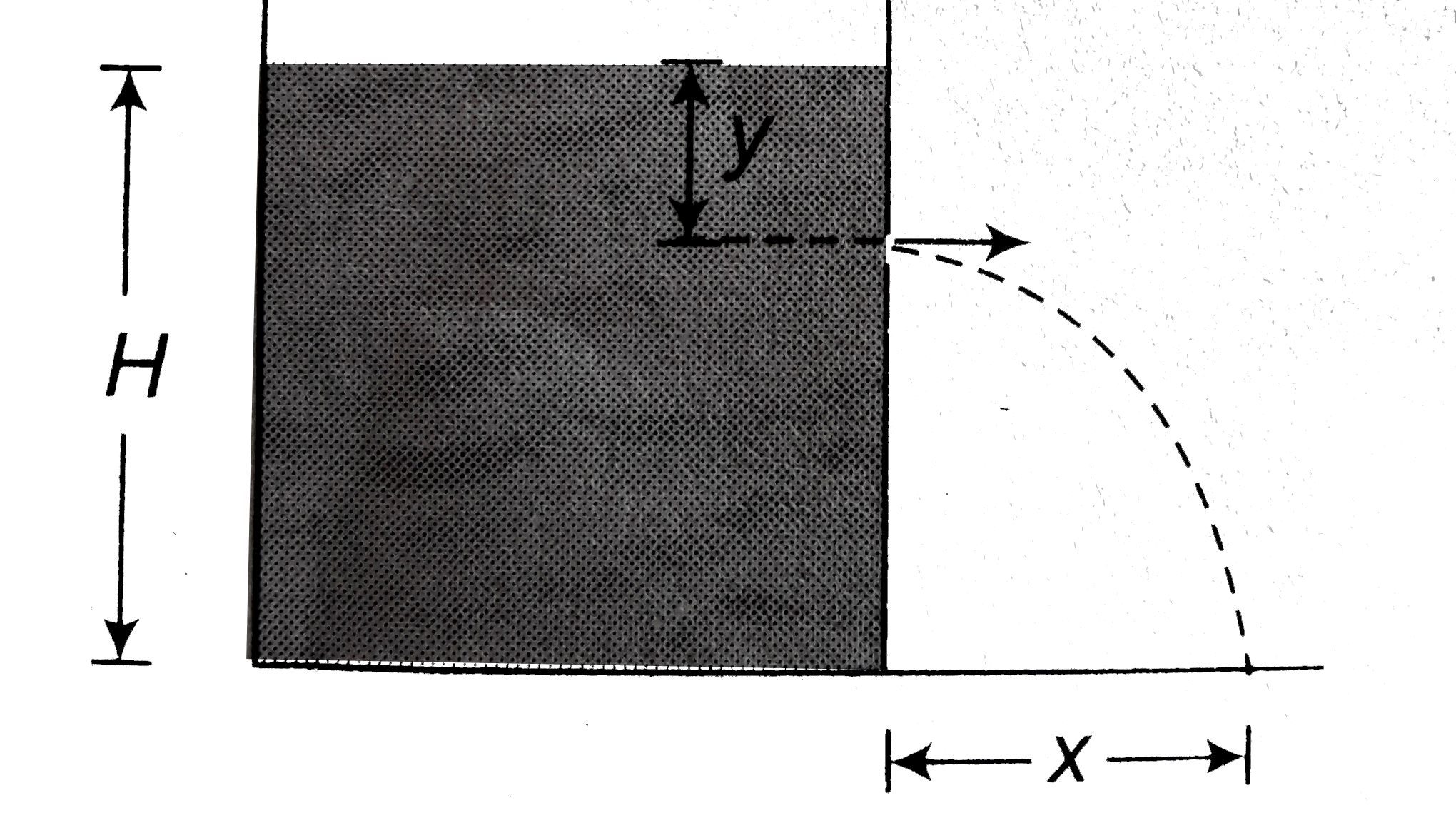 .AIf y is increased from zero to H, x will first increase and then decreaseBx is maximum for y=H/2CThe maximum value of x is HDThe maximum value of x will depend on the density of the liquid.
.AIf y is increased from zero to H, x will first increase and then decreaseBx is maximum for y=H/2CThe maximum value of x is HDThe maximum value of x will depend on the density of the liquid.A vessel of area of cross-section A has liquid to a height H . There is a hole at the bottom of vessel having area of cross-section a . The time taken to decrease the level from H1 to H2 will sec
AAa√2g[√H1−√H2]B√2ghC√2gh(H1−H2)DAa√g2[√H1−√H2
A tank of square cross-section of each side is filled with a liquid of height h . Find the thrust experienced by the vertical surfaces and bottom surface of the tank.
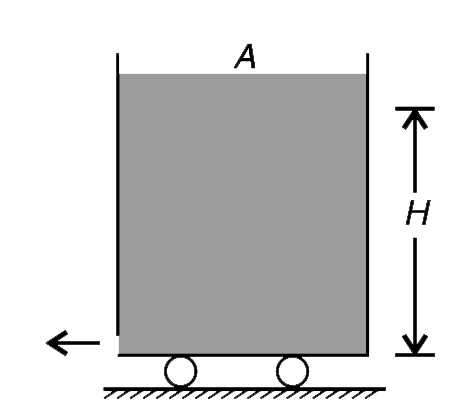
View SolutionAn open tank of cross sectional area A contains water up to height H. It is kept on a smooth horizontal surface. A small orifice of area A0 is punched at the bottom of the wall of the tank. Water begins to drainout. Mass of the empty tank may be neglected.
(i) Prove that the tank will move with a constant acceleration till it is emptied. Find this acceleration.
(iii) Find the find speed acquired by the tank when it is completely empty.
View SolutionA cylindrical vessel of uniform cross-section contains liquid upto the height' H’ . At a depth H=H2 below the free surface of the liquid there is an orifice. Using Bernoulli's theorem, find the velocity of efflux of liquid.
View SolutionAssertion : The pressure at the bottom of two tanks of different area of cross sections are equal if they contain same liquid to same height .
Reason : Pressure of a liquid is hdg and is independent of shape and width of the container .
View SolutionA body of uniform cross-sectional area floats in a liquid of density thrice its value. The portion of exposed height will be :
View Solution
