The vector -→Ais:
greater than →A in magnitude
less than →A in magnitude
in the same direction as →A
in the direction opposite to →A
The correct Answer is:D
Step by step video, text & image solution for The vector -vec(A) is : by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- UNIT DIMENSION, VECTOR & BASIC MATHSBOOK - BANSALCHAPTER - UNIT DIMENSION, VECTOR & BASIC MATHSEXERCISE - Solved Example
17 Videos
- UNIT DIMENSION, VECTOR & BASIC MATHSBOOK - BANSALCHAPTER - UNIT DIMENSION, VECTOR & BASIC MATHSEXERCISE - PRACTICE EXERCISE
4 Videos
- KINETIC THEORY OF GASESBOOK - BANSALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Section-B
13 Videos
Similar Questions
Knowledge Check
The vector →a lies in the plane of vectors →band→c. Which one of the following is correct ?
A→a.(→b×→c)=0B→a.(→b×→c)=1C→a.(→b×→c)=−1D→a.(→b×→c)=3The magnitude of the x-component of vector →A is 3 and the magnitude of vector →A is 5. What is the magnitude of the y-component of vector →A ?
A3B4C5D8If the vector →a lies in the planar of vectors →band→c, then which one of the following is correct?
A→a.(→b×→c)=0B→a.(→b×→c)=1C→a(→b×→c)=−1D→a(→b×→a)=3
A vector →A is along the positive x-axis and its vector product with another vector →B is zero, then vector →B could be
View SolutionWhich one of the following is correct ? If the vector →c is normal to the vectors →aand→b, then →c, is :
View SolutionThe resultant of two vectors →A and →B is perpendicular to the vector →A and its magnitudes is equal to half of the magnitudes of vector →B (figure). The angle between →A and →B is

View SolutionKeeping one vector constant, if direction of other to be added in the first vector is changed continuously, tip of the resultant vector describes a circles, In the following figure vector →a is kept constant. When vector →b addede to →a changes its direction, the tip of the resultant vector →r=→a+→b describes circles of radius b with its centre at the tip of vector →a. Maximum angle between vector →a and the resultant →r=→a+→b is
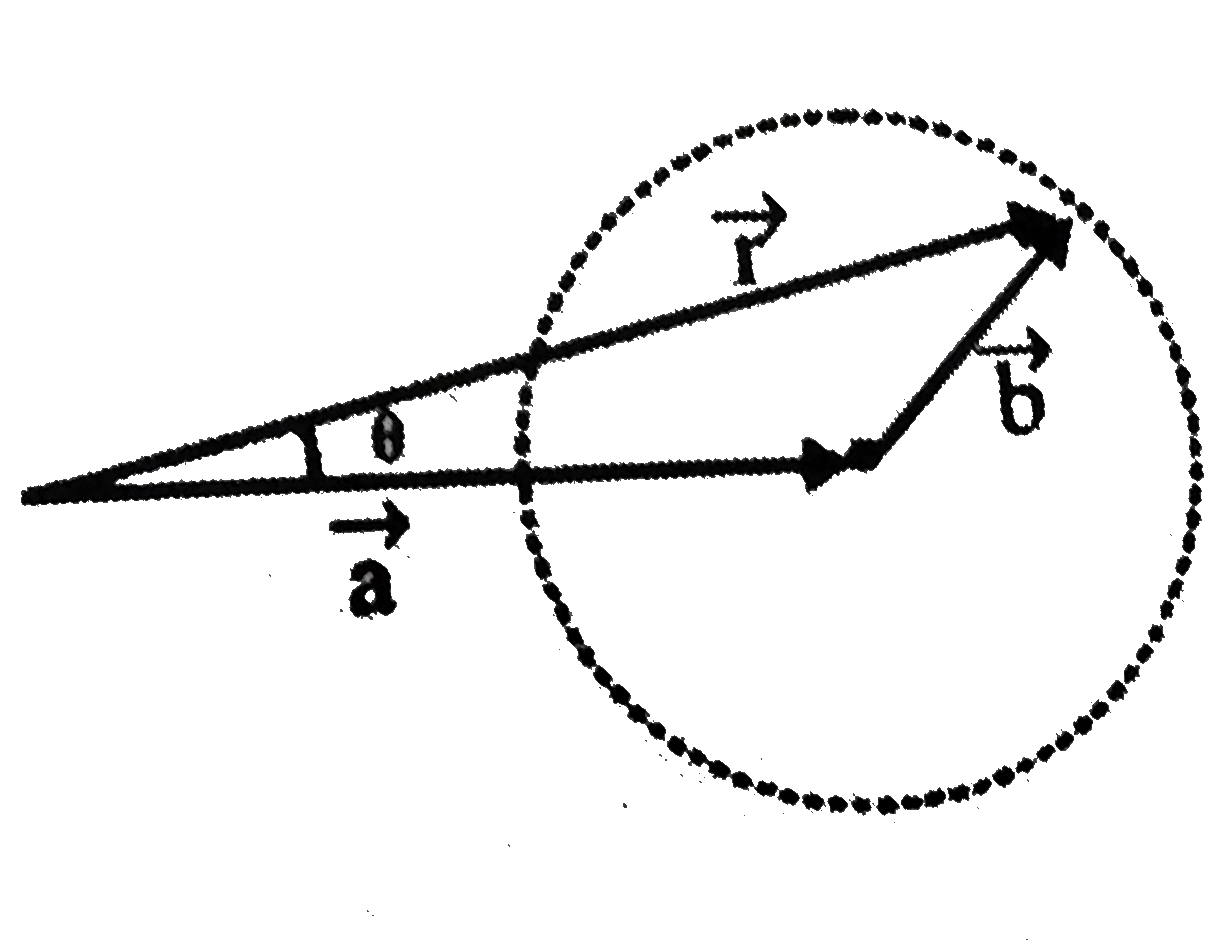
View SolutionThe vectors vec(a) = - 4hat(i) + 3hat(k), vec(b)
View Solution