Calculate Centre of mass of a non-uniform rod with linear mass density γ

λ(mass/length)=kx2
Video Solution
Text Solution
For a small element dx, the linear mass density can be considered constant:
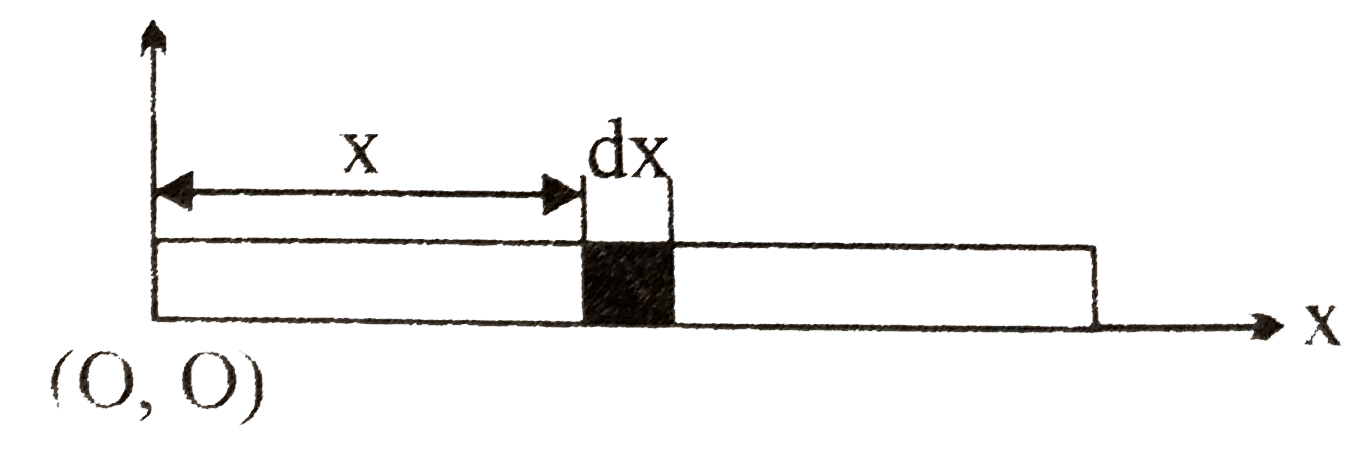
MassLength=lanbda=kx2 ∴ mass of this small element dm=λdx=kx2dx
M=k∫L0x2dx=KL33
xcm=∫x dm∫dm=K∫L0x3dxKL33=3L4
|
Topper's Solved these Questions
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - Solved Examples
15 Videos
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - Practice Exercise
20 Videos
- GRAVITATIONBOOK - BANSALCHAPTER - GRAVITATIONEXERCISE - EXERCISE -4 Section - B
6 Videos
Similar Questions
Knowledge Check
The position of the centre of mass of a cube of uniform mass density will be at
Athe centre of one faceBthe centre of the interaction of diagonals of one face.Cthe geometric centre of the cubeDthe edge of a cubeThe centre of mass of a non uniform rod of length L whoose mass per unit length varies asp=kx2/L, (where k is a constant and x is the distance measured form one end) is at the following distances from the same end
A3L/4BL/4C2L/3DL/3The centre of mass of a non uniform rod of length L, whose mass per unit length varies as ρ=k.x2L where k is a constant and x is the distance of any point from one end is (from the same end)
A(34)LB(14)LC(16)LD(23)L
The centre of mass of a non-uniform rod of length L whose mass per unit length λ is proportional to x2, where x is distance from one end
View SolutionThe centre of mass of a non uniform rod of length L whose mass per unit length λ=Kx2/L where K is a constant and x is the distance from one end is
(A) 3L4
(B) L8
(C) KL
(D) 3KL
View SolutionThe centre of a mass of any object having uniform density is at its centroid
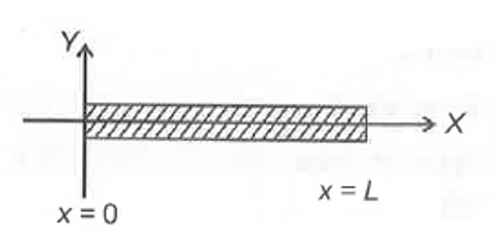
View SolutionFind the position of centre of mass of the uniform lamina shown in figure. If the mass density of the lamina is σ.
View SolutionThe centre of mass of a, non uniform rod of length L whose mass per unit length p varies as p =kx2Lwhere k: is a constant and x is the distance of any point from one end, is (from the same end):
View Solution