A small sphere falls from rest in a viscous liquid. Due to frication, heat is produced. Find the relation between the rate of production of heat and the radius of the sphere at terminal velocity.
The correct Answer is:dQdt∝r5
Terminal velocity vT=2r2g9η(ρs−ρL)
and viscous force F=6πrvT
Viscous force is the dissipative force. Hence.
dQdt=FvT=(6πηrvT)(vT)=6πηrv2T
=6πηr{29r2gη(ρs−ρL)}2=8πg227η(ρs−ρL)2r5=dQdt∝r5
Topper's Solved these Questions
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Exercise- 3 PART - IV
9 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Exercise- 2 PART - III
11 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 92 illustration
2 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise
43 Videos
Similar Questions
A small sphere falls from rest in a viscous liquid. Due to friction, heat is prodced. Find the relation between the rate of production of heat and the radius of the sphere at terminal velocity.
View SolutionA small sphere falls from rest in a viscous liquid. Due to friction, heat is produced. Find the relation between the rate of produced heat and the radius of the sphere at terminal velocity.
View Solution
Knowledge Check
A small sphere falls from rest in a viscous liquid. Due to frication, heat is produced. Find the relation between the rate of production of heat and the radius of the sphere at terminal velocity.
Ar3Br2Cr3Dr4For a small temperature difference between the body and the surroundings the relation between the rate of loss heat R and the temperature of the body is depicted by
A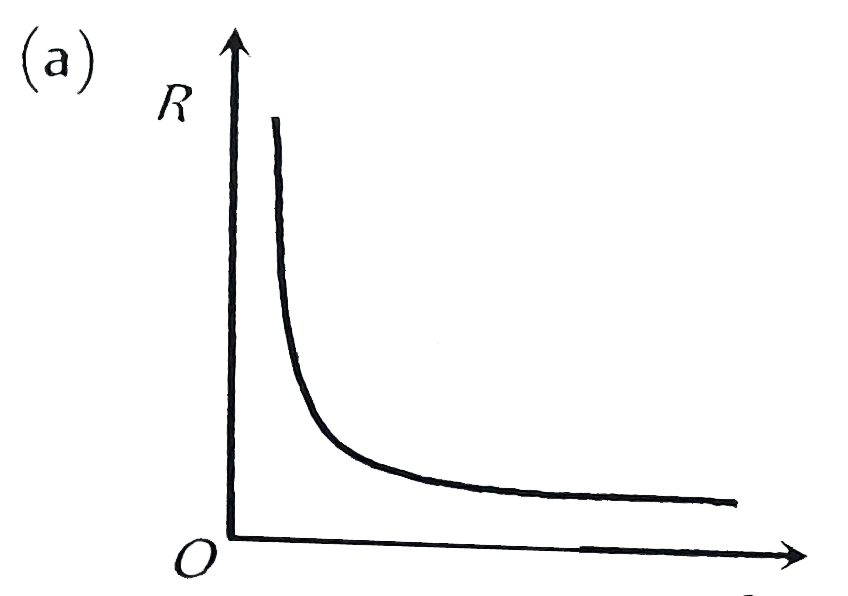 B
B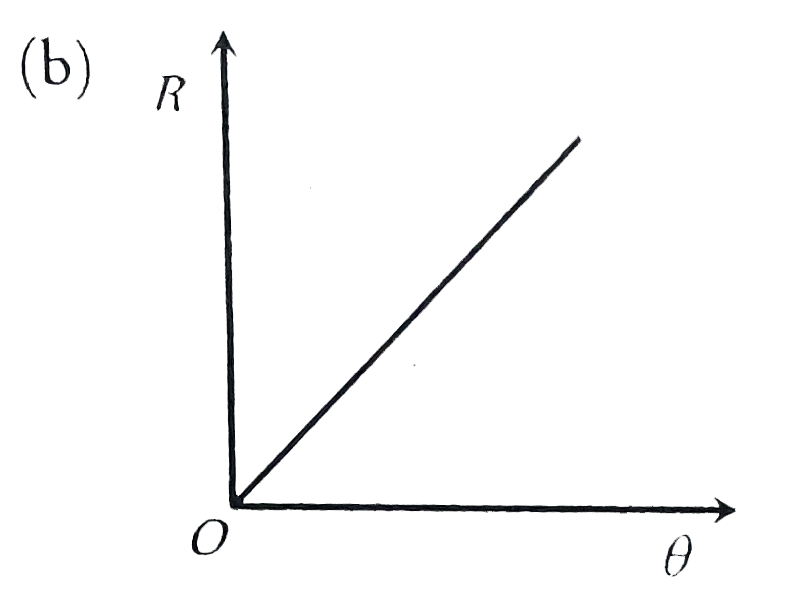 C
C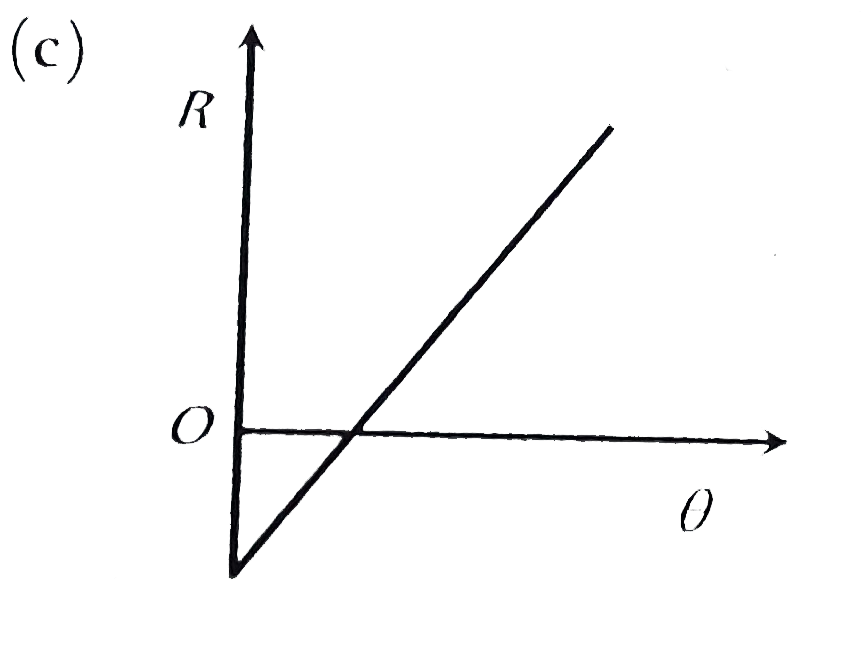 D
D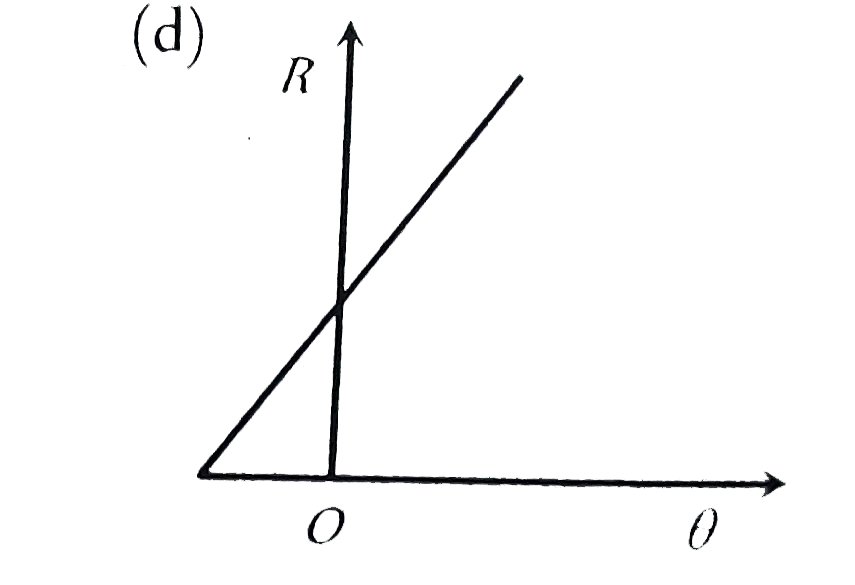
When a sphere falling in a viscous fluid attains a terminal velocity, then
Athe net force acting on the sphere is zeroBthe drag force balances the buoyant forceCthe drag force balances the weight of the sphereDthe buoyant force balances the weight and drag force
A small spherical body of radius r is falling under gravity in a viscous medium. Due to friction the meduim gets heated. How does the rate of heating depends on radius of body when it attains terminal velocity?
View SolutionHeat is produced due to viscous forces when a small sphere of radius r falls from rest in a viscous liquid. When the sphere has reached its terminal velocity, the rate of heat production is proportional to rn, where n = _____.
View SolutionA small spherical ball falling under gravity in a viscous medium heat the medium due to viscous drage force. The rate of heating is proportional to rn. (r= radius of the sphere find )n.
View SolutionFor a small temperature difference between the body and the surroundings, the relation between the rate of heat loss R and the temperature of the body is depicted by
View SolutionA solid metallic sphere of radius r is allowed to fall freely through air. If the frictional resistance due to air is proportional to the cross-sectional area and to the square of the velocity, then the terminal velocity of the sphere is proportional to which of the following ?
View Solution