shown in the figure is a small loop that is kept co-axially with the bigger loop. If the slider moves from A to B, then

Current flow in both the loops will be in opposite senses.
Clockwise current in loop 1 and anti-clockwise current in loop 2 flow
No current flows in loop 2
Clockwise current flows in loop 2
When the slider moves towards B, the resistance of the circuit (bigger loop) decrease. Therefore, the current in the bigger loop increases. The increasing current results in increasing flux (ϕαi) linked in the smaller coil. Consequently, induced emf will be generated along the smaller loop creating an induced current so as to oppose the increase in flux. Therefore the current flows anticlockwise, in the inner loop.
Topper's Solved these Questions
Similar Questions
A rectangular current carrying loop PQRS is kept in a uniform magnetic field as shown in the figure.
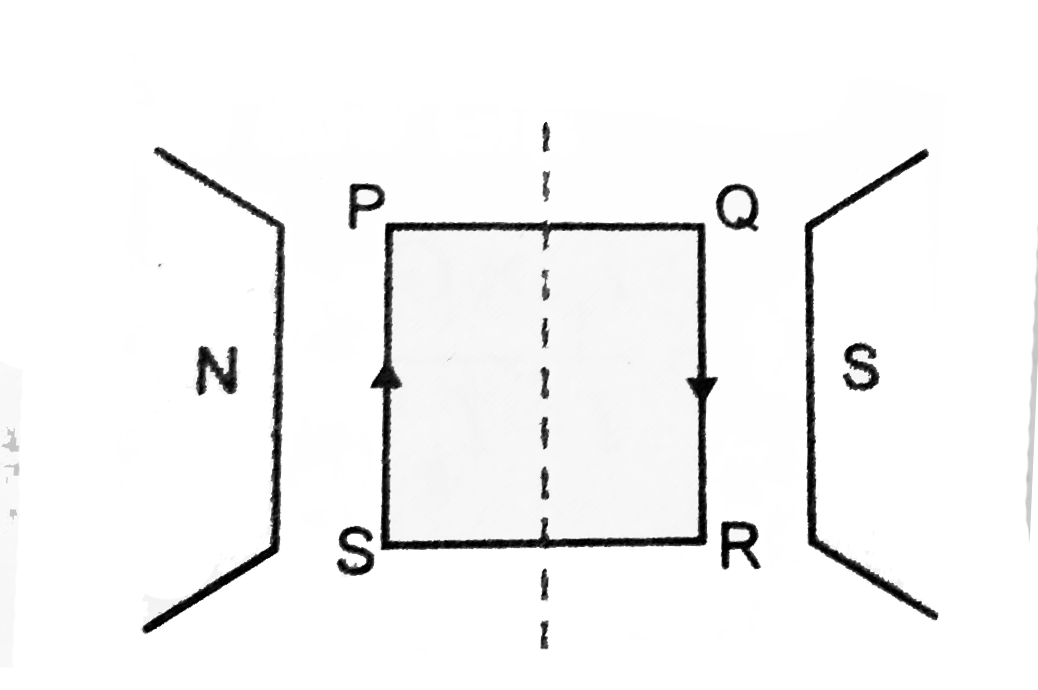
(a) What is the direction of magnetic moment of the current loop?
(b) When is the torque acting on the loop
(i) maximum, (ii) zero?
View SolutionA constant current I flows through a long straight wire as shown in figure. A square loop starts moving towards righ with as constant speed v.

a Find induced emf produced i the loop as a function x
b. If total resistance of the loop is R, then find induced current in the loop,
View Solution
Knowledge Check
A circular loop of radius 0.3 cm lies parallel to amuch bigger circular loop of radius 20 cm. The centre of the small loop is on the axis of the bigger loop. The distance between their centres is 15 cm. If a current of 2.0 A flows through the smaller loop, then the flux linked with bigger loop is
A9.1×10−11weberB6×10−11weberC3.3×10−11weberD6.6×10−11weberA circular loop of radius 0.3 cm lies parallel to much bigger circular of radius 20 cm. The centre of the small loop is on the axis of the bigger loop. The distance between their centres is 15 cm. If a current of 2.0 A flows, through the smaller loop, then the flux linked with the bigger loop is:
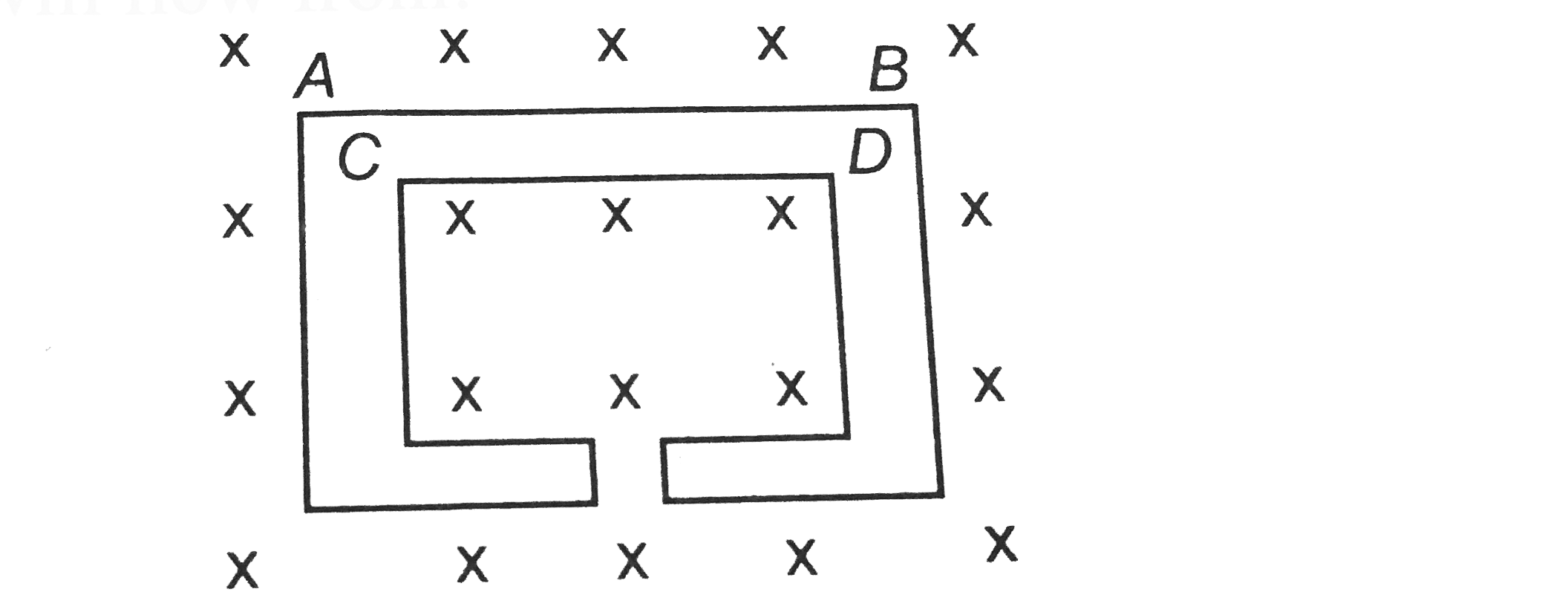
A3.3×10−11 weberB6×10−11 weberC6.6×10−9 weberD9.1×10−11 weberA wire is bent to form the double loop shown in figure. There is a uniform magnetic field directed into the plane of the loop. If the magnitude of this field is decreasing, current will flow from:
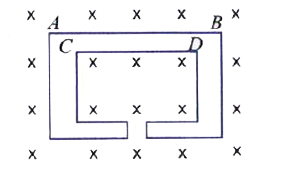 AA to B and C to DBB to A and D to CCA to B and D to CDB to A and C to D
AA to B and C to DBB to A and D to CCA to B and D to CDB to A and C to D
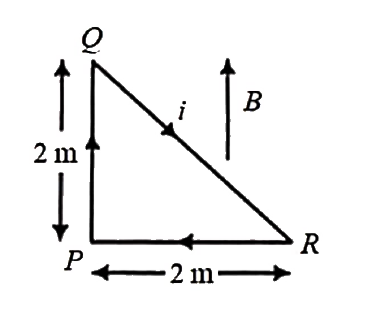
A loop PQR carries a current i=2A as shown in the figure. A uniform magnetic field B = 2T exists in space parallel to the plane of the loop . The magnetic torque on the loop is
View SolutionA wire is bent to form the double loop shown in figure. There is a uniform magnetic field directed into the plane of the loop. If the magnitude of this field is decreasing current will flow from:
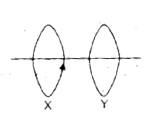
View SolutionThe two loops shown in the figure have their planes parallel to each other . A clockwise current flows in the loop X as viewed from X towards Y. The two coils will repel each other if the current in the loop X is :
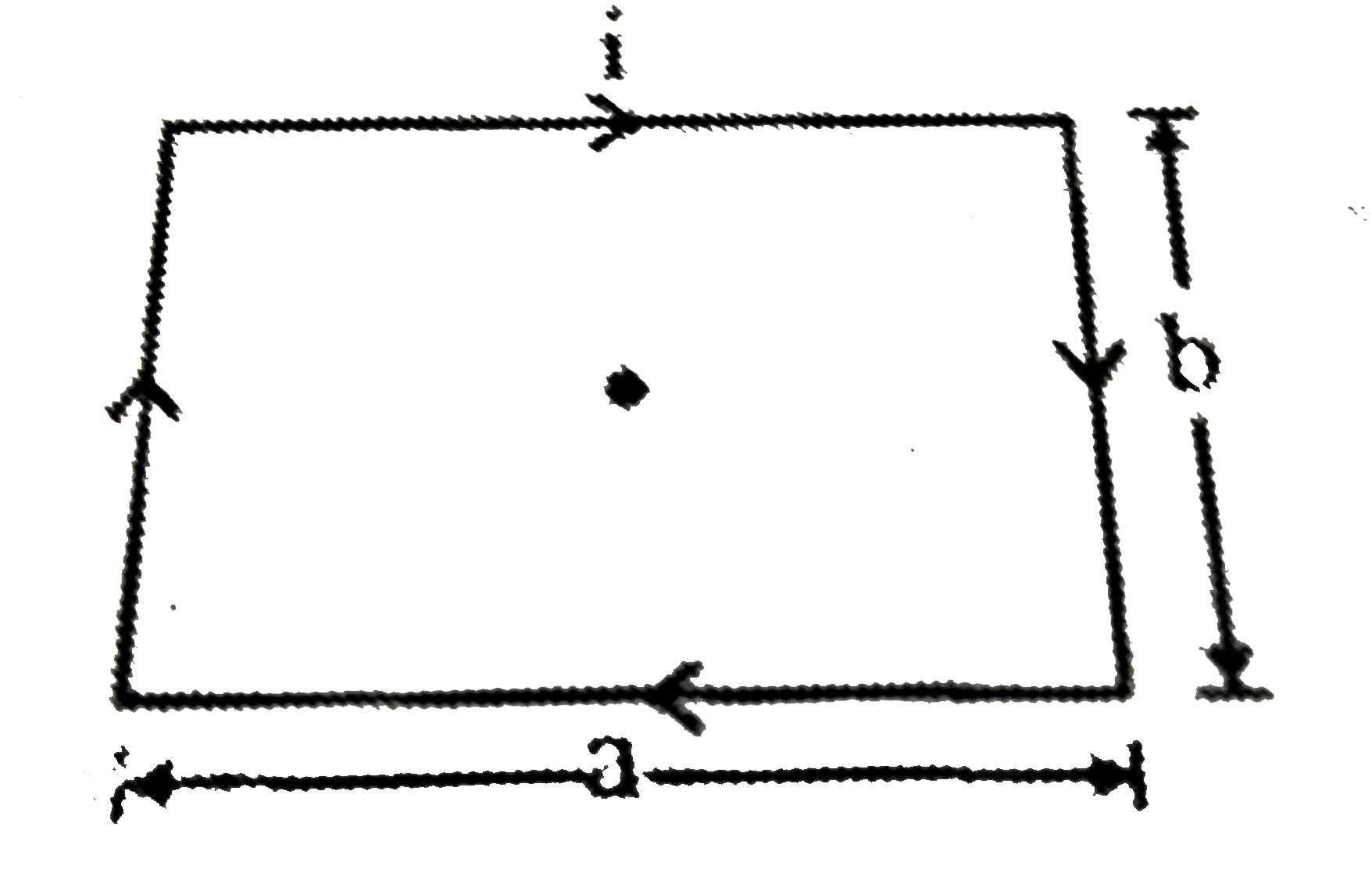
View SolutionShown in the figure is a rectangular loop of conductor carrying a current i. The length and breath of the loop are respectively a and b. The magnetic field at the centre of loop is -
View SolutionA bar magnet with its north (N) and south (S) poles as shown in the following figure is initially moving to the left, along the axis of, and away from a circular conducting loop. A current l is induced in the loop, with a being the acceleration of the magnet due to this current. As seen from the magnet looking in the direction of the loop,

View Solution