Initially two particles A and B are present at (0,0) and (d,0) respectively They start moving with speed VA=Vˆi+Vˆj and VB=−Vˆj If R is magnitude of relative separation between them and T0 be the time when separation between them is minimum, then
T0=d5V
Rmin=2d√5
Graph of R versus time is straight line
Graph of R versus time is circle.
The correct Answer is:A, B
To solve the problem, we will follow these steps:
Step 1: Define the initial positions and velocities
- Particle A is at position (0,0) and moves with velocity VA=V^i+V^j.
- Particle B is at position (d,0) and moves with velocity VB=−V^j.
Step 2: Determine the relative velocity
The relative velocity of A with respect to B is given by:
VAB=VA−VB
Substituting the values:
VAB=(V^i+V^j)−(0^i−V^j)=V^i+2V^j
Step 3: Calculate the magnitude of the relative velocity
The magnitude of the relative velocity VAB is:
|VAB|=√(V)2+(2V)2=√V2+4V2=√5V2=V√5
Step 4: Find the time T0 when the separation is minimum
To find the time when the separation is minimum, we need to determine the perpendicular distance from particle B to the line of motion of particle A. The minimum distance occurs when the line connecting A and B is perpendicular to the direction of VAB.
The distance D between the initial positions of A and B is d. The time T0 to reach the minimum separation is given by:
T0=Dcosα|VAB|
Where cosα=1√5 (from the triangle formed by the components of VAB):
T0=d⋅1√5V√5=d5V
Step 5: Calculate the minimum separation Rmin
The minimum separation occurs when the perpendicular distance from particle B to the line of motion of A is given by:
Rmin=Dsinα
Where sinα=2√5 (using the triangle formed by the components of VAB):
Rmin=d⋅2√5=2d√5
Final Results
- The time T0 when the separation is minimum is:
T0=d5V
- The minimum separation Rmin is:
Rmin=2d√5
- Particle A is at position (0,0) and moves with velocity VA=V^i+V^j.
- Particle B is at position (d,0) and moves with velocity VB=−V^j.
The relative velocity of A with respect to B is given by:
VAB=VA−VB
Substituting the values:
VAB=(V^i+V^j)−(0^i−V^j)=V^i+2V^j
The magnitude of the relative velocity VAB is:
|VAB|=√(V)2+(2V)2=√V2+4V2=√5V2=V√5
To find the time when the separation is minimum, we need to determine the perpendicular distance from particle B to the line of motion of particle A. The minimum distance occurs when the line connecting A and B is perpendicular to the direction of VAB.
T0=Dcosα|VAB|
Where cosα=1√5 (from the triangle formed by the components of VAB):
T0=d⋅1√5V√5=d5V
The minimum separation occurs when the perpendicular distance from particle B to the line of motion of A is given by:
Rmin=Dsinα
Where sinα=2√5 (using the triangle formed by the components of VAB):
Rmin=d⋅2√5=2d√5
- The time T0 when the separation is minimum is:
T0=d5V
- The minimum separation Rmin is:
Rmin=2d√5
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Subjective
107 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic
70 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
In a given system of unit standard measurement of mass is 100 gm standard measurement of length is 200 cm and standard measurement of time is 5 sec 10 J energy in the given system of unit has value N, then value of N is
View SolutionThe manifestation of band structure in solids is due to
View SolutionStatement-1: The numerical value (n)of a physical quantity is inversely proportional to size of unit (u) being used to measure it.
Statement-2:The magnitude of physical quantity does not change by changing the units.
View SolutionA cylinder of height 20m is completely filled with water. The velocity of effux of water (∈ms−1) through a small hole on the side wall of the cylinder near its bottom is
View SolutionHow does the width of the depletion layer of a p-n junction diode change with decrease in reverse bias?
View SolutionShip A moving with velocity vec(V_(1)) = 30 hat(i) + 50 hat(j) from position (0, 0) and ship B moving with velocity vec(V_(2)) = - 10 hat(i) from position (80, 150). The time for minimum separation is : (A) 2.6 (B) 2.2 (C) 2.4 (D) None
View SolutionTwo particles are located on a horizontal plane at a distance 60m. At t=0 both the particles are simultaneously projected at angle 45∘ with velocities 2ms−1and14ms−1, respectively. Find
(a) Minimum separation between them during motion.
(b) At what time is the separation between them minimum ?
 .
.
View SolutionParticles A and B are moving with constant velocities along x and y axis respectively, the graph of separation between them with time is
View Solution(a) Two protons are placed at separation d on a smooth surface. Find the speed of each proton when separation between them is doubled.
(b) An α-particle and a proton are placed at a separation d on a smooth surface.Find the speed of each when separation between them becomes infinite. (m: mass of proton , e: charge of proton.)
View SolutionTwo particles A and B locat at (0,0) and (4,4) respectively start moving simu lataneou ly with velocities vA=−4ˆi and VB=−4ˆj:
View SolutionTwo objects A and B are moving in opposite directions with velocities v_(A) and v_(B) respectively, the magnitude of relative velocity of A w.r.t. B is
View Solutionकिसी कण के सापेक्ष वेग क्या अभिप्राय है ? दो कण A तथा B एक सीधी सड़क पर एक ही दिशा में क्रमश :vA एवं vB वेग में गतिशील हैं। दिखाइए कि कण A का कण B के सापेक्ष वेग vAB=vA−vB.
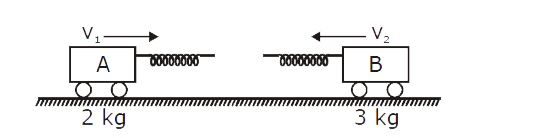
View SolutionTwo carts (A & B) having spring bumpers collides as shown in figure. Initially v1≠0 & v2=0. When the separation between the carts is minimum.
View SolutionFind out speed of particles when separation between them is r.
View SolutionIf two objects moving with a velocities of V_(A)andV_(B) at an angle of theta between them, the relative velocity is
View Solution