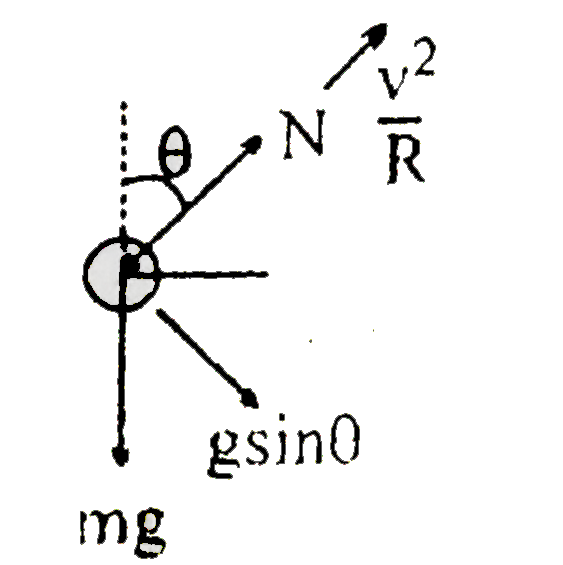
A particle of mass m is going along surface of smooth hemisphere of radius R in verticle plane. At the moment shown its speed is v. Choose correct option(s).

mg−Ncosθ=m(gsin2θ−v2Rcosθ)
N−mgcosθ=mv2R
mg−Nsinθ=mv2R
Nsinθ=m(gsinθcosθ−v2Rsinθ)
The correct Answer is:A, B
mg−Ncosθ=mgsinθ−mv2Rcosθ
Nsinθ=mv2Rsinθ+mgcosθsinθ
N−mgcosθ=mv2R
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Subjective
107 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic
70 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
A heavy particle of mass m is in motion on a smooth surface of hemisphere of radius R and there is no friction. At the initial instant the particle is at the topmost point A and has an initial velocity v0 At what point will the particle leave the surface of the hemisphere ? Also determine the value of v0 for which the particle will leave the sphere at the initial instant.
View SolutionA particle of mass m is released from the top of a smooth hemisphere of radius R with the horizontal speed μ. Calculate the angle with verticle where it loses contact with the hemisphere.
View Solution
Knowledge Check
A particle of mass m initially at rest starts moving from point A on the surface of a fixed smooth hemisphere of radius r as shown. The particle looses its contact with hemisphere at point B.C is centre of the hemisphere. The equation relating θ and θ' is
 .A3sinθ=2cosθ'B2sinθ=3cosθ'C3sinθ'=2cosθD2sinθ=3cosθ'
.A3sinθ=2cosθ'B2sinθ=3cosθ'C3sinθ'=2cosθD2sinθ=3cosθ'The sphere A of mass m1 moves with velocity V on a frictionless horizontal surface and strikes with sphere B of mass m2 at rest. The sphere A comes back with speed V/10. Choose the correct option.
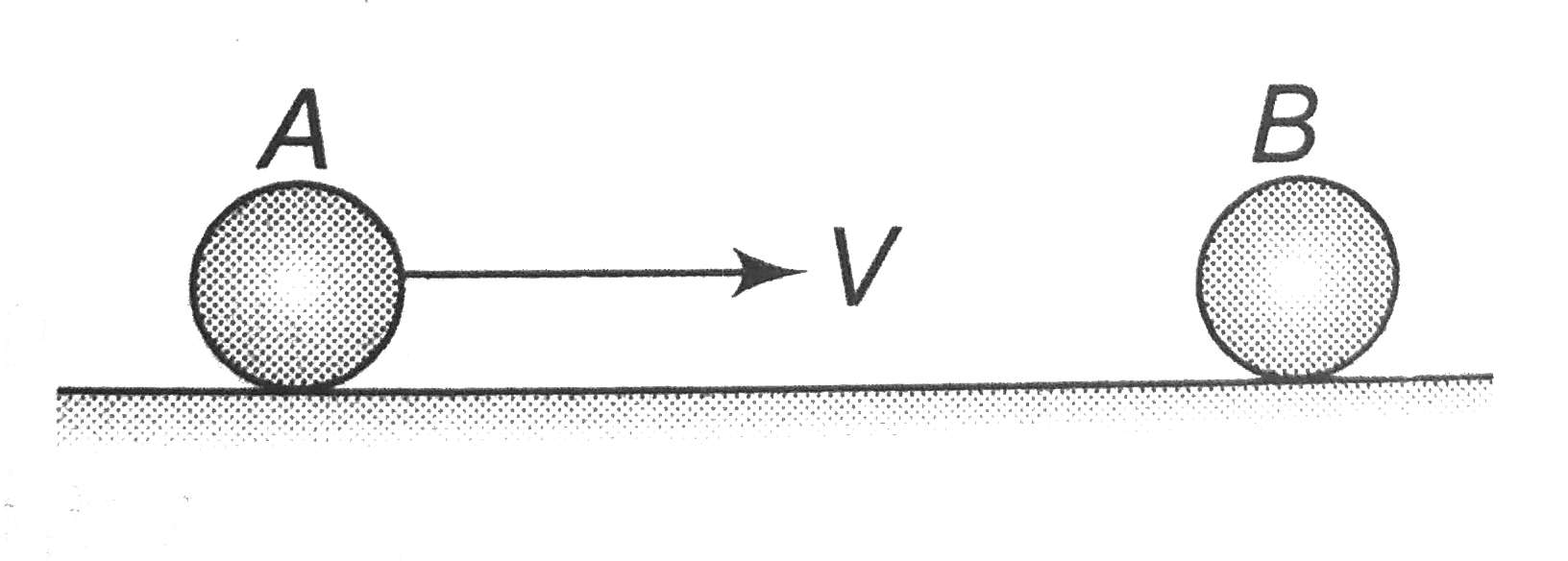 Am1>m2Bm1=m2Cm1<m2Dnone of these
Am1>m2Bm1=m2Cm1<m2Dnone of theseA particle of mass m is being circulated on a vertical circle of radius r. If the speed of particle at the highest point be v, then
Amg=mv2rBmg>mv2rCmg≤mv2rDmg≥mv2r
A particle of mass 'm' moves with a constant speed along a circular path of radius r under the action of a force F .Its speed is given by
View SolutionA particle of mass m is released from a height H on a smooth curved surface which ends into a vertical loop of radius R, as shown. Choose the correct alernative(s) if H=2R.
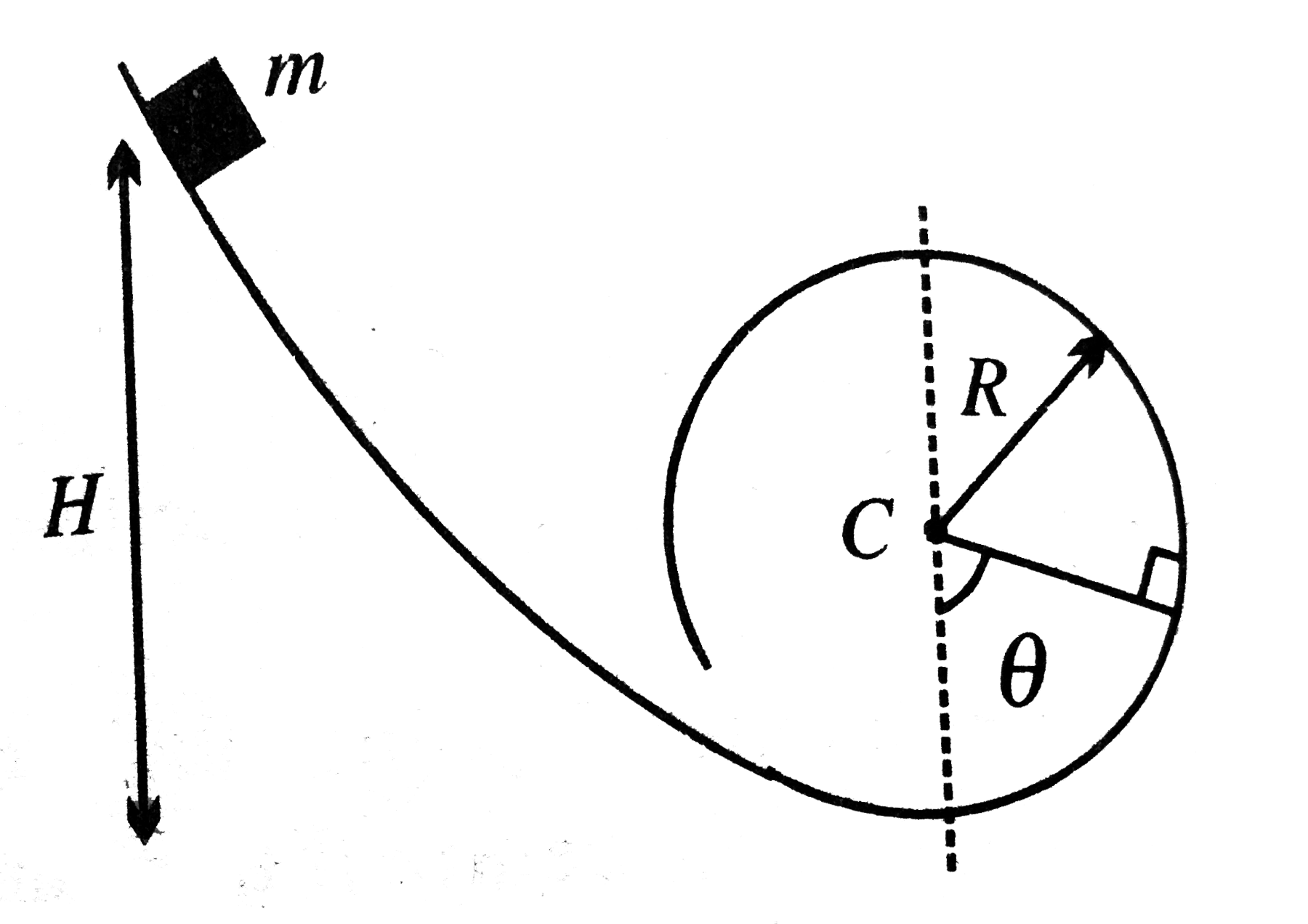
View SolutionA particle of mass M moves with constant speed along a circular path of radius r under the action of a force F. Its speed is
View SolutionA particle of mass 'm' is moving along a circle of radius 'r'. At some instant, its speed is 'v' and it is gaining speed at a uniform rate'a', then, at the given instant, acceleration of the mparticle is :
View SolutionA body of mass Mkg is on the top point of a smooth hemisphere of radius 5m . It is released to slide down the surface of hemisphere it leaves the surface when its velocity is 5ms−1 At this instant the angle made by the radius vector of the body with the vertical is ( Take g=10m/s2)
View Solution
